Which describes the system of equations above?
Understand the Problem
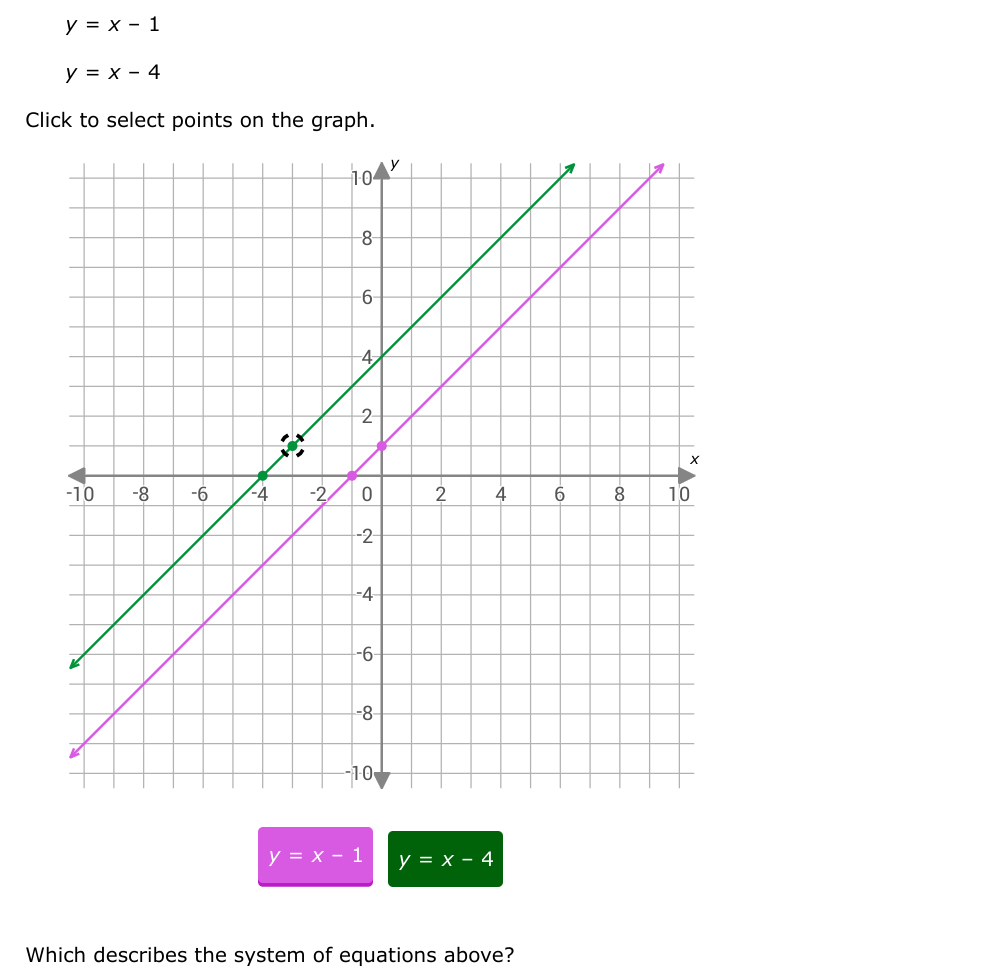
The question is asking to describe the relationship between the two linear equations represented in the graph. The focus is on understanding the system of equations and their geometric representation.
Answer
The equations represent parallel lines: $y = x - 1$ and $y = x - 4$.
Answer for screen readers
The system of equations consists of two parallel lines: $y = x - 1$ and $y = x - 4$.
Steps to Solve
- Identify the equations and slopes
The given equations are: $$ y = x - 1 $$ $$ y = x - 4 $$
Both equations are in slope-intercept form, $y = mx + b$, where $m$ is the slope.
- For $y = x - 1$, the slope ($m_1$) is 1.
- For $y = x - 4$, the slope ($m_2$) is also 1.
- Determine the y-intercepts
Next, we find the y-intercepts ($b$) of each equation:
- For $y = x - 1$, the y-intercept is $-1$ (where the line crosses the y-axis).
- For $y = x - 4$, the y-intercept is $-4$.
- Analyze the relationship between the lines
Since both lines have the same slope (1) but different y-intercepts, this indicates that the lines are parallel. They will never intersect because they rise at the same rate but start from different heights on the y-axis.
The system of equations consists of two parallel lines: $y = x - 1$ and $y = x - 4$.
More Information
Parallel lines have the same slope but different y-intercepts, which means they never meet. This is an essential concept in understanding linear equations and their graphical representations.
Tips
- Confusing parallel lines with intersecting lines: Remember that parallel lines have identical slopes and will never intersect, while intersecting lines have different slopes.
- Miscalculating the y-intercepts: Always verify the y-intercept by examining the constant in the equations.
AI-generated content may contain errors. Please verify critical information