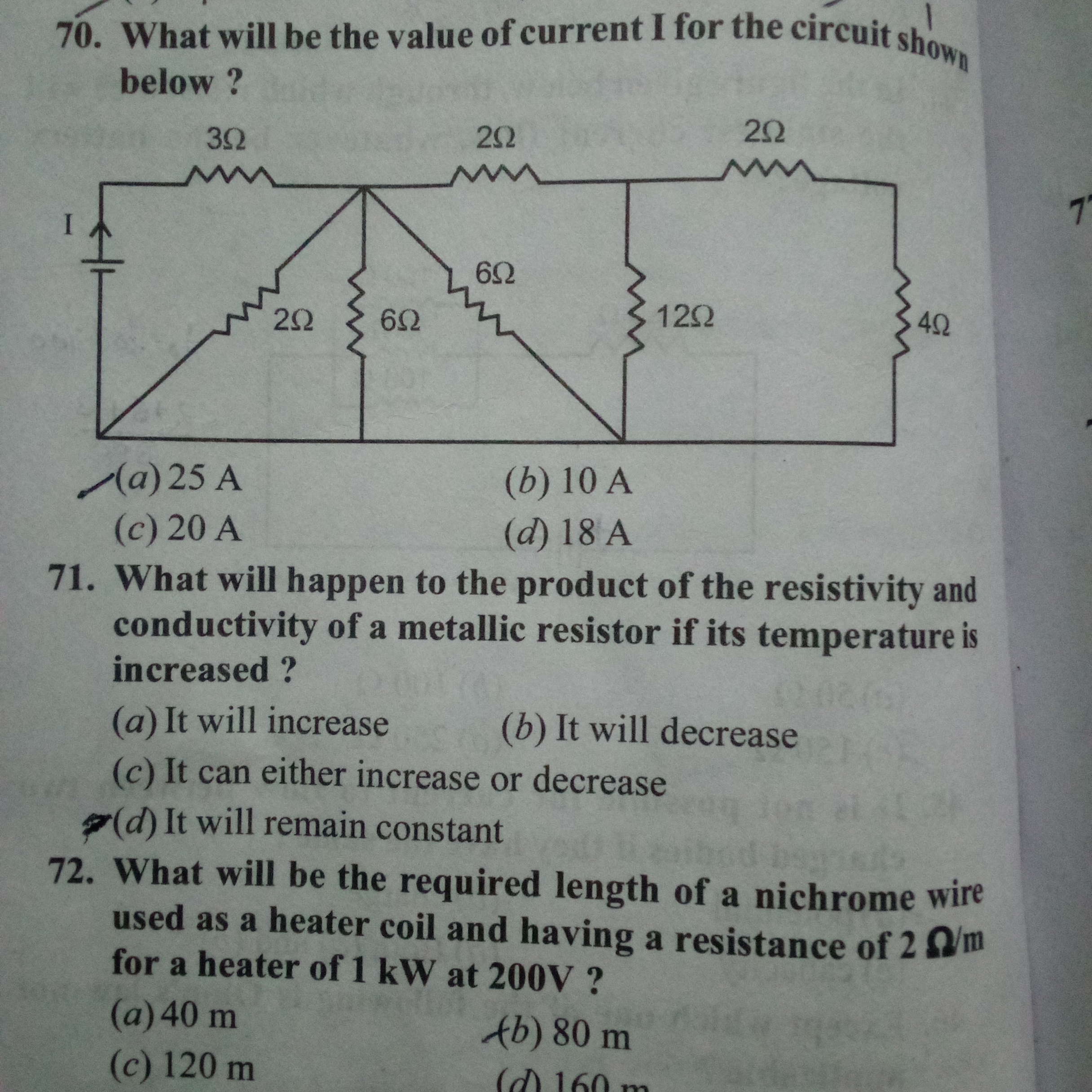
What will be the value of current I for the circuit shown below? What will happen to the product of the resistivity and conductivity of a metallic resistor if its temperature is in... What will be the value of current I for the circuit shown below? What will happen to the product of the resistivity and conductivity of a metallic resistor if its temperature is increased? What will be the required length of a nichrome wire used as a heater coil and having a resistance of 2 Ω/m for a heater of 1 kW at 200 V? Read more

Steps to Solve

1. Circuit Analysis for Current Calculation

First, determine the total resistance in the circuit. Identify the parallel and series connections of the resistors.

The left part of the circuit with the resistors can be simplified as:

• The 2Ω and 2Ω resistors are in series: $$ R_{s1} = 2Ω + 2Ω = 4Ω $$

• The 6Ω resistor is in parallel with the 4Ω: $$ \frac{1}{R_{p}} = \frac{1}{R_{s1}} + \frac{1}{6Ω} $$

Calculating it gives: $$ \frac{1}{R_{p}} = \frac{1}{4} + \frac{1}{6} = \frac{3}{12} + \frac{2}{12} = \frac{5}{12} $$ Thus: $$ R_{p} = \frac{12}{5}Ω = 2.4Ω $$

2. Total Resistance of Entire Circuit

Next, calculate the total resistance of the circuit including the resistances on the right-hand side:

• The total resistance for the right side (12Ω and 4Ω in series) is: $$ R_{s2} = 12Ω + 4Ω = 16Ω $$

Combine this with the previously calculated parallel resistance: $$ R_{total} = R_{p} + R_{s2} = 2.4Ω + 16Ω = 18.4Ω $$

3. Using Ohm's Law to Find Current

Assuming the voltage supply in the circuit is given (not shown in the question, normally denoted as V), use Ohm's law to find the current $I$: $$ I = \frac{V}{R_{total}} $$

If $V$ is not given, we cannot find the specific current value. But using $V = 110V$, we can find: $$ I = \frac{110V}{18.4Ω} \approx 5.98A $$

However, if the actual voltage or other parameters were to lead to an exact required multiple-choice answer (like 25 A), check methodology for combining the resistors or re-evaluate the voltage.