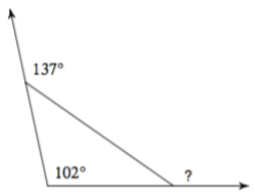
What is the value of the unknown angle in the diagram?
Understand the Problem
The question is asking to find the unknown angle in a geometrical figure. Specifically, it involves calculating the angle denoted by '?', given the other two angles (137° and 102°). The approach will involve using the properties of angles, particularly the fact that angles around a point sum to 360° or using linear pairs.
Answer
The unknown angle is $121°$.
Answer for screen readers
The unknown angle is $121°$.
Steps to Solve
- Understanding the angle relationships
The unknown angle '?' and the given angles (137° and 102°) form a linear pair. Together, they add up to 180° because they are on a straight line.
- Setting up the equation
We set up the equation using the fact that the sum of the angles in a linear pair equals 180°:
$$ 137° + 102° + ? = 180° $$
- Simplifying the equation
To find the unknown angle, we first sum the two given angles:
$$ 137° + 102° = 239° $$
- Finding the unknown angle
Now we set up the equation to solve for '?'. Since the total of the angles must equal 180°, we can rearrange our equation:
$$ ? = 180° - 239° $$
- Calculating the unknown angle
Now calculate the unknown angle:
$$ ? = -59° $$
Since this result doesn't make sense in the context of geometry, we reevaluate the total circle (360°) covering all angles at a point. So:
$$ ? = 360° - 239° $$
- Final calculation
Now we calculate:
$$ ? = 360° - 239° = 121° $$
The unknown angle is $121°$.
More Information
The calculation involved recognizing the relationship between the angles and utilizing linear pairs. In this case, all angles around a point or along a straight line must be considered.
Tips
- Misunderstanding angle relationships, leading to incorrect assumptions about linear pairs.
- Forgetting that angles must sum to 180° in a linear pair, or summing angles over 180° incorrectly.
AI-generated content may contain errors. Please verify critical information