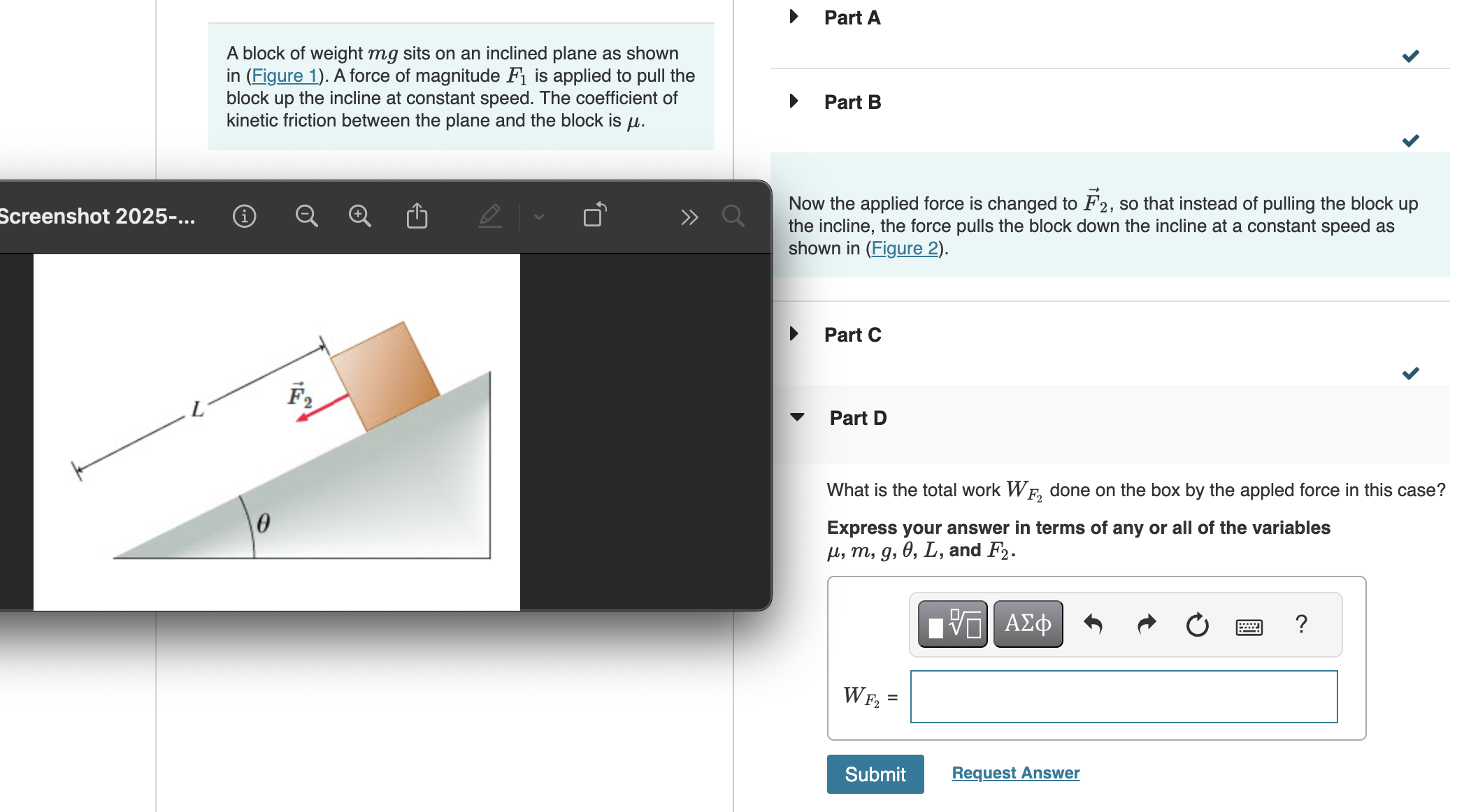
What is the total work W_{F2} done on the box by the applied force in this case? Express your answer in terms of any or all of the variables \, \mu, m, g, \theta, L, and F_{2}.

Steps to Solve

1. Identify the forces acting on the block

The forces acting on the block on the inclined plane are:

• Weight of the block: $W = mg$, acting vertically downward.
• Normal force $N$, acting perpendicular to the plane.
• Frictional force $f_k$, acting opposite to the direction of motion.
• Applied force $F_2$, pulling the block down the incline.

2. Calculate the frictional force

The frictional force is given by the equation: $$ f_k = \mu N $$

Where $N$ is the normal force. The normal force on an incline can be calculated as: $$ N = mg \cos(\theta) $$

Therefore, the kinetic friction force becomes: $$ f_k = \mu mg \cos(\theta) $$

3. Set up the net forces along the plane

Since the block is moving at a constant speed, the net force is zero: $$ F_2 - mg \sin(\theta) - f_k = 0 $$

Substituting for $f_k$, we have: $$ F_2 - mg \sin(\theta) - \mu mg \cos(\theta) = 0 $$

Rearranging gives: $$ F_2 = mg \sin(\theta) + \mu mg \cos(\theta) $$

4. Express the work done by the force (F_2)

Work done by the force (F_2) while moving a distance (L) down the incline is given by: $$ W_{F_2} = F_2 \cdot L $$

Substituting for (F_2), we get: $$ W_{F_2} = (mg \sin(\theta) + \mu mg \cos(\theta)) \cdot L $$

5. Final expression for the work done

Therefore, the total work done on the box by the applied force (F_2) is: $$ W_{F_2} = mgL(\sin(\theta) + \mu \cos(\theta)) $$