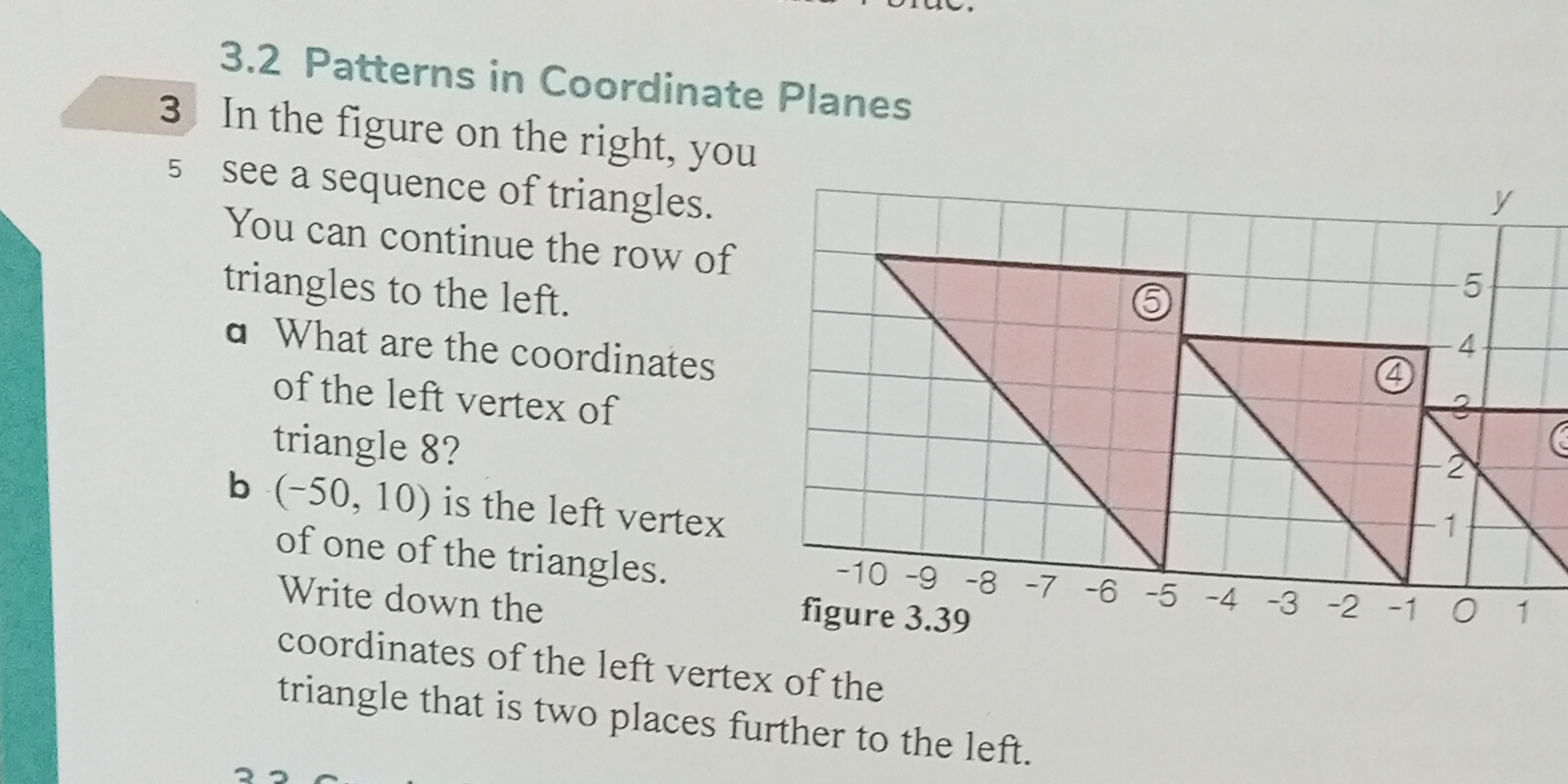
What are the coordinates of the left vertex of triangle 8? (-50, 10) is the left vertex of one of the triangles. Write down the coordinates of the left vertex of the triangle that... What are the coordinates of the left vertex of triangle 8? (-50, 10) is the left vertex of one of the triangles. Write down the coordinates of the left vertex of the triangle that is two places further to the left.
Understand the Problem
The question is asking for the coordinates of specific vertices of triangles within a sequence shown in a coordinate plane. The first part seeks to find the left vertex of triangle 8, and the second part involves determining the coordinates of another vertex based on a given point.
Answer
a) $(-8, 0)$; b) $(-52, 10)$
Answer for screen readers
a) The coordinates of the left vertex of triangle 8 are $(-8, 0)$.
b) The coordinates of the left vertex of the triangle that is two places further to the left are $(-52, 10)$.
Steps to Solve
-
Identify the coordinate pattern From the figure, observe that each triangle has a consistent structure. The left vertex of triangle 8 can be determined by identifying the coordinates of the left vertices of previous triangles in the sequence.
-
Find the left vertex of triangle 8 In the pattern, the left vertices of the triangles are sequentially positioned. The left vertex of triangle 1 is at point (0, 0), triangle 2 is at (-1, 0), and this continues, decreasing by 1 on the x-axis for each subsequent triangle. Therefore, the left vertex of triangle 8 would be at: $$ x = 0 - 8 = -8 $$ Thus, the coordinates of the left vertex are $(-8, 0)$.
-
Determine the vertex 2 places to the left To find the coordinates of the left vertex of the triangle that is two places further to the left of the given point $(-50, 10)$, subtract 2 from the x-coordinate: $$ x = -50 - 2 = -52 $$ The y-coordinate remains unchanged, therefore the coordinates are $(-52, 10)$.
a) The coordinates of the left vertex of triangle 8 are $(-8, 0)$.
b) The coordinates of the left vertex of the triangle that is two places further to the left are $(-52, 10)$.
More Information
The left vertex positions of triangles follow a simple linear pattern on the coordinate plane, decreasing sequentially by one unit along the x-axis for each triangle in a row. This makes it easy to predict the coordinates for any triangle in the sequence.
Tips
- Confusing the coordinate system: Sometimes, students forget that moving left decreases the x-coordinate.
- Overlooking the y-coordinate: In problems asking for vertices, always check if the y-value needs to be adjusted, although in this case it remains constant.
AI-generated content may contain errors. Please verify critical information