What is the sum of the first 20 odd numbers? Also solve the equations: x² + y² = 130 and x² - y² = 32.

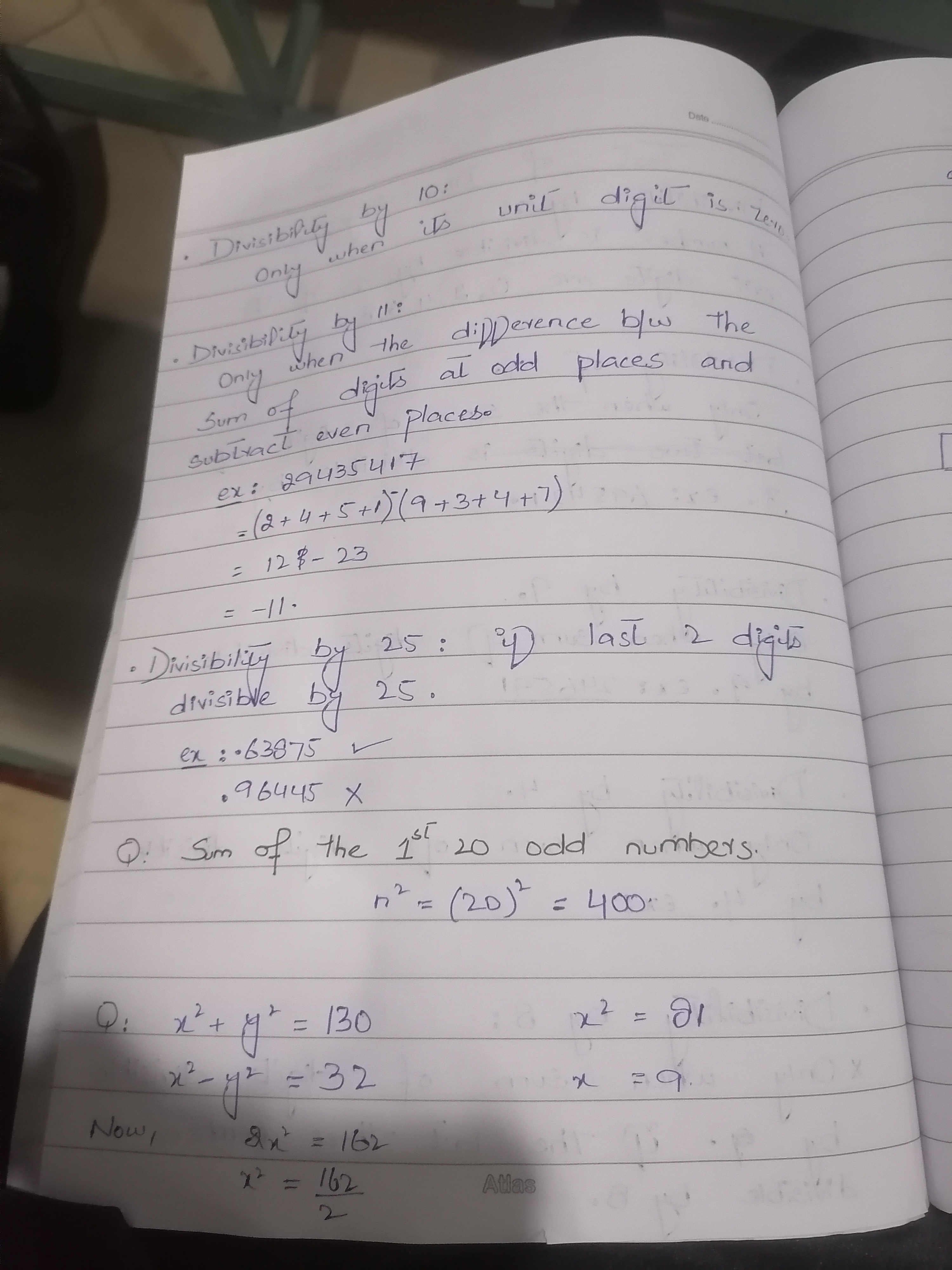
Understand the Problem

The question seems to involve various mathematical concepts related to divisibility rules and algebraic equations, including summing odd numbers and solving for variables in equations. It involves determining whether specific numbers are divisible by 10, 11, and 25, and solving for x given certain equations.

Answer

The sum of the first 20 odd numbers is 400. $ x = 9 $ and $ y = 7 $.

Steps to Solve

Sum of the First 20 Odd Numbers

The sum of the first ( n ) odd numbers can be calculated using the formula ( n^2 ). For the first 20 odd numbers, we have:

$$ n = 20 $$ $$ \text{Sum} = n^2 = 20^2 = 400 $$

Setting Up the System of Equations

From the equations provided, set up the system as follows:

( x^2 + y^2 = 130 )
( x^2 - y^2 = 32 )
Solving for ( x^2 ) and ( y^2 )

To solve for ( x^2 ) and ( y^2 ), add the two equations:

$$ (x^2 + y^2) + (x^2 - y^2) = 130 + 32 $$ This simplifies to:

$$ 2x^2 = 162 $$ Now, divide both sides by 2:

$$ x^2 = \frac{162}{2} = 81 $$

Finding ( y^2 )

Now substitute ( x^2 ) back into the first equation to find ( y^2 ):

$$ 81 + y^2 = 130 $$ Subtract 81 from both sides:

$$ y^2 = 130 - 81 = 49 $$

Calculating ( x ) and ( y )

Now calculate ( x ) and ( y ):

$$ x = \sqrt{81} = 9 $$ $$ y = \sqrt{49} = 7 $$

The sum of the first 20 odd numbers is 400, ( x ) is 9, and ( y ) is 7.

More Information

The sum of the first ( n ) odd numbers always gives ( n^2 ). This is a fundamental result in mathematics, particularly useful in number theory. The values for ( x ) and ( y ) were solved using a system of equations involving squares.

Tips

Not correctly applying the ( n^2 ) formula for the sum of odd numbers.
Confusing the addition and subtraction when solving the system of equations.
Forgetting to isolate variables correctly when solving for ( y^2 ).

AI-generated content may contain errors. Please verify critical information

Thank you for voting!