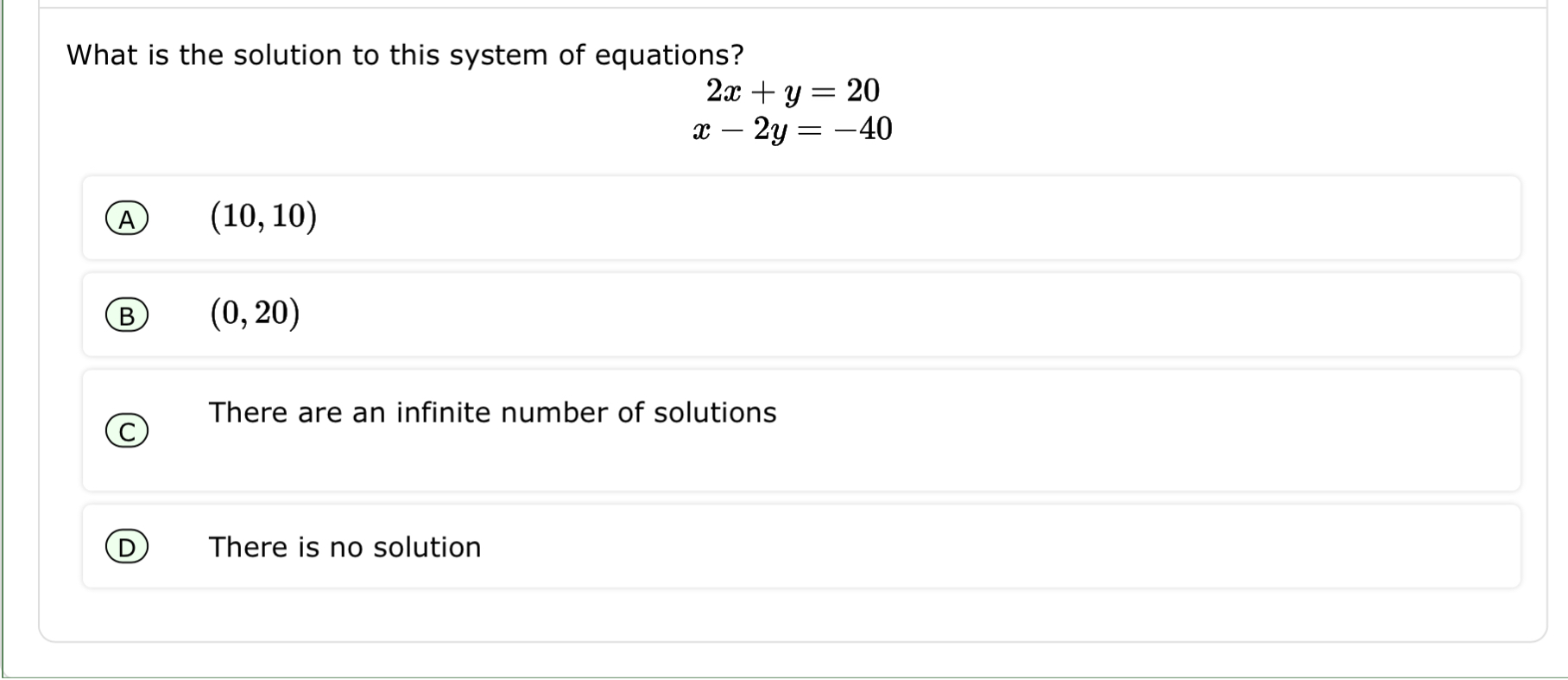
What is the solution to this system of equations? 2x + y = 20 and x - 2y = -40.
Understand the Problem
The question is asking for the solution to a system of equations, which involves determining the values of 'x' and 'y' that satisfy both equations simultaneously. We will need to solve these equations using methods such as substitution or elimination.
Answer
The solution is $(0, 20)$.
Answer for screen readers
The solution to the system of equations is $(0, 20)$.
Steps to Solve
-
Write down the equations
We have the following system of equations:
$$ 2x + y = 20 $$
$$ x - 2y = -40 $$ -
Isolate one variable
Let's isolate $y$ in the first equation:
$$ y = 20 - 2x $$ -
Substitute into the second equation
Now, substitute the expression for $y$ into the second equation:
$$ x - 2(20 - 2x) = -40 $$ -
Simplify the equation
Distribute and simplify:
$$ x - 40 + 4x = -40 $$
Combine like terms:
$$ 5x - 40 = -40 $$ -
Solve for x
Add 40 to both sides:
$$ 5x = 0 $$
Then divide by 5:
$$ x = 0 $$ -
Substitute back to find y
Now substitute $x = 0$ back into the expression for $y$:
$$ y = 20 - 2(0) $$
So:
$$ y = 20 $$ -
Final solution
The solution to the system of equations is:
$$ (x, y) = (0, 20) $$
The solution to the system of equations is $(0, 20)$.
More Information
The point $(0, 20)$ represents the intersection of the two lines defined by the equations. In a graphical context, this means that both equations are satisfied at this point.
Tips
- Failing to substitute properly can lead to incorrect values.
- Not simplifying equations correctly can cause mistakes in the final answer.
- Confusing the variables during substitution can cause wrong results.
AI-generated content may contain errors. Please verify critical information