What is the solution set for the inequality?
Understand the Problem
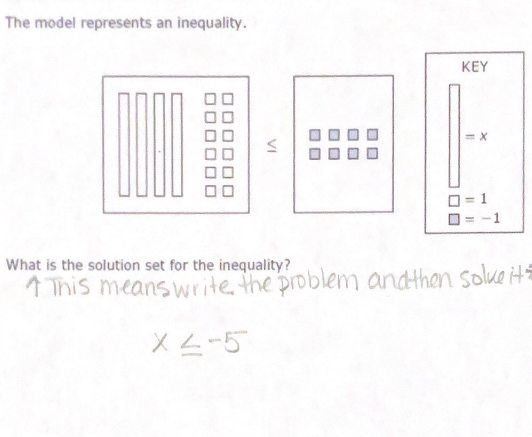
The question is asking for the solution set of an inequality depicted in a model. The user has noted that they need to write the problem and then solve it, indicating that they are looking for a step-by-step solution related to inequalities.
Answer
The solution set is \( (-\infty, -5] \).
Answer for screen readers
The solution set for the inequality is:
$$ (-\infty, -5] $$
Steps to Solve
- Identify the Inequality Represented by the Model
The model shows two groups of counters. The left group has more counters than the right group. According to the key, each blank square (representing the variable ( x )) has a value related to 1 and -1. Based on the arrangement, we can interpret the inequality.
- Write the Inequality
From the model, it can be observed that the left has 6 counters (as indicated by the 3 filled bars) while the right has 2 counters (presented in 2 filled boxes). Thus, the inequality can be expressed as:
$$ x \leq -5 $$
- Solve the Inequality
In this context, the inequality ( x \leq -5 ) means that ( x ) can take any value that is less than or equal to -5.
- Express the Solution Set
The solution set represents all values of ( x ) that satisfy the inequality. This can be written in interval notation as:
$$ (-\infty, -5] $$
This indicates that all numbers less than or equal to -5 are included in the solution.
The solution set for the inequality is:
$$ (-\infty, -5] $$
More Information
The inequality represents a situation in which ( x ) must be less than or equal to -5. The solution set denotes all real numbers from negative infinity up to and including -5.
Tips
- Misinterpreting the model: Ensure that the differences in the number of counters are clearly understood to avoid incorrect inequality signs.
- Confusing direction of the inequality: Be careful to correctly interpret whether ( x ) should be less than or greater than the given value.
AI-generated content may contain errors. Please verify critical information