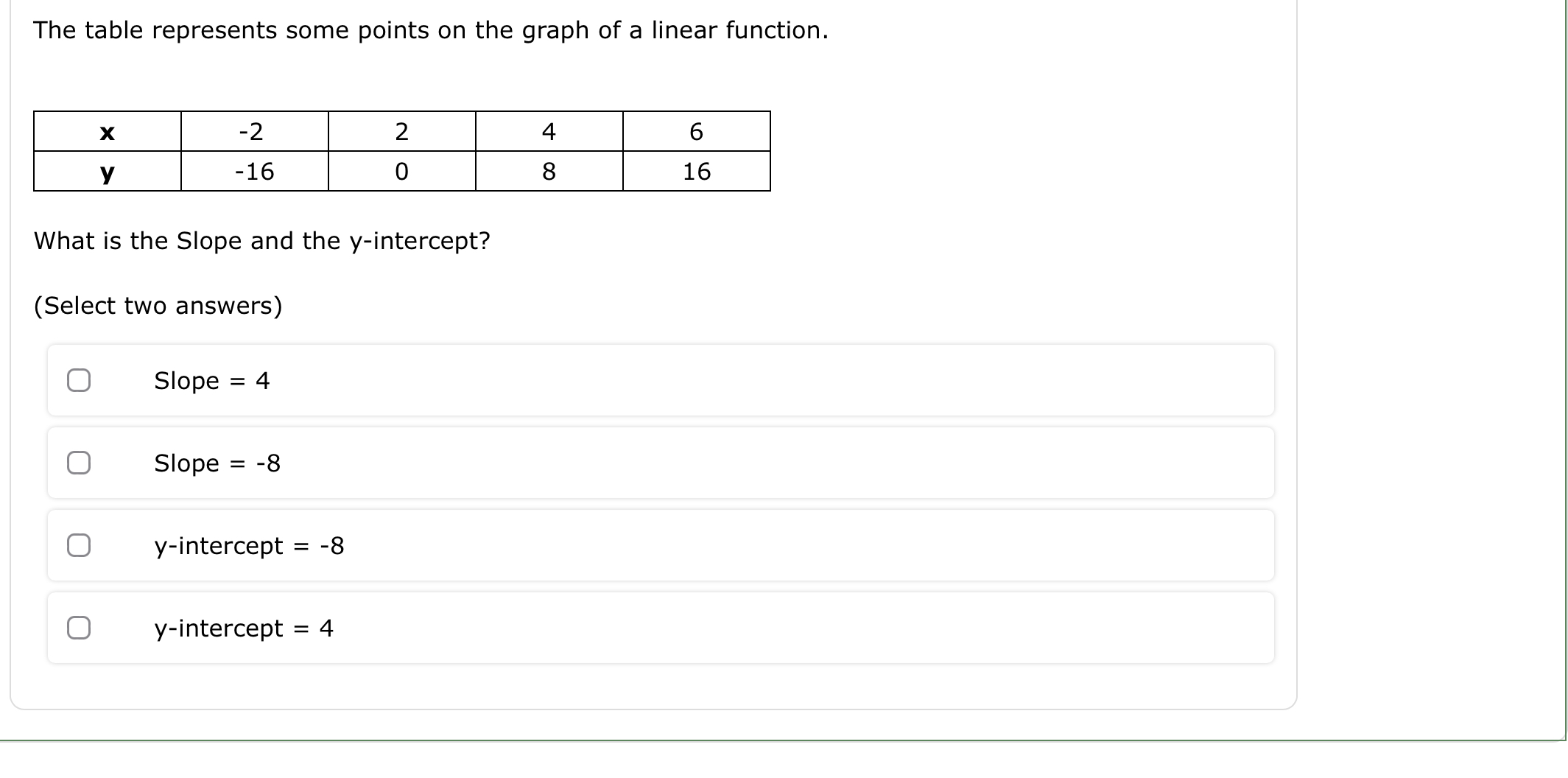
What is the slope and the y-intercept?
Understand the Problem
The question asks for the slope and y-intercept of a linear function represented by a table of values. This requires calculating the slope using the differences in the y-values and x-values and finding the y-intercept based on the line equation.
Answer
Slope = 4, y-intercept = -8
Answer for screen readers
- Slope = 4
- y-intercept = -8
Steps to Solve
-
Identify points from the table The given points from the table are:
- When $x = -2$, $y = -16$
- When $x = 2$, $y = 0$
- When $x = 4$, $y = 8$
- When $x = 6$, $y = 16$
-
Calculate the slope The formula for slope $m$ between two points $(x_1, y_1)$ and $(x_2, y_2)$ is given by:
$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$
Using the points $(2, 0)$ and $(4, 8)$:
$$ m = \frac{8 - 0}{4 - 2} = \frac{8}{2} = 4 $$
-
Verify slope with another pair of points Let's also verify using the points $(2, 0)$ and $(6, 16)$:
$$ m = \frac{16 - 0}{6 - 2} = \frac{16}{4} = 4 $$
The slope is consistent and equals to 4.
-
Determine the y-intercept The y-intercept occurs when $x = 0$. We can use the equation of the line in slope-intercept form, $y = mx + b$.
Using the slope $m = 4$ and the point $(2, 0)$:
Substitute $x = 2$ and $y = 0$:
$$ 0 = 4(2) + b \implies 0 = 8 + b \implies b = -8 $$
Therefore, the y-intercept is $-8$.
- Slope = 4
- y-intercept = -8
More Information
The slope indicates the rate of change of $y$ with respect to $x$. A slope of 4 means for every increase of 1 unit in $x$, $y$ increases by 4 units. The y-intercept, $-8$, tells us where the line crosses the $y$-axis.
Tips
- Not using distinct points: Ensure you are using two different points to calculate the slope.
- Incorrect calculations: Double-check subtraction and division steps when calculating slope and intercept.
AI-generated content may contain errors. Please verify critical information