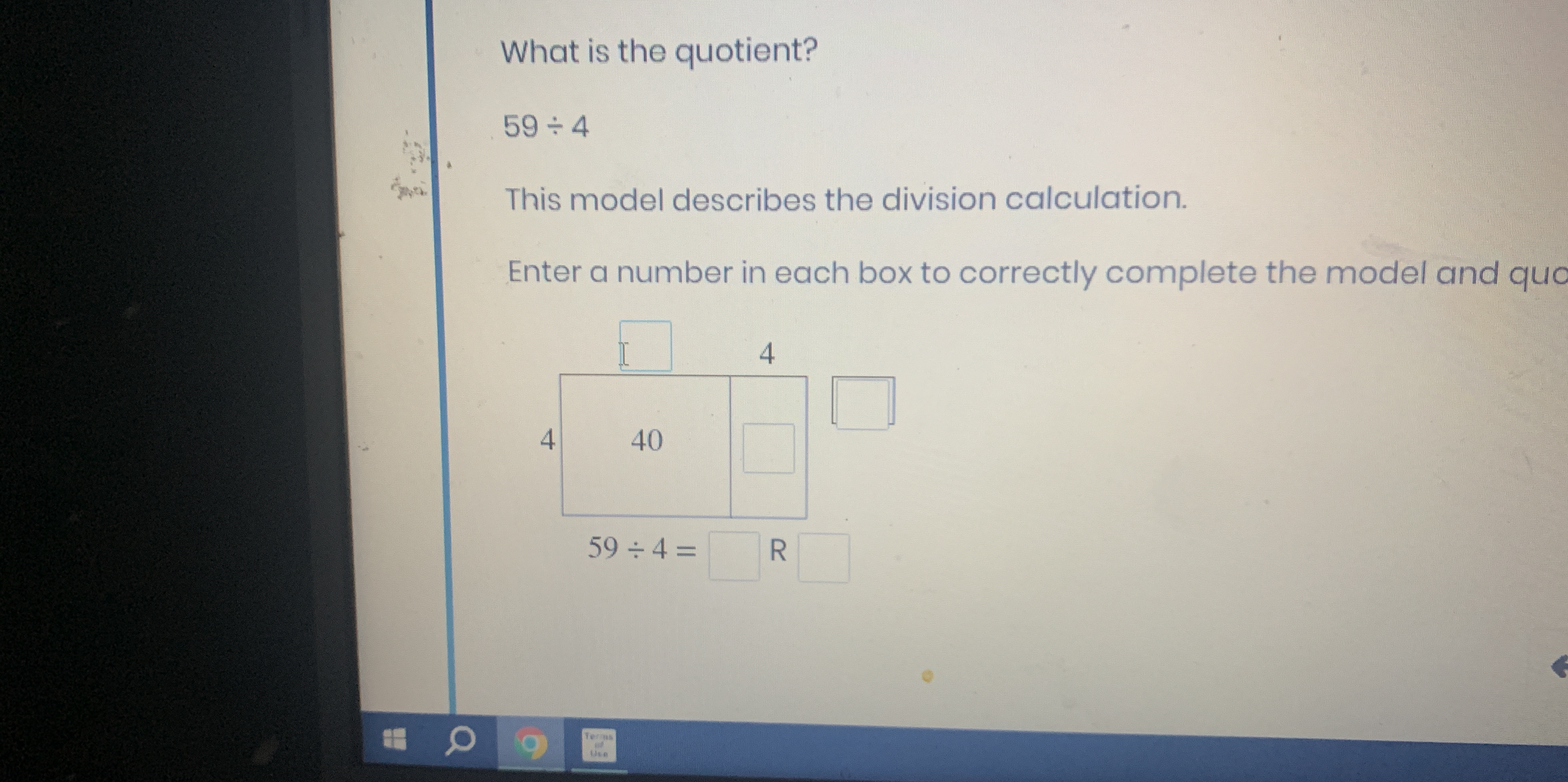
What is the quotient of 59 ÷ 4?

Understand the Problem

The question is asking for the quotient of the division operation 59 ÷ 4. It also requires filling in a model that represents this division, using boxes to indicate the dividend, divisor, and quotient.

Answer

The quotient is $14$ with a remainder of $3$. Thus, $59 \div 4 = 14 \text{ R } 3$.

Steps to Solve

Set Up the Division Problem
We start by writing the division problem:
$$ 59 \div 4 $$
Perform the Division
Next, we divide 59 by 4:

4 goes into 5 once, which gives us a product of (4 \times 1 = 4).
Subtract this from 5:
$$ 5 - 4 = 1 $$
Bring down the next digit (9), making it 19.
Now, divide 19 by 4: 4 goes into 19 four times, which gives us (4 \times 4 = 16).
Subtract:
$$ 19 - 16 = 3 $$

Identify the Quotient and Remainder
The quotient of the division is what's left after the whole number division.
So, the complete division is:
$$ 59 \div 4 = 14 \quad \text{R } 3 $$
Here, (14) is the quotient and (3) is the remainder.
Fill the Model Boxes
Now, we fill in the model:

The dividend (number being divided) is 59.
The divisor (number dividing) is 4.
The quotient (result of the division) is 14.
The remainder (R) is 3.

The quotient is (14) with a remainder of (3).

So, (59 \div 4 = 14 \text{ R } 3).

More Information

In this division, (59) is known as the dividend, (4) is called the divisor, and (14) is the quotient. The (3) is the remainder since (59) is not exactly divisible by (4).

Tips

Forgetting to bring down the next digit when the first digit of the dividend is smaller than the divisor.
Confusing the remainder with the quotient. Always check to ensure the remainder is less than the divisor.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!