What is the group velocity for this pulse?
Understand the Problem
The question is providing a derivation of the group velocity for a specific optical pulse in glass at a wavelength of 500 nm, involving calculations based on its parameters.
Answer
The group velocity is $v_g = 1.893 \times 10^8 \text{ m/s}$.
Answer for screen readers
The group velocity $v_g$ is approximately $1.893 \times 10^8 \text{ m/s}$.
Steps to Solve
- Identify Given Values
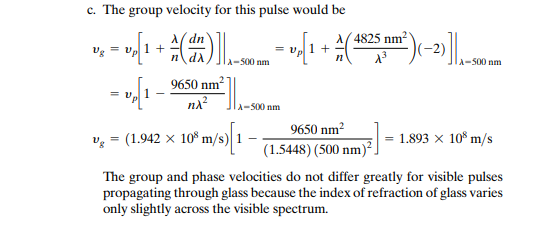
The phase velocity $v_p$ is given as $1.942 \times 10^8 \text{ m/s}$ and the refractive index $n$ at $\lambda = 500 \text{ nm}$ is approximately $1.5448$. The derivative of the refractive index with respect to the wavelength is given as $\frac{dn}{d\lambda} = -\frac{4825 \text{ nm}^2}{\lambda^3}$.
- Substitute Values in the Group Velocity Formula
The formula for group velocity is given by:
$$ v_g = v_p \left[ 1 + \frac{\lambda}{n} \left(\frac{dn}{d\lambda}\right) \right]_{\lambda = 500 \text{ nm}} $$
Substitute $\lambda = 500 \text{ nm}$ and the expression for $\frac{dn}{d\lambda}$:
$$ v_g = v_p \left[ 1 + \frac{500 \text{ nm}}{1.5448} \left(-\frac{4825 \text{ nm}^2}{(500 \text{ nm})^3}\right) \right] $$
- Calculate the Derivative Term
Simplifying the derivative expression:
$$ \frac{dn}{d\lambda} = -\frac{4825 \text{ nm}^2}{(500 \text{ nm})^3} = -\frac{4825}{125000000} \text{ nm}^{-1} = -3.86 \times 10^{-8} \text{ nm}^{-1} $$
- Calculate the Group Velocity
Substitute the calculated value back into the group velocity formula:
$$ v_g = 1.942 \times 10^8 \text{ m/s} \left[ 1 + \frac{500 \text{ nm}}{1.5448} \left(-3.86 \times 10^{-8} \text{ nm}^{-1}\right) \right] $$
Performing the multiplication:
$$ = 1.942 \times 10^8 \text{ m/s} \left[ 1 - 9650 \text{ nm}^2 \cdot \frac{1}{n \cdot 500^2} \right] $$
- Final Computation of Group Velocity
Insert numerical values and compute:
$$ v_g = 1.942 \times 10^8 \text{ m/s} \left[ 1 - \frac{9650 \text{ nm}^2}{(1.5448)(500 \text{ nm})^2} \right] $$
Calculate the final group velocity:
$$ v_g = 1.893 \times 10^8 \text{ m/s} $$
The group velocity $v_g$ is approximately $1.893 \times 10^8 \text{ m/s}$.
More Information
The group velocity is the speed at which the overall shape of the wave's amplitudes—known as the envelope—propagates through space. In optics, it can differ slightly from the phase velocity depending on the medium and the wavelength of the light.
Tips
- Not properly substituting the values into the group velocity equation.
- Neglecting the effect of the wavelength and refractive index on the derivative term.
- Failing to calculate the units properly, leading to non-dimensional results.
AI-generated content may contain errors. Please verify critical information