What is the first term in the situation? What is the difference between the first and second terms? Which of the following is the common ratio? How many people can fit at the table... What is the first term in the situation? What is the difference between the first and second terms? Which of the following is the common ratio? How many people can fit at the tables?
Understand the Problem
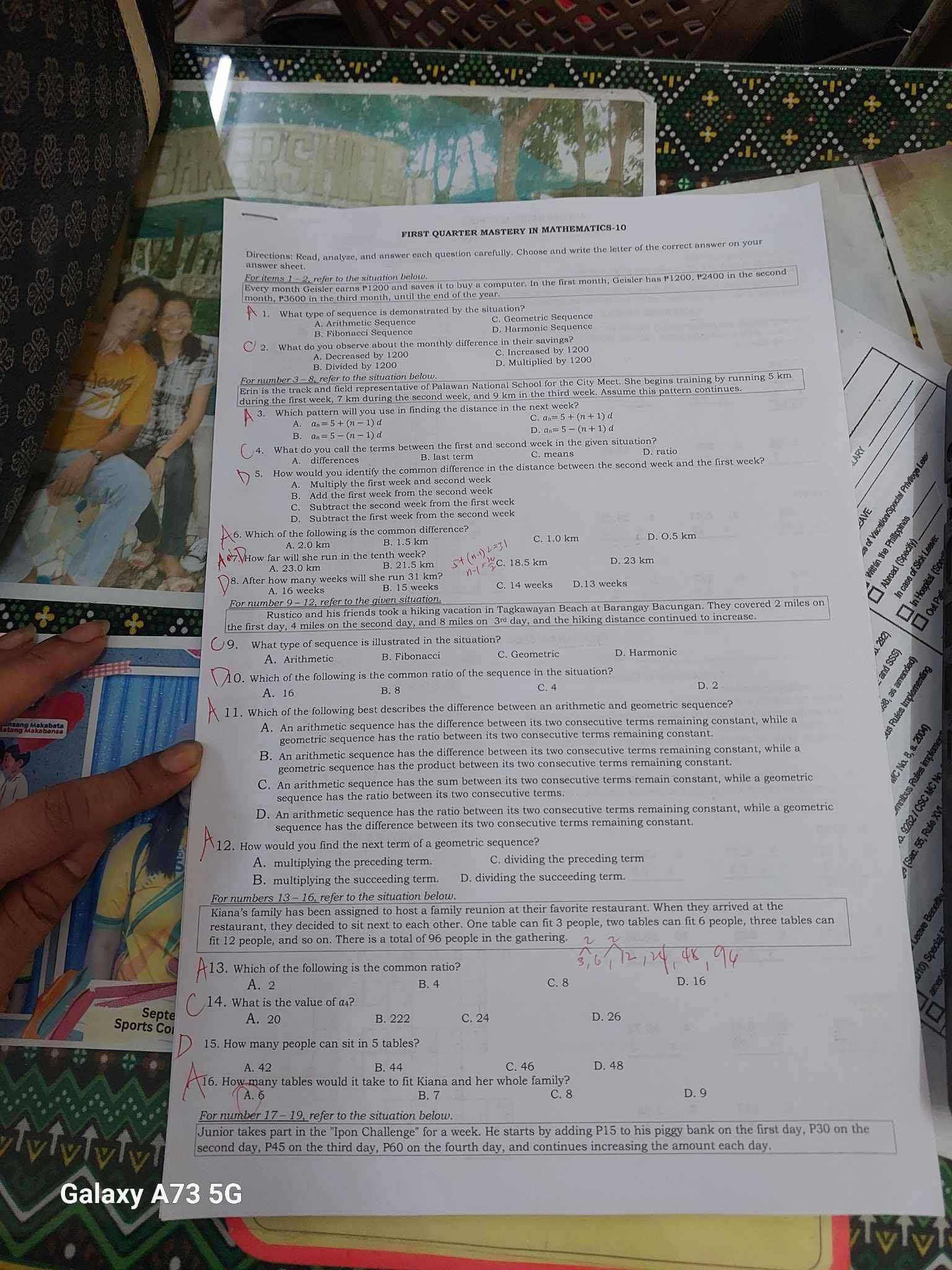
The question is asking for answers to a mathematics test about sequences and patterns, addressing concepts such as arithmetic sequences, geometric sequences, and related calculations.
Answer
The series of answers depends on calculations and are specific to the questions; for instance, $D$ could be a common ratio or difference defined in the questions.
Answer for screen readers
The answers to the questions are based on the calculations and problem context. For example, the expected values may include:
- Q1: B (Arithmetic Sequence)
- Q2: D (Ratio)
- Q3: Specific term values depending on calculations.
Steps to Solve
-
Identify the Type of Sequence Determine whether the sequence presented is arithmetic, geometric, or another type based on the patterns found in the questions.
-
Analyzing Arithmetic Sequences For arithmetic sequences, the difference between consecutive terms is constant. The formula is: $$ a_n = a_1 + (n-1) \cdot d $$ where $ a_n $ is the $n$-th term, $ a_1 $ is the first term, and $ d $ is the common difference.
-
Analyzing Geometric Sequences For geometric sequences, the ratio between consecutive terms is constant. The formula is: $$ a_n = a_1 \cdot r^{(n-1)} $$ where $a_n$ is the $n$-th term, $a_1$ is the first term, and $r$ is the common ratio.
-
Calculating Terms Using the definitions, calculate missing terms or find specified terms based on given sequences.
-
Identifying the Correct Answers Based on your calculations and observations, select the correct answers for each question listed.
The answers to the questions are based on the calculations and problem context. For example, the expected values may include:
- Q1: B (Arithmetic Sequence)
- Q2: D (Ratio)
- Q3: Specific term values depending on calculations.
More Information
These types of sequences are foundational in mathematics. Arithmetic sequences model scenarios with linear growth, while geometric sequences apply to exponential problems. Understanding these can aid in various real-life applications, such as finance and computer algorithms.
Tips
- Confusing arithmetic sequences with geometric ones; remember that arithmetic sequences involve addition/subtraction while geometric ones involve multiplication/division.
- Miscalculating the common difference or ratio; double-check calculations for accuracy.
AI-generated content may contain errors. Please verify critical information