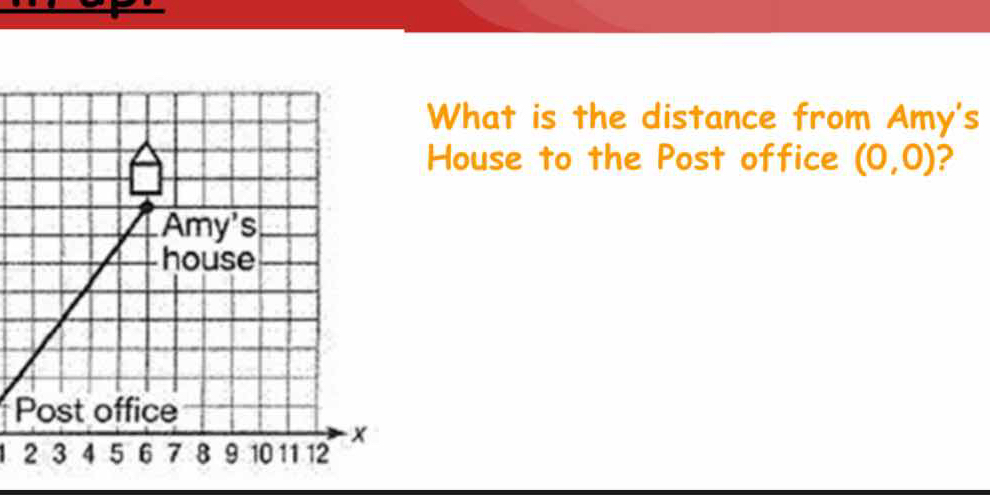
What is the distance from Amy's House to the Post office (0,0)?
Understand the Problem
The question is asking for the calculation of the distance between two points on a coordinate grid: Amy's house and the Post office located at (0,0). We will use the distance formula to solve this.
Answer
The distance from Amy's house to the Post office is $5$.
Answer for screen readers
The distance from Amy's house to the Post office is $5$.
Steps to Solve
-
Identify Coordinates of Points Identify the coordinates of Amy's house and the Post office.
Assuming Amy's house is located at coordinates $(x_1, y_1)$. -
Apply the Distance Formula Use the distance formula, which is given by:
$$ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} $$
For the Post office at $(0,0)$, we have:
$$ d = \sqrt{(0 - x_1)^2 + (0 - y_1)^2} $$
This simplifies to:
$$ d = \sqrt{x_1^2 + y_1^2} $$ -
Substitute Coordinates Next, substitute the specific coordinates of Amy's house into the formula.
For example, if Amy's house is at $(3, 4)$, we would substitute:
$$ d = \sqrt{3^2 + 4^2} $$ -
Calculate Distance Perform the calculations:
$$ d = \sqrt{9 + 16} = \sqrt{25} = 5 $$
Determine the final distance.
The distance from Amy's house to the Post office is $5$.
More Information
Distance in a coordinate plane is calculated using the distance formula, which finds the straight-line distance between two points. This method is widely applicable in various fields including geometry, physics, and engineering.
Tips
- Incorrect Coordinates: Mistakenly using the wrong coordinates for either point can lead to an incorrect answer. Always double-check the coordinates.
- Math Errors: Failing to correctly square the numbers or miscalculating the square root can lead to wrong results. Review each arithmetic step carefully.
AI-generated content may contain errors. Please verify critical information