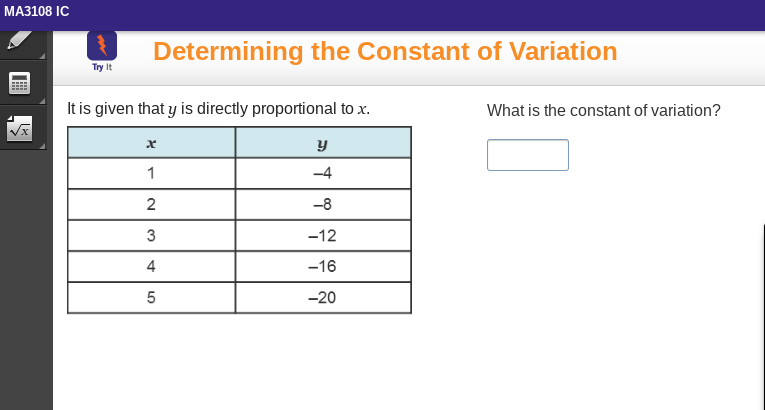
What is the constant of variation when y is directly proportional to x?
Understand the Problem
The question is asking for the constant of variation given that y is directly proportional to x, using the provided values in a table. To find the constant of variation, we need to identify the relationship between x and y based on the proportionality.
Answer
The constant of variation is $k = -4$.
Answer for screen readers
The constant of variation is $k = -4$.
Steps to Solve
- Understand the direct proportionality relationship
Since $y$ is directly proportional to $x$, we can express this relationship using the equation: $$ y = kx $$ where $k$ is the constant of variation.
- Substitute values to find the constant $k$
To find $k$, we can use any pair of corresponding values from the table. Let's use the first row where $x = 1$ and $y = -4$: $$ -4 = k(1) $$
- Solve for $k$
By rearranging the equation, we can solve for $k$: $$ k = -4 $$
- Verify with other values
We should check that this value holds for other pairs in the table. For example, for $x = 2$: $$ y = kx $$ $$ y = -4(2) = -8 $$
This is correct, so we can check another pair, such as $x = 3$: $$ y = -4(3) = -12 $$
All values confirm that $k = -4$.
The constant of variation is $k = -4$.
More Information
The constant of variation represents the ratio of $y$ to $x$ when $y$ is directly proportional to $x$. In this case, $-4$ indicates that as $x$ increases, $y$ decreases at a rate of 4 times the corresponding $x$ value.
Tips
- Confusing direct variation with inverse variation; remember that in direct variation, if $x$ increases, $y$ also changes in a consistent manner according to the constant $k$.
- Forgetting to check with multiple values can lead to assuming the constant is correct based on only one set of values.
AI-generated content may contain errors. Please verify critical information