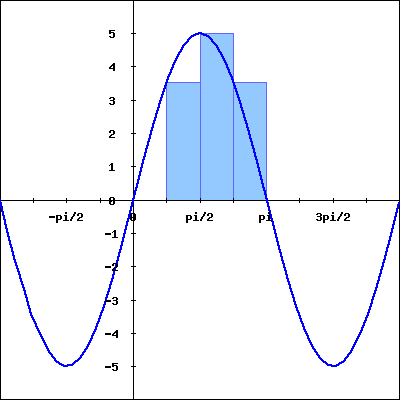
What does the graph of the sine function depicted here represent?

Steps to Solve

1. Identify Key Features of the Graph

In the given graph, we see a sine function plotted, denoted by $y = \sin(x)$. The key points of interest include the peaks and troughs, specifically at $x = \frac{\pi}{2}$ (maximum point), $x = \frac{3\pi}{2}$ (minimum point), and $x = -\frac{\pi}{2}$ (minimum point).

2. Understand the Shaded Areas

The blue shaded areas represent the area under the curve of the sine function between specific limits. To find the shaded area, we need to calculate the definite integral of the sine function over those intervals.

3. Calculate the Area under the Curve

For the intervals indicated by the shaded areas, we need to set up the integral. If the shaded area occurs from $x = 0$ to $x = \frac{\pi}{2}$, we compute:

$$ \text{Area} = \int_0^{\frac{\pi}{2}} \sin(x) , dx $$

4. Evaluate the Integral

To evaluate the integral:

$$ \int \sin(x) , dx = -\cos(x) + C $$

Thus,

$$ \text{Area} = \left[-\cos(x)\right]_0^{\frac{\pi}{2}} = -\cos\left(\frac{\pi}{2}\right) - (-\cos(0)) = 0 + 1 = 1 $$

5. Interpret the Result

The area under the sine function from $0$ to $\frac{\pi}{2}$ is equal to 1. This indicates the total area of the first shaded region.