What are the terms and definitions related to first-order linear differential equations?
Understand the Problem
The question appears to list definitions and concepts related to first-order linear differential equations, likely requiring explanations or selections of terms in the context of differential equations.
Answer
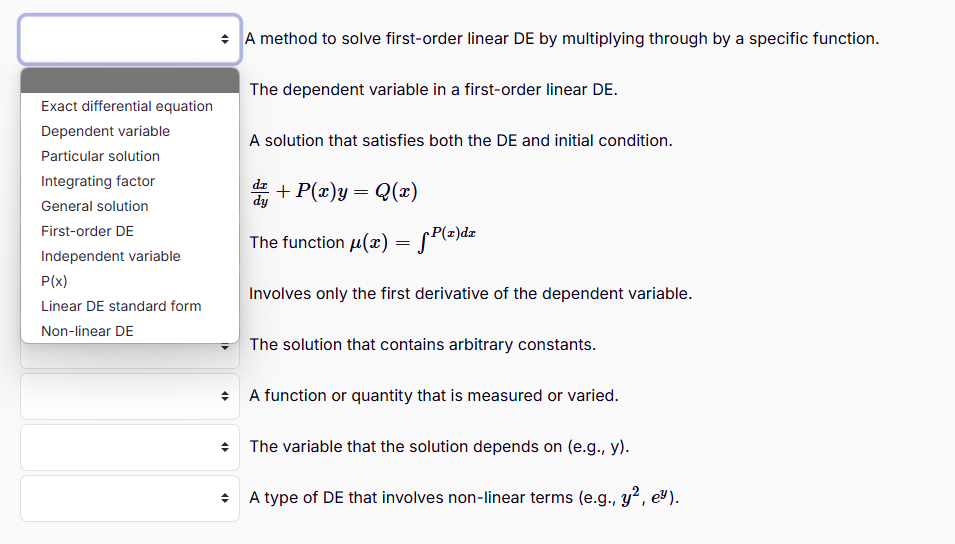
Integrating factor; Dependent variable; Particular solution; First-order DE; Integrating factor; General solution; Independent variable; Dependent variable; Non-linear DE.
A method to solve first-order linear DE by multiplying through by a specific function: Integrating factor. The dependent variable in a first-order linear DE: Dependent variable. A solution that satisfies both the DE and initial condition: Particular solution. ( \frac{dx}{dy} + P(x)y = Q(x) ) involves only the first derivative: First-order DE. The function ( \mu(x) = \int P(x)dx ): Integrating factor. The solution that contains arbitrary constants: General solution. A function or quantity that is measured or varied: Independent variable. The variable that the solution depends on (e.g., y): Dependent variable. A type of DE that involves non-linear terms (e.g., ( y^2, e^y )): Non-linear DE.
Answer for screen readers
A method to solve first-order linear DE by multiplying through by a specific function: Integrating factor. The dependent variable in a first-order linear DE: Dependent variable. A solution that satisfies both the DE and initial condition: Particular solution. ( \frac{dx}{dy} + P(x)y = Q(x) ) involves only the first derivative: First-order DE. The function ( \mu(x) = \int P(x)dx ): Integrating factor. The solution that contains arbitrary constants: General solution. A function or quantity that is measured or varied: Independent variable. The variable that the solution depends on (e.g., y): Dependent variable. A type of DE that involves non-linear terms (e.g., ( y^2, e^y )): Non-linear DE.
More Information
These terms are fundamental to understanding and solving first-order linear differential equations, which are critical in many scientific and engineering applications.
Tips
Common mistakes include misidentifying the independent and dependent variables and confusing particular and general solutions.
AI-generated content may contain errors. Please verify critical information