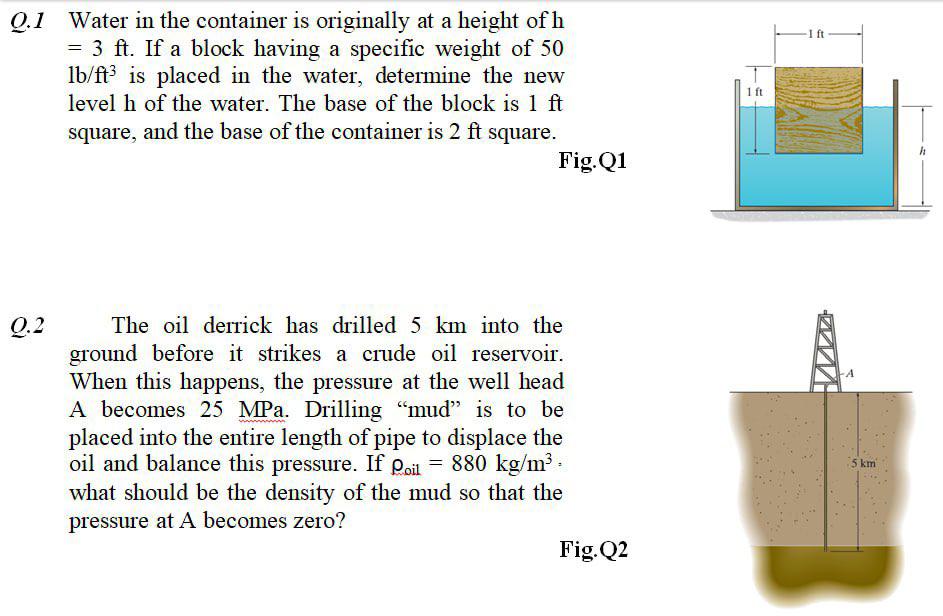
Water in the container is originally at a height of h = 3 ft. If a block having a specific weight of 50 lb/ft³ is placed in the water, determine the new level h of the water. The b... Water in the container is originally at a height of h = 3 ft. If a block having a specific weight of 50 lb/ft³ is placed in the water, determine the new level h of the water. The base of the block is 1 ft square, and the base of the container is 2 ft square. The oil derrick has drilled 5 km into the ground before it strikes a crude oil reservoir. When this happens, the pressure at the well head A becomes 25 MPa. Drilling mud is to be placed into the entire length of the pipe to displace the oil and balance this pressure. If ρ_oil = 880 kg/m³, what should be the density of the mud so that the pressure at A becomes zero? Read more

Steps to Solve

1. Calculate Volume Displaced by the Block

The volume displaced by the block can be calculated using its weight and specific weight.

The weight of the block is given as $50 , \text{lb/ft}^3$, and the specific weight ($\gamma$) is defined as:

$$ V_{\text{displaced}} = \frac{W_{\text{block}}}{\gamma} $$

Where $W_{\text{block}} = 50 , \text{lb}$.

Using the specific weight of water ($\gamma_{\text{water}} = 62.4 , \text{lb/ft}^3$), we find the volume displaced:

$$ V_{\text{displaced}} = \frac{50}{62.4} \approx 0.8032 , \text{ft}^3. $$

2. Determine the New Water Level

The base area of the container is $2 , \text{ft}^2$. The increase in water level ($h_{\text{new}}$) due to the displaced volume is:

$$ h_{\text{new}} = \frac{V_{\text{displaced}}}{A_{\text{base}}} $$

Where $A_{\text{base}} = 2 , \text{ft}^2$.

Substituting the values:

$$ h_{\text{new}} = \frac{0.8032}{2} \approx 0.4016 , \text{ft}. $$

3. Calculate Total Height of Water After Submersion

To find the total height of water after submersion:

$$ h_{\text{final}} = h_{\text{initial}} + h_{\text{new}} $$

Where $h_{\text{initial}} = 3 , \text{ft}$. Thus:

$$ h_{\text{final}} = 3 + 0.4016 \approx 3.4016 , \text{ft}. $$

4. Determine Mud Density for Pressure Balancing

Using the hydrostatic pressure formula:

$$ P = \rho g h $$

At depth $h = 5 , \text{km} = 5000 , \text{m}$, we have the pressure at point A as 25 MPa. We need the pressure for the mud to balance this pressure.

For the mud density ($\rho_{\text{mud}}$):

$$ P_{\text{mud}} = \rho_{\text{mud}} g h = 25 \times 10^6 , \text{Pa}. $$

Assuming $g \approx 9.81 , \text{m/s}^2$, we can calculate:

$$ \rho_{\text{mud}} = \frac{25 \times 10^6}{9.81 \times 5000}. $$

Calculating gives:

$$ \rho_{\text{mud}} \approx 510.4 , \text{kg/m}^3. $$