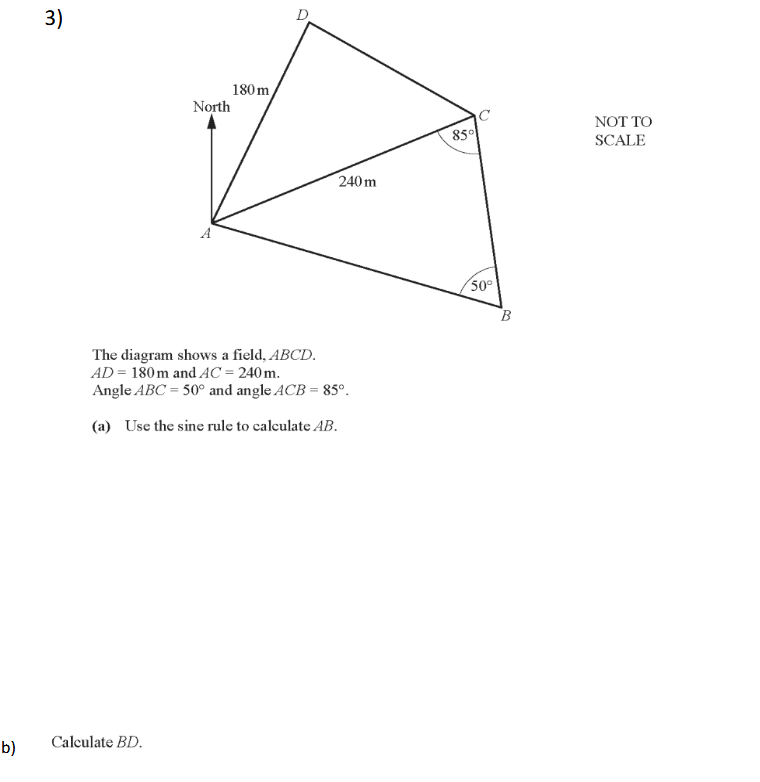
Use the sine rule to calculate AB. Calculate BD.

Steps to Solve

1. Identify the Angles

   In triangle ABC, we have:

   • Angle ABC = $50^\circ$
   • Angle ACB = $85^\circ$

   To find angle CAB, we use the fact that the sum of angles in a triangle is $180^\circ$:

   $$ \text{Angle CAB} = 180^\circ - \text{Angle ABC} - \text{Angle ACB} $$ $$ \text{Angle CAB} = 180^\circ - 50^\circ - 85^\circ = 45^\circ $$

2. Apply the Sine Rule

   The sine rule states that:

   $$ \frac{a}{\sin A} = \frac{b}{\sin B} = \frac{c}{\sin C} $$

   Here, let:

   • $a = AB$, which we want to find,
   • $b = AC = 240, \text{m}$,
   • $c = AD = 180, \text{m}$.

   The corresponding angles are:

   • A = CAB = $45^\circ$
   • B = ABC = $50^\circ$
   • C = ACB = $85^\circ$

   Thus we can write:

   $$ \frac{AB}{\sin 45^\circ} = \frac{240}{\sin 50^\circ} $$

3. Rearrange the Sine Rule

   To find AB, rearrange the equation:

   $$ AB = \frac{240 \cdot \sin 45^\circ}{\sin 50^\circ} $$

4. Calculate Values

   Using the approximate values:

   • $\sin 45^\circ \approx 0.7071$
   • $\sin 50^\circ \approx 0.7660$

   Plug in these values:

   $$ AB = \frac{240 \cdot 0.7071}{0.7660} $$

5. Perform the Calculation

   Calculate AB:

   $$ AB \approx \frac{169.704}{0.7660} \approx 221.5, \text{m} $$