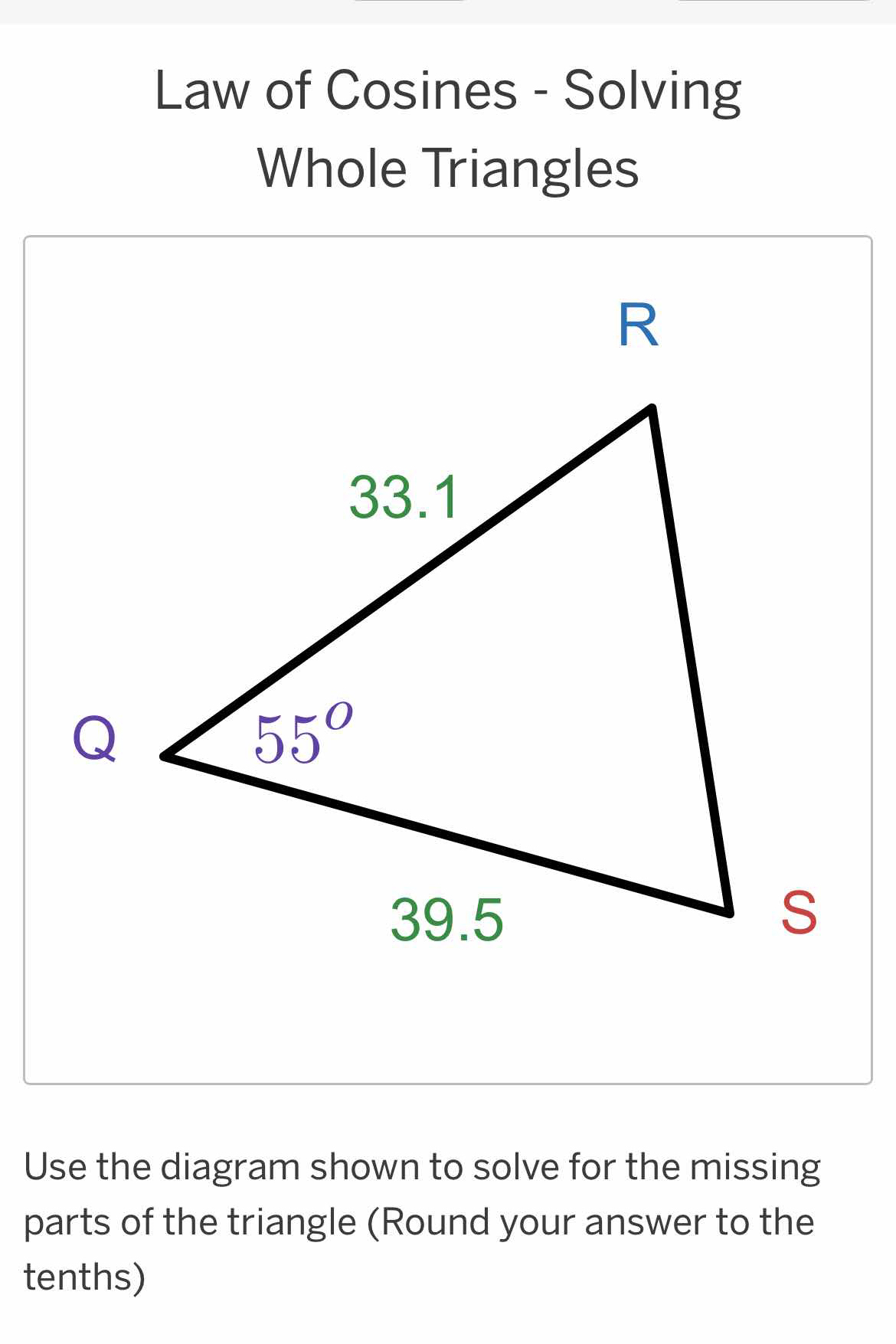
Use the diagram shown to solve for the missing parts of the triangle. Round your answer to the tenths.

Steps to Solve

1. Identify Known Values

Given triangle with sides and angles:

• Side $QS = 39.5$
• Side $QR = 33.1$
• Angle $Q = 55^\circ$

2. Calculate Missing Side RS Using Law of Cosines

We apply the Law of Cosines to find the missing side, $RS$:

$$ RS^2 = QR^2 + QS^2 - 2 \cdot QR \cdot QS \cdot \cos(Q) $$

Substituting the known values:

$$ RS^2 = (33.1)^2 + (39.5)^2 - 2 \cdot (33.1) \cdot (39.5) \cdot \cos(55^\circ) $$

3. Compute the Values

Calculate the right side of the equation:

• ( (33.1)^2 = 1095.61 )
• ( (39.5)^2 = 1560.25 )
• Now calculate ( 2 \cdot (33.1) \cdot (39.5) \cdot \cos(55^\circ) )

Using the cosine value:

$$ \cos(55^\circ) \approx 0.5736 $$

Thus,

$$ 2 \cdot (33.1) \cdot (39.5) \cdot 0.5736 \approx 792.00 $$

Plugging everything back into the equation:

$$ RS^2 = 1095.61 + 1560.25 - 792.00 \approx 1863.86 $$

4. Find Side Length RS

Take the square root:

$$ RS \approx \sqrt{1863.86} \approx 43.2 $$

5. Calculate Remaining Angles Using Law of Sines

With angles and one side known, use the Law of Sines to find angle $R$:

$$ \frac{RS}{\sin(Q)} = \frac{QR}{\sin(R)} $$

Substituting values:

$$ \frac{43.2}{\sin(55^\circ)} = \frac{33.1}{\sin(R)} $$

Calculate $\sin(55^\circ)$:

$$ \sin(55^\circ) \approx 0.8192 $$

Resulting in:

$$ \frac{43.2}{0.8192} \approx 52.7 $$

Now find $R$:

$$ \sin(R) = \frac{33.1}{52.7} \approx 0.628 $$

Thus:

$$ R \approx \sin^{-1}(0.628) \approx 38.77^\circ $$

6. Find Angle S

Finally using the triangle sum property, find angle $S$:

$$ S = 180^\circ - Q - R \approx 180^\circ - 55^\circ - 38.77^\circ \approx 86.23^\circ $$