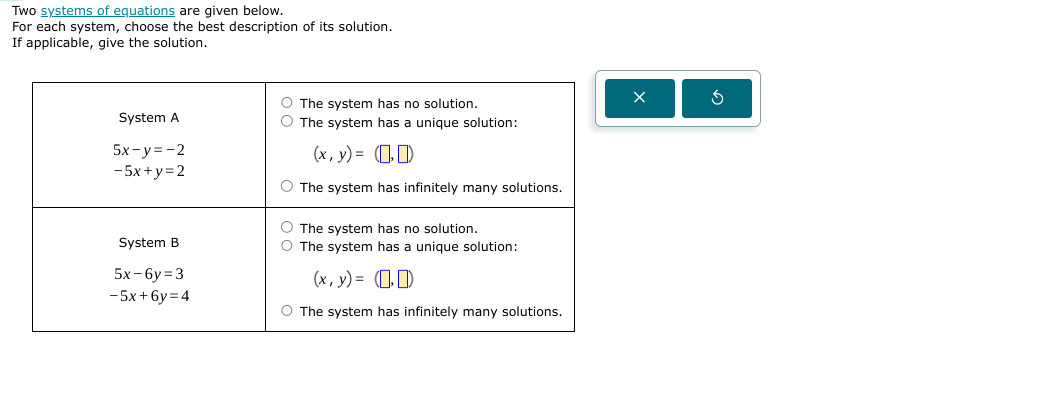
Two systems of equations are given below. For each system, choose the best description of its solution. If applicable, give the solution.
Understand the Problem
The question requires analyzing two systems of equations to determine if they have no solution, a unique solution, or infinitely many solutions, and to provide the solution if applicable.
Answer
- System A: Infinitely many solutions - System B: No solution
Answer for screen readers
- For System A: The system has infinitely many solutions.
- For System B: The system has no solution.
Steps to Solve
- Analyze System A We have the equations: $$ 5x - y = -2 $$ $$ -5x + y = 2 $$
Next, let's add both equations to eliminate $y$: $$ (5x - y) + (-5x + y) = -2 + 2 $$ This simplifies to: $$ 0 = 0 $$
This indicates that the two equations represent the same line, which means there are infinitely many solutions.
- Analyze System B The equations for this system are: $$ 5x - 6y = 3 $$ $$ -5x + 6y = 4 $$
Adding both equations to eliminate $x$ yields: $$ (5x - 6y) + (-5x + 6y) = 3 + 4 $$ This simplifies to: $$ 0 = 7 $$
This is a contradiction, indicating that there is no solution to the system.
- For System A: The system has infinitely many solutions.
- For System B: The system has no solution.
More Information
In System A, the equations are dependent, meaning they represent the same line in a graph, hence, infinitely many solutions exist. In System B, the equations lead to a contradiction, indicating they are parallel lines that never intersect, hence no solutions.
Tips
- Failing to simplify equations correctly can lead to incorrect conclusions about the nature of the systems.
- Confusing dependent equations (infinitely many solutions) with inconsistent ones (no solution) can occur if the analysis of the equations is rushed.
AI-generated content may contain errors. Please verify critical information