The researchers calculated a chi-square value of 29.25. If there are three degrees of freedom, what does this mean?
Understand the Problem
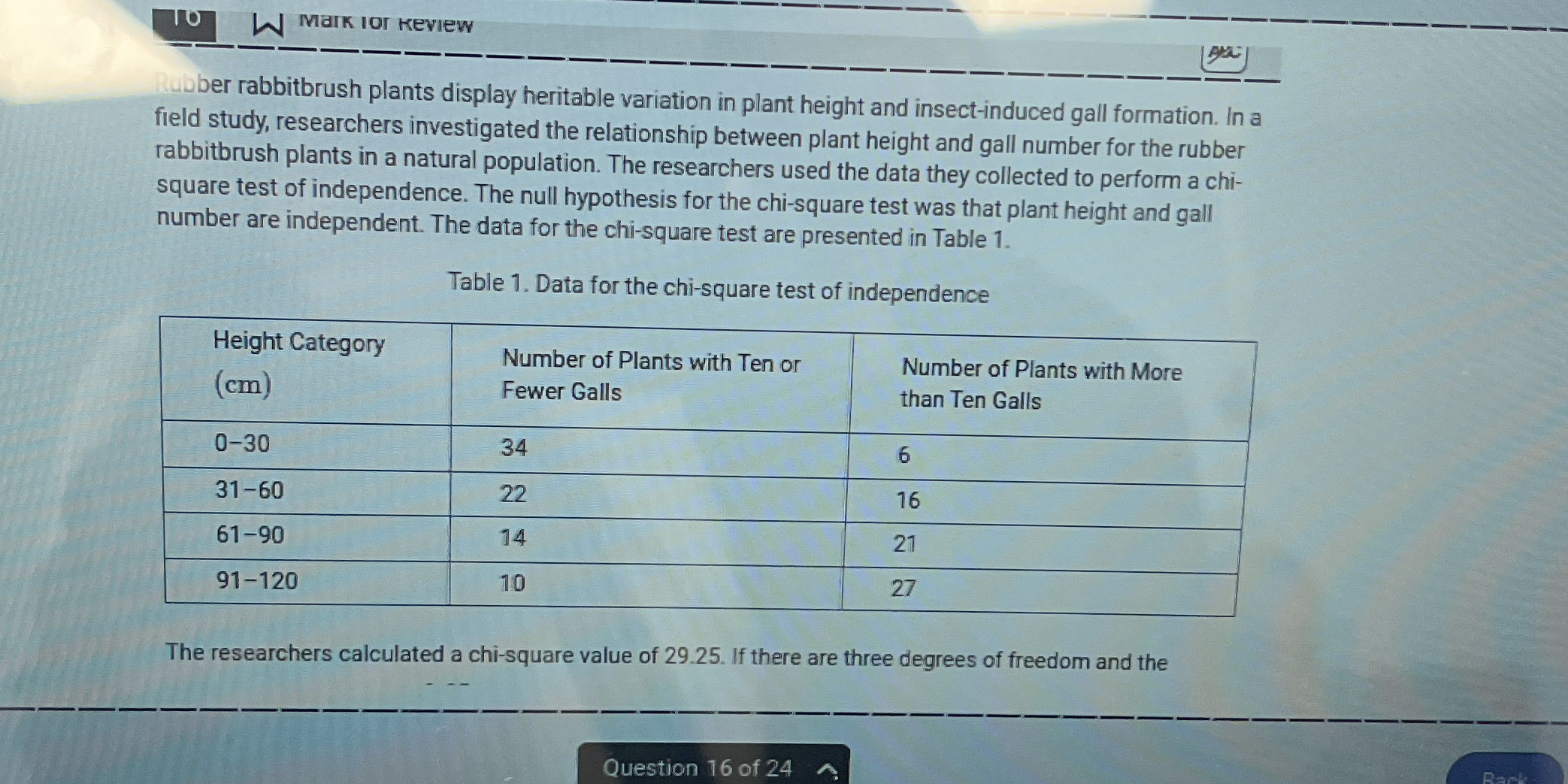
The question is asking about the chi-square test of independence performed on data regarding rubber rabbitbrush plants, specifically to interpret the calculated chi-square value and its significance relative to degrees of freedom.
Answer
The chi-square value of 29.25 is significant, indicating dependence between plant height and gall number.
Answer for screen readers
The calculated chi-square value of 29.25 indicates that there is a significant relationship between plant height and gall number, leading to the rejection of the null hypothesis.
Steps to Solve
-
Understanding the Chi-Square Test The chi-square test of independence checks whether two categorical variables are independent. Here, the variables are plant height and gall number.
-
Set up the Contingency Table Based on the data provided in Table 1, we can summarize it in a contingency table:
Height Category (cm) ≤ 10 Galls > 10 Galls Total 0-30 34 6 40 31-60 22 16 38 61-90 14 21 35 91-120 10 27 37 Total 80 70 150 -
Calculate Expected Frequencies For each cell, the expected frequency is calculated as: $$ E = \frac{(\text{Row Total}) \times (\text{Column Total})}{\text{Grand Total}} $$ For example, for the height category 0-30, galls ≤ 10: $$ E_{0-30, \leq 10} = \frac{40 \times 80}{150} = 21.33 $$
-
Compute the Chi-Square Statistic The chi-square statistic is calculated using: $$ \chi^2 = \sum \frac{(O - E)^2}{E} $$ where ( O ) is the observed frequency and ( E ) is the expected frequency.
-
Degrees of Freedom The degrees of freedom for a chi-square test of independence is given by: $$ df = (r - 1) \times (c - 1) $$ where ( r ) is the number of rows and ( c ) is the number of columns. Here, ( df = (4 - 1)(2 - 1) = 3 ).
-
Interpret the Chi-Square Value A calculated chi-square value of 29.25 with 3 degrees of freedom can be compared to a critical value from the chi-square distribution table for a chosen significance level (e.g., 0.05).
-
Decision on the Null Hypothesis If the calculated chi-square is greater than the critical value, we reject the null hypothesis, indicating that plant height and gall number are dependent.
The calculated chi-square value of 29.25 indicates that there is a significant relationship between plant height and gall number, leading to the rejection of the null hypothesis.
More Information
The significance of the chi-square test is determined by comparing the calculated value to a critical value found in chi-square tables. For 3 degrees of freedom at a 0.05 significance level, the critical value is approximately 7.815. Since 29.25 > 7.815, the result is statistically significant.
Tips
- Misunderstanding Degrees of Freedom: Forgetting to correctly calculate degrees of freedom can lead to incorrect critical value comparisons.
- Incorrect Calculation of Expected Frequencies: Ensure to apply the formula for expected frequencies accurately.
AI-generated content may contain errors. Please verify critical information