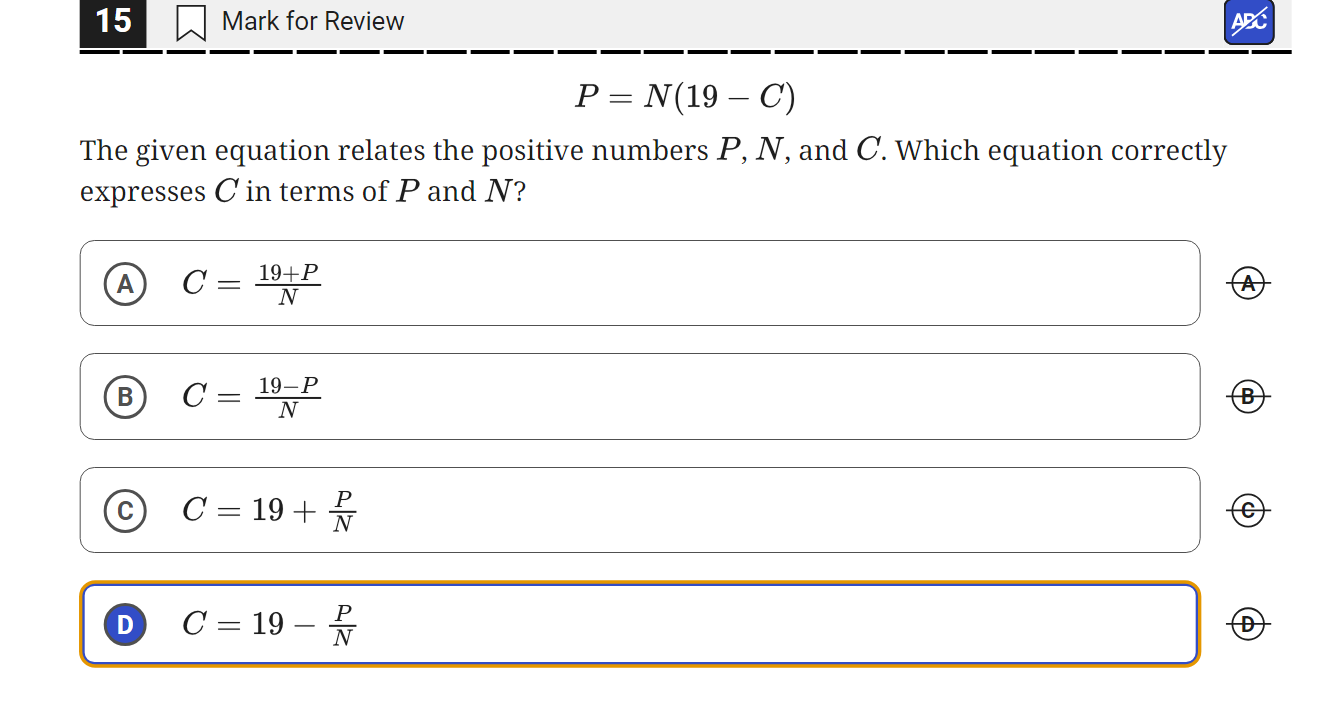
The given equation relates the positive numbers P, N, and C. Which equation correctly expresses C in terms of P and N?
Understand the Problem
The question is asking us to manipulate the given equation P = N(19 - C) to express C in terms of P and N. We need to rearrange the equation to isolate C.
Answer
$$ C = 19 - \frac{P}{N} $$
Answer for screen readers
The equation that expresses (C) in terms of (P) and (N) is
$$ C = 19 - \frac{P}{N} $$
Steps to Solve
- Distribute N across the equation
Start with the given equation:
$$ P = N(19 - C) $$
Distribute (N) to the terms inside the parentheses:
$$ P = 19N - NC $$
- Isolate the NC term
To isolate the term containing (C), move (19N) to the left side:
$$ P - 19N = -NC $$
- Divide by -N
Next, divide both sides by (-N) to solve for (C):
$$ C = \frac{19N - P}{N} $$
Rearranging gives:
$$ C = 19 - \frac{P}{N} $$
- Final expression for C
This can be simplified to the final expression:
$$ C = 19 - \frac{P}{N} $$
The equation that expresses (C) in terms of (P) and (N) is
$$ C = 19 - \frac{P}{N} $$
More Information
This expression shows how (C) varies inversely with (P) when (N) is held constant. Understanding the rearrangement of equations is crucial in algebra for deriving relationships between variables.
Tips
- Incorrect distribution: Failing to properly distribute (N) could lead to an incorrect equation.
- Sign errors: Be careful with negative signs, especially when moving terms across the equation.
- Inappropriate division: Remember to apply division to all parts of the equation when isolating terms.
AI-generated content may contain errors. Please verify critical information