The coordinate of point X on PQ such that PX to XQ is 1:5 is
Understand the Problem
The question is asking for the coordinate of point X on the line segment PQ such that the ratio of the distances PX and XQ is 1:5. This involves finding a point along the line segment based on the specified ratio.
Answer
The coordinate of point X is $-3$.
Answer for screen readers
The coordinate of point X on the line segment PQ such that $PX:XQ = 1:5$ is $-3$.
Steps to Solve
- Identify the coordinates of points P and Q
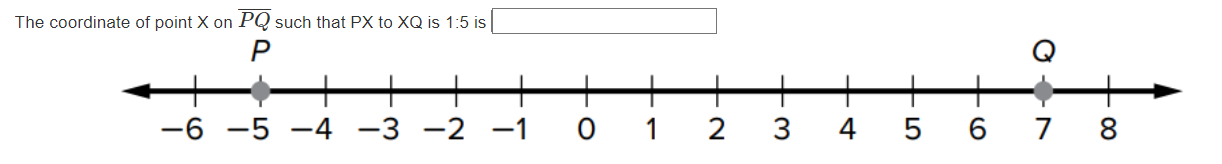
From the diagram, we have:
- The coordinate of point $P$ is $-5$.
- The coordinate of point $Q$ is $7$.
- Determine the total distance between P and Q
The total distance $d$ from $P$ to $Q$ is calculated as follows: $$ d = |Q - P| $$ Substituting the coordinates: $$ d = |7 - (-5)| = |7 + 5| = 12 $$
- Use the ratio to find the distances PX and XQ
Given the ratio of distances $PX:XQ = 1:5$, we can denote:
- Let the distance $PX = x$.
- Then, the distance $XQ = 5x$.
- Therefore, the total distance can be expressed as: $$ x + 5x = 12 $$ This simplifies to: $$ 6x = 12 $$
- Solve for x
Dividing both sides by 6 gives: $$ x = 2 $$
So, the distances are:
- $PX = 2$
- $XQ = 10$
- Calculate the coordinate of point X
Since $X$ is to the right of $P$, we find: $$ X = P + PX = -5 + 2 = -3 $$
The coordinate of point X on the line segment PQ such that $PX:XQ = 1:5$ is $-3$.
More Information
The sectioning of the line based on a ratio helps in understanding proportionate distances on a line. This method can also be applied in various fields like geometry and coordinate systems for similar problems.
Tips
- Mixing up the ratio and incorrectly assigning the distances can lead to wrong calculations. Ensure that the distances correspond correctly to the given ratio.
- Not calculating the total distance correctly can also cause errors. Verify the distance calculation before proceeding.
AI-generated content may contain errors. Please verify critical information