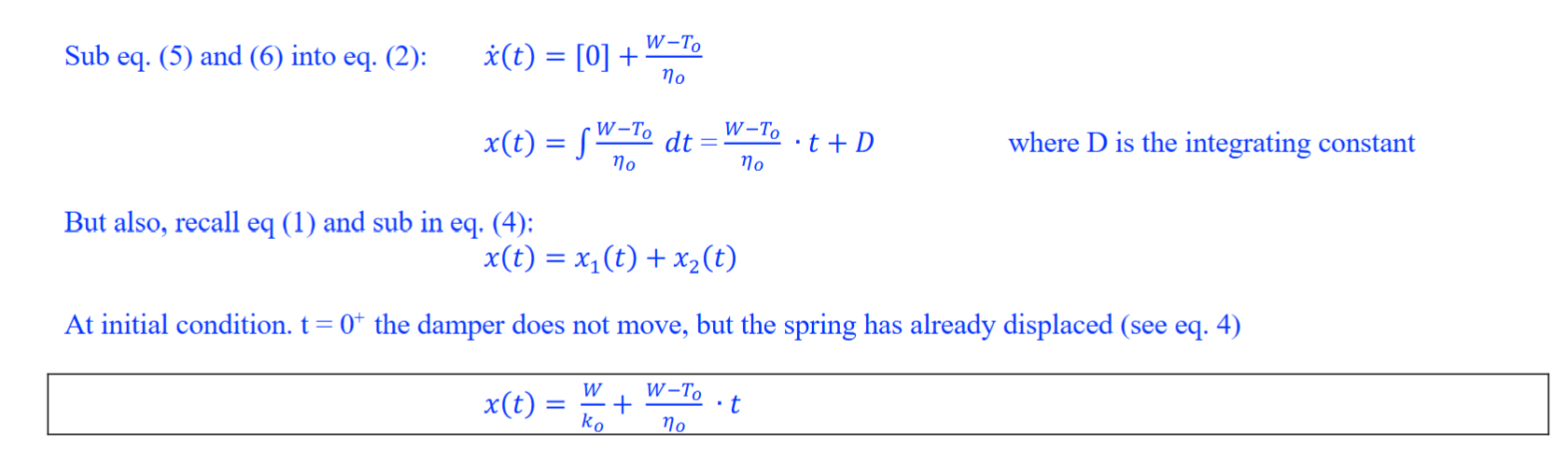
Sub eq. (5) and (6) into eq. (2): ẋ(t) = [0] + W − T₀ / η₀ = ∫ W − T₀ / η₀ dt = (W − T₀ / η₀)·t + D where D is the integrating constant. But also, recall eq (1) and sub in eq (4):... Sub eq. (5) and (6) into eq. (2): ẋ(t) = [0] + W − T₀ / η₀ = ∫ W − T₀ / η₀ dt = (W − T₀ / η₀)·t + D where D is the integrating constant. But also, recall eq (1) and sub in eq (4): x(t) = x₁(t) + x₂(t). At initial condition t = 0+ the damper does not move, but the spring has already displaced. x(t) = W / k₀ + (W − T₀ / η₀)·t.
Understand the Problem
The question appears to be related to engineering or physics, specifically involving the mathematical analysis of a damped spring system. It requires the application of differential equations and integration to derive a function x(t), considering initial conditions and previous equations.
Answer
The displacement is given by \( x(t) = \frac{W}{k_0} + \frac{W - T_0}{\eta_0} \cdot t \).
Answer for screen readers
The function for the displacement ( x(t) ) is given by: $$ x(t) = \frac{W}{k_0} + \frac{W - T_0}{\eta_0} \cdot t $$
Steps to Solve
-
Substituting equations into (2) Substitute equations (5) and (6) into equation (2) to represent $ \dot{x}(t) $.
This forms: $$ \dot{x}(t) = \left[0\right] + \frac{W - T_0}{\eta_0} $$
-
Integrating to find x(t) Integrate the result from the previous step with respect to time $ t $: $$ x(t) = \int \left(\frac{W - T_0}{\eta_0}\right) dt = \frac{W - T_0}{\eta_0} \cdot t + D $$ Here, $ D $ is the integration constant.
-
Combining equations Recall equation (1) and substitute it into equation (4): $$ x(t) = x_1(t) + x_2(t) $$
-
Applying initial conditions At the initial condition where $ t = 0^+ $, the damper does not move but the spring has already displaced. Substitute this condition into the integrated equation: $$ x(0) = \frac{W}{k_0} + \frac{W - T_0}{\eta_0} \cdot 0 = \frac{W}{k_0} $$
-
Final expression Hence, the full expression for $ x(t) $ at time $ t $ is: $$ x(t) = \frac{W}{k_0} + \frac{W - T_0}{\eta_0} \cdot t $$
The function for the displacement ( x(t) ) is given by: $$ x(t) = \frac{W}{k_0} + \frac{W - T_0}{\eta_0} \cdot t $$
More Information
This equation models the displacement in a damped spring system, where:
- ( W ) is the weight.
- ( T_0 ) is the initial tension.
- ( \eta_0 ) is the damping coefficient.
- ( k_0 ) is the spring constant. This function combines the initial displacement of the spring with the effect of damping over time.
Tips
- Misunderstanding when to apply initial conditions. Ensure that you clearly outline the conditions before substituting into equations.
- Forgetting to include the integration constant ( D ) during integration, which can lead to incomplete solutions.
AI-generated content may contain errors. Please verify critical information