Solve using substitution. -5x + 8y = -1; x + y = 8.
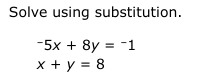
Understand the Problem
The question is asking to solve a system of equations using the substitution method. We will isolate one variable in one equation and substitute it into the other equation.
Answer
The solution is \(x = 5\) and \(y = 3\).
Answer for screen readers
The solution to the system of equations is (x = 5) and (y = 3).
Steps to Solve
-
Isolate one variable from one equation Start by isolating (y) in the second equation (x + y = 8). [ y = 8 - x ]
-
Substitute the isolated variable into the first equation Next, substitute (y) in the first equation (-5x + 8y = -1) using the expression we found in the first step. [ -5x + 8(8 - x) = -1 ]
-
Simplify the equation Distribute and simplify the equation. [ -5x + 64 - 8x = -1 ] Combine like terms: [ -13x + 64 = -1 ]
-
Solve for (x) Isolate (x) by moving 64 to the other side. [ -13x = -1 - 64 ] [ -13x = -65 ] Divide by -13: [ x = 5 ]
-
Substitute (x) back to find (y) Now that we have (x), substitute (x = 5) back into the equation (y = 8 - x). [ y = 8 - 5 ] [ y = 3 ]
The solution to the system of equations is (x = 5) and (y = 3).
More Information
The method of substitution is useful for solving systems of equations, especially when one equation is easy to manipulate. Here, isolating (y) made it straightforward to substitute into the first equation.
Tips
- Forgetting to distribute correctly: When substituting, be sure to multiply correctly.
- Mismanaging signs: Pay attention to positive and negative signs when moving terms from one side of the equation to the other.
AI-generated content may contain errors. Please verify critical information