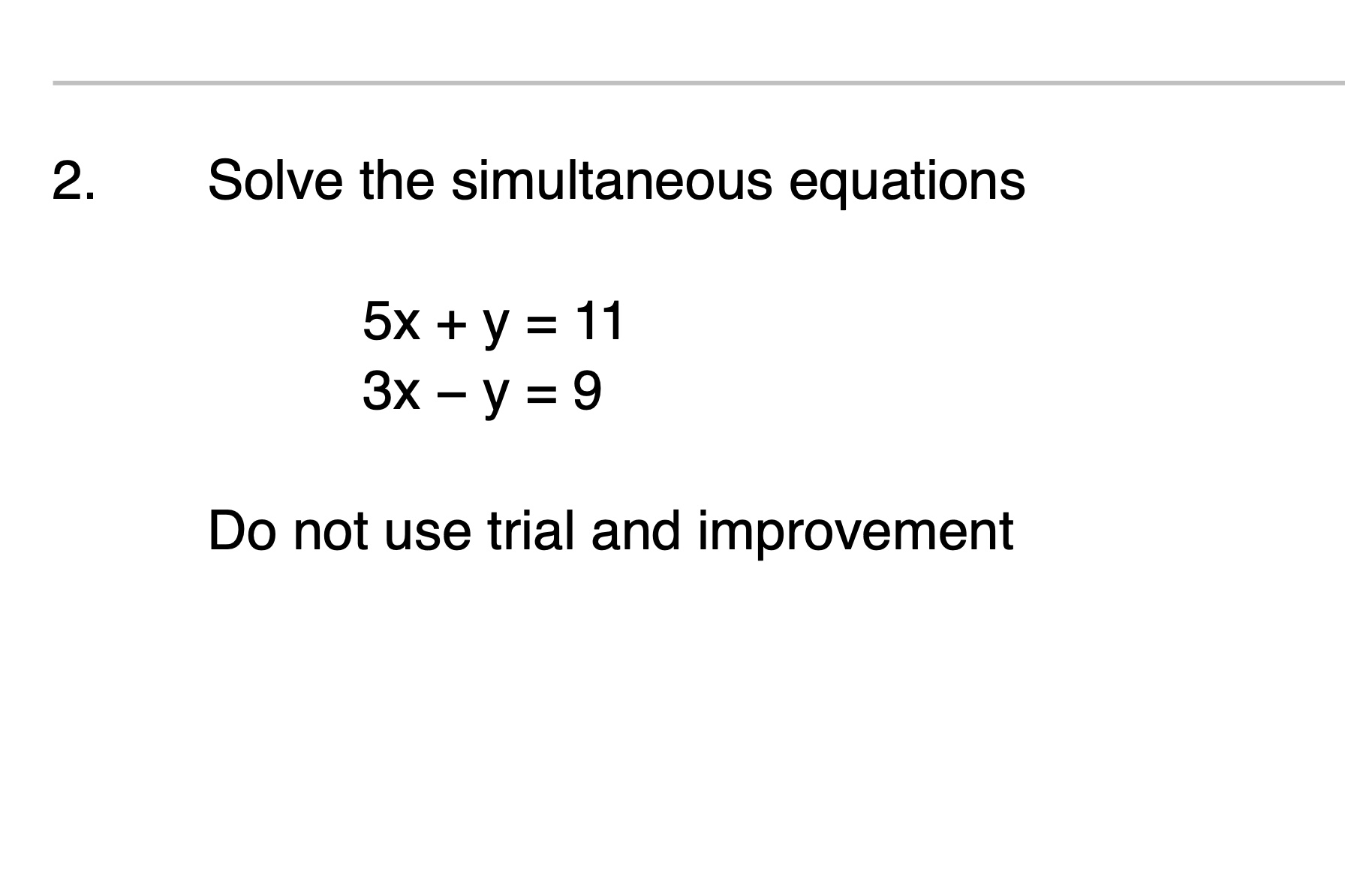
Solve the simultaneous equations 5x + y = 11 and 3x - y = 9. Do not use trial and improvement.
Understand the Problem
The question is asking to solve two simultaneous equations: 5x + y = 11 and 3x - y = 9, without using trial and error methods. A systematic approach such as substitution or elimination is required.
Answer
The solution is \(x = 2.5\) and \(y = -1.5\).
Answer for screen readers
The solution to the simultaneous equations is: [ x = 2.5, \quad y = -1.5 ]
Steps to Solve
-
Choose a Method We will use the elimination method to solve the simultaneous equations. The equations are: [ 5x + y = 11 \quad (1) ] [ 3x - y = 9 \quad (2) ]
-
Add the Equations To eliminate (y), we can add equations (1) and (2) together. First, rearrange equation (2) to match the format of equation (1): [ 3x - y + y = 9 + y ] This results in: [ 3x = 9 + y ]
Now, we can add the two equations together: [ (5x + y) + (3x - y) = 11 + 9 ] This simplifies to: [ 5x + 3x = 20 ]
-
Combine Like Terms Now, combine the like terms: [ 8x = 20 ]
-
Solve for (x) To find (x), divide both sides by 8: [ x = \frac{20}{8} = 2.5 ]
-
Substitute (x) Back Now, substitute (x = 2.5) back into one of the original equations. We'll use equation (1): [ 5(2.5) + y = 11 ]
Calculating (5 \times 2.5) gives: [ 12.5 + y = 11 ]
- Isolate (y) Now isolate (y): [ y = 11 - 12.5 = -1.5 ]
The solution to the simultaneous equations is: [ x = 2.5, \quad y = -1.5 ]
More Information
The solution indicates that the two lines represented by the equations intersect at the point ((2.5, -1.5)).
Tips
- Misaligning terms when adding equations, leading to incorrect coefficients.
- Forgetting to reverse the signs when rearranging the second equation can lead to errors.
- Not plugging the calculated value of (x) back into the correct original equation.
AI-generated content may contain errors. Please verify critical information