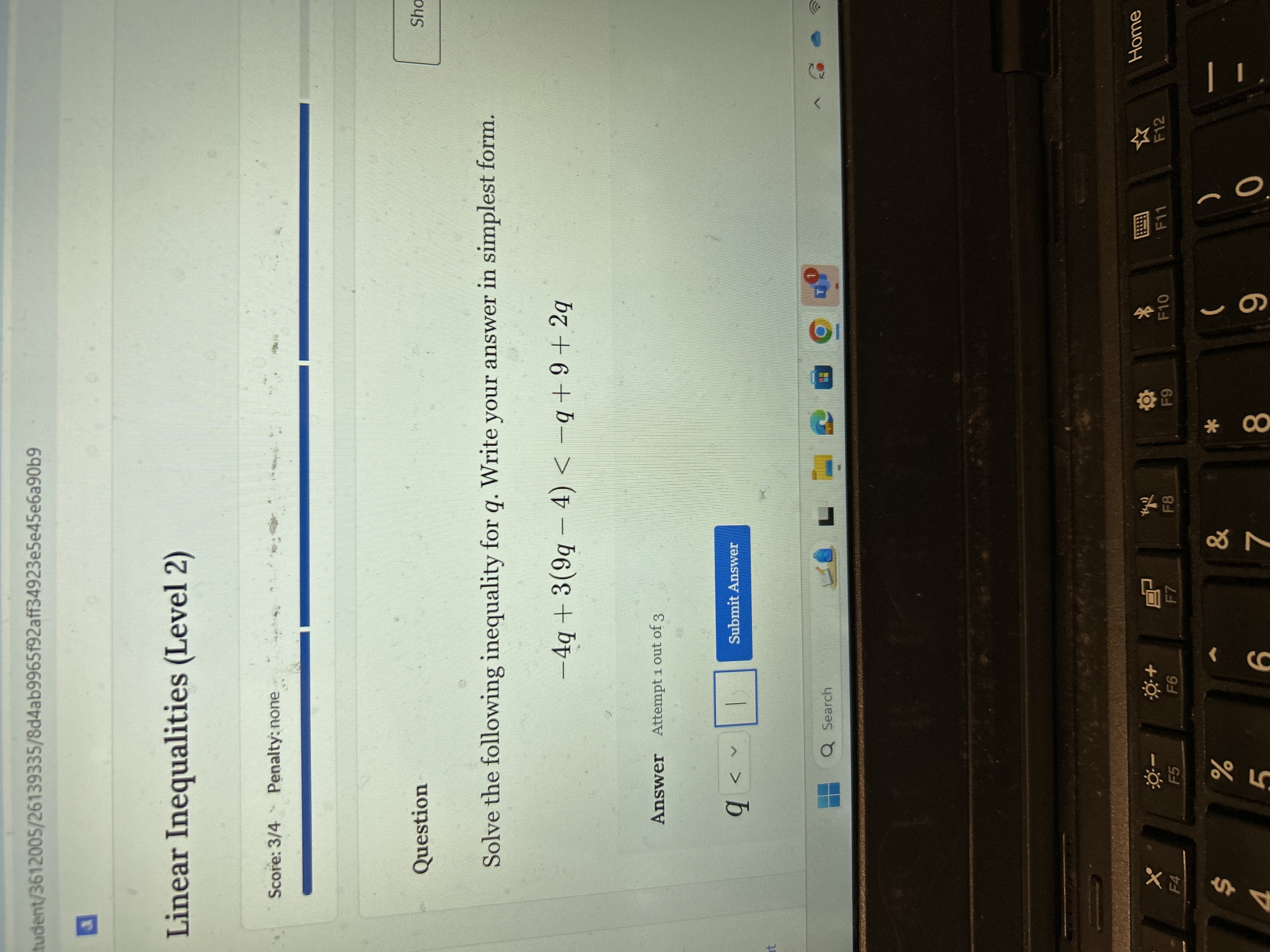
Solve the following inequality for q. Write your answer in simplest form: -4q + 3(9q - 4) < -q + 9 + 2q
Understand the Problem
The question is asking to solve the inequality for the variable q, and to provide the answer in its simplest form. This involves rearranging the terms in the inequality and isolating q to find its possible values.
Answer
$$ q > -10.5 $$
Answer for screen readers
The solution to the inequality is
$$ q > -10.5 $$.
Steps to Solve
- Start with the original inequality
The given inequality is
$$ -4q + 3(q - 4) < -q + 9 + 2q $$.
- Distribute terms on the left side
Distribute the $3$ into $(q - 4)$:
$$ -4q + 3q - 12 < -q + 9 + 2q $$.
- Combine like terms on both sides
On the left side, combine $-4q$ and $3q$, and on the right side combine $2q$ and $-q$:
$$ -q - 12 < (9 + q) $$.
- Isolate the variable $q$
Move $q$ to the left side and $-12$ to the right side:
$$ -q - q < 9 + 12 $$
This simplifies to:
$$ -2q < 21 $$.
- Divide by -2 and flip the inequality sign
When dividing by a negative number, the inequality must be flipped:
$$ q > -\frac{21}{2} $$.
- Simplify the fraction
Convert it to a mixed number if needed:
$$ q > -10.5 $$.
The solution to the inequality is
$$ q > -10.5 $$.
More Information
This inequality means that $q$ can take any value greater than $-10.5$. The process of solving the inequality involves distributing, combining like terms, and isolating the variable while being mindful of flipping the inequality sign when dividing by a negative number.
Tips
- Not flipping the inequality sign: When dividing by a negative number, always remember to reverse the inequality direction.
- Incorrect distribution: Ensure that you apply the distributive property correctly when multiplying terms.
- Combining unlike terms: Be cautious while combining like terms to avoid mistakes.
AI-generated content may contain errors. Please verify critical information