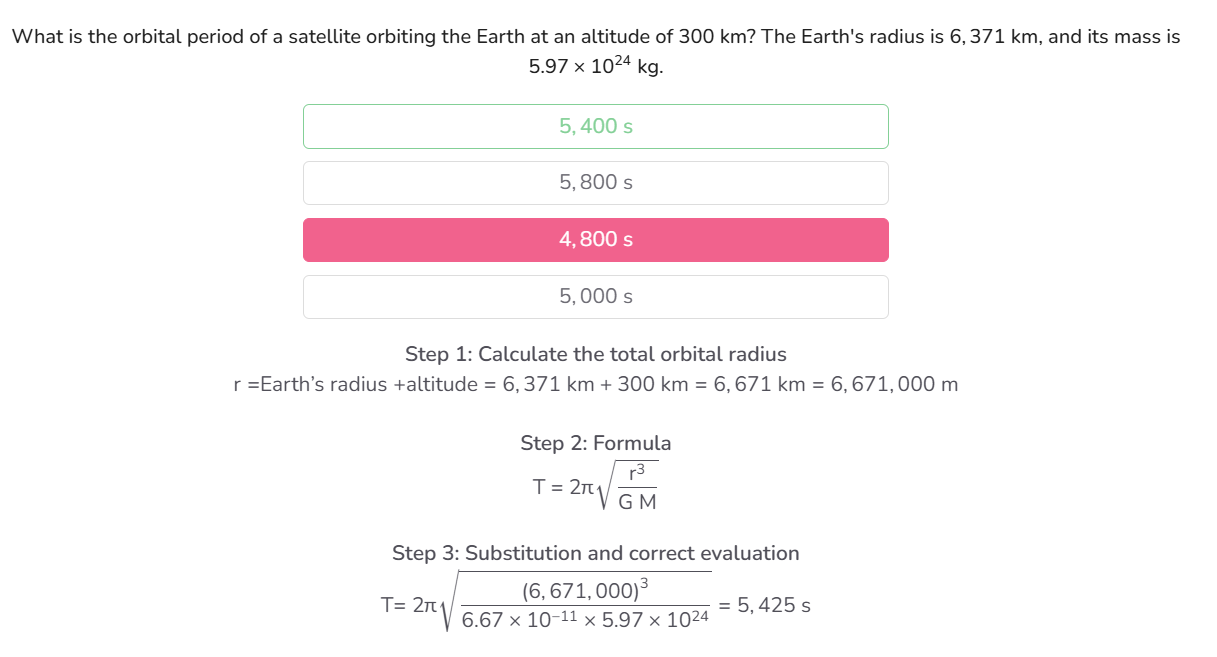
What is the orbital period of a satellite orbiting the Earth at an altitude of 300 km? The Earth's radius is 6,371 km, and its mass is 5.97 x 10^24 kg.

Steps to Solve

1. Calculate the total orbital radius

To find the total orbital radius of the satellite, we add the Earth's radius to the altitude: $$ r = \text{Earth's radius} + \text{altitude} = 6371 \text{ km} + 300 \text{ km} = 6671 \text{ km} = 6,671,000 \text{ m} $$

2. Write the formula for orbital period

The formula for the orbital period ( T ) of a satellite is given by: $$ T = 2\pi \sqrt{\frac{r^3}{GM}} $$ where:

• ( G = 6.67 \times 10^{-11} \text{ m}^3/\text{kg s}^2 ) (gravitational constant)
• ( M = 5.97 \times 10^{24} \text{ kg} ) (mass of the Earth)

3. Substitute the values into the formula and calculate

Substitute the values of ( r ), ( G ), and ( M ) into the formula: $$ T = 2\pi \sqrt{\frac{(6,671,000)^3}{6.67 \times 10^{-11} \times 5.97 \times 10^{24}}} $$

Now calculate the value:

1. Calculate ( (6,671,000)^3 )
2. Calculate ( 6.67 \times 10^{-11} \times 5.97 \times 10^{24} )
3. Divide the first result by the second
4. Take the square root
5. Finally, multiply by ( 2\pi )

Performing these calculations gives: $$ T \approx 5,425 \text{ s} $$