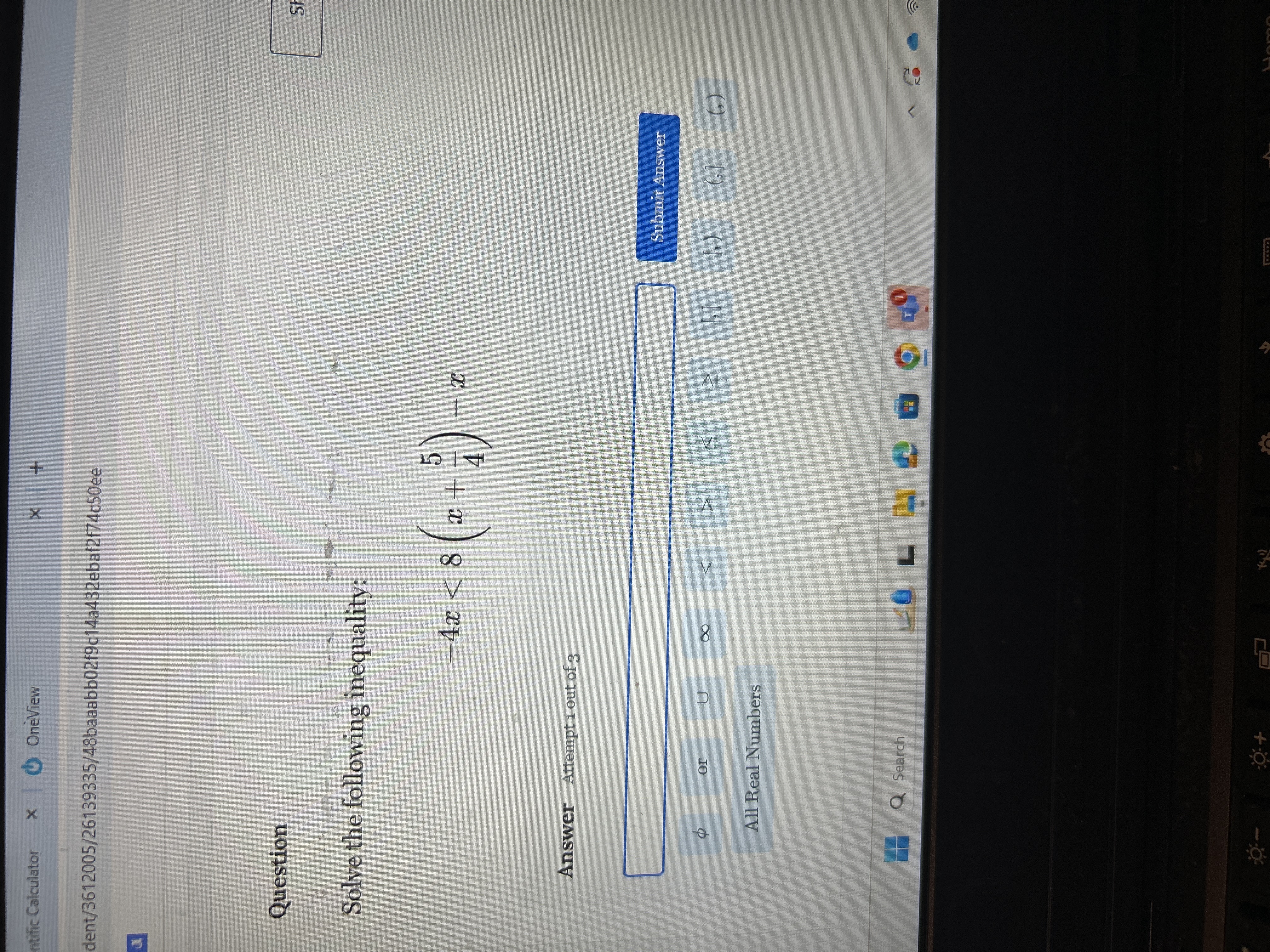
Solve the following inequality: -4x < 8(x + 5/4) - x
Understand the Problem
The question is asking to solve an inequality involving the variable x. It requires applying algebraic methods to isolate x and determine the range of values that satisfy the inequality.
Answer
The solution to the inequality is \( x > -\frac{10}{11} \).
Answer for screen readers
The solution to the inequality is ( x > -\frac{10}{11} ).
Steps to Solve
-
Rewrite the Inequality
Start with the given inequality: $$ -4x < 8\left(x + \frac{5}{4}\right) - x $$
-
Simplify the Right Side
Distribute the 8: $$ -4x < 8x + 10 - x $$
Combine like terms: $$ -4x < 7x + 10 $$
-
Isolate x
Add $4x$ to both sides: $$ 0 < 11x + 10 $$
Subtract 10 from both sides: $$ -10 < 11x $$
-
Divide by 11
Divide both sides by 11 to isolate $x$: $$ \frac{-10}{11} < x $$
This can also be written as: $$ x > -\frac{10}{11} $$
-
State the Solution Set
The solution for the inequality is: $$ x > -\frac{10}{11} $$
The solution to the inequality is ( x > -\frac{10}{11} ).
More Information
This solution means that any value greater than (-\frac{10}{11}) will satisfy the original inequality. This can be represented on a number line where everything to the right of (-\frac{10}{11}) is included in the solution set.
Tips
- Misapplying Distribution: Ensure to distribute correctly when multiplying terms, especially with negatives.
- Incorrectly Combining Like Terms: Double-check the addition and subtraction of terms to avoid errors.
- Dividing with Negatives: Remember that dividing by a negative flips the inequality sign.
AI-generated content may contain errors. Please verify critical information