Solve the equations: 3(2x - 14) - 3x = 13(x - 4) and 3 = -9(x - 5) + 15x.
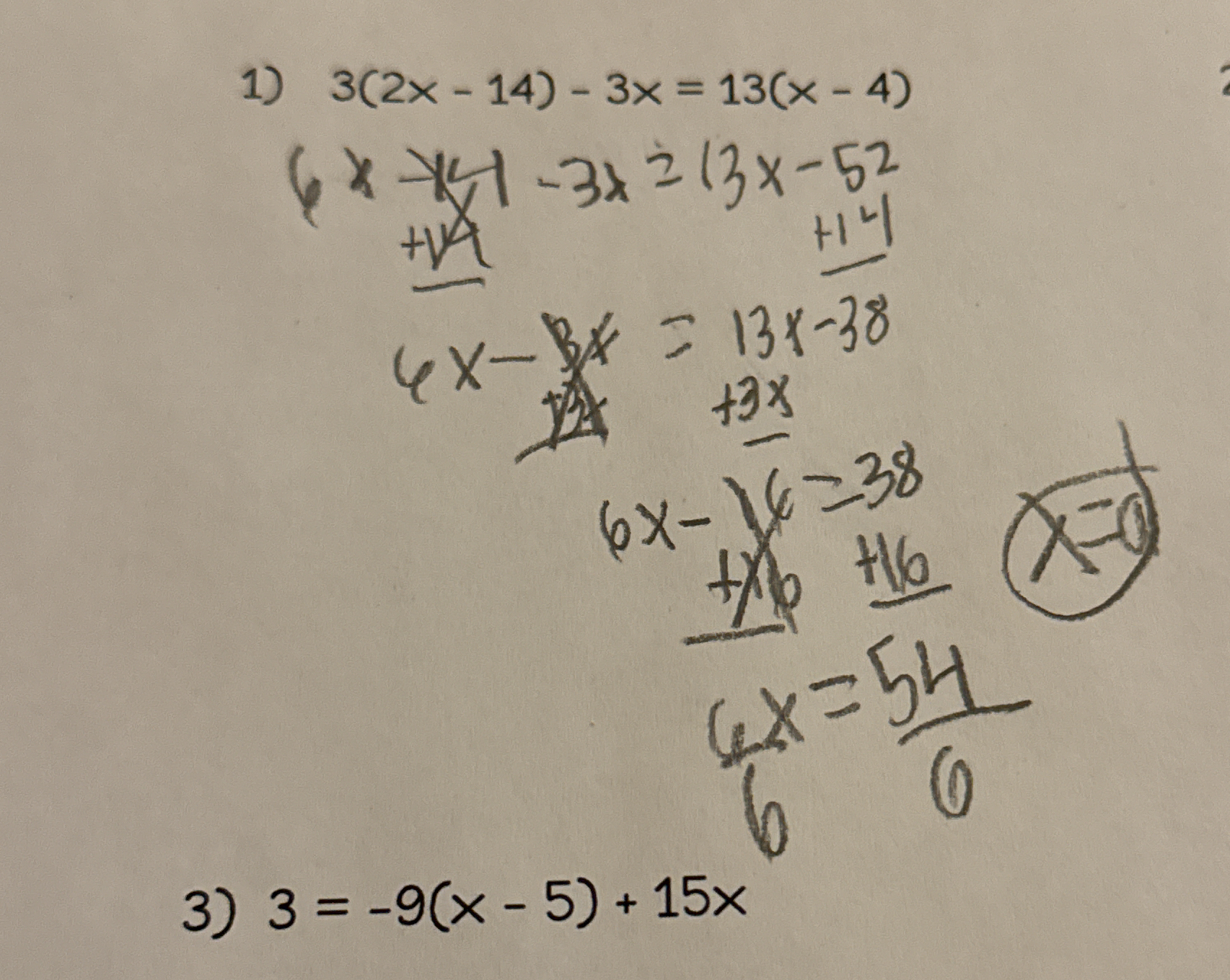
Understand the Problem
The question involves solving two linear equations for the variable x. It displays calculations leading to the solutions for each equation.
Answer
$x = -7$
Answer for screen readers
The solution for $x$ is
$$ x = -7 $$
Steps to Solve
- Expand the equations
Begin by expanding both sides of the equation for step 3:
$$ 3 = -9(x - 5) + 15x $$
Expanding the left side, we get:
$$ 3 = -9x + 45 + 15x $$
- Combine like terms
Next, combine like terms on the right side:
$$ 3 = (-9x + 15x) + 45 $$
This simplifies to:
$$ 3 = 6x + 45 $$
- Isolate the variable
Now, isolate $x$ by subtracting 45 from both sides:
$$ 3 - 45 = 6x $$
This results in:
$$ -42 = 6x $$
- Solve for x
Finally, divide by 6 to find the value of $x$:
$$ x = \frac{-42}{6} = -7 $$
The solution for $x$ is
$$ x = -7 $$
More Information
This problem involves solving a linear equation by applying the distributive property, combining like terms, and isolating the variable. It demonstrates fundamental algebra techniques that are essential in solving equations.
Tips
- Forgetting to distribute negative signs when expanding terms.
- Miscombining like terms or forgetting to combine all instances of a variable.
- Failing to properly isolate the variable, leading to incorrect division or manipulation.
AI-generated content may contain errors. Please verify critical information