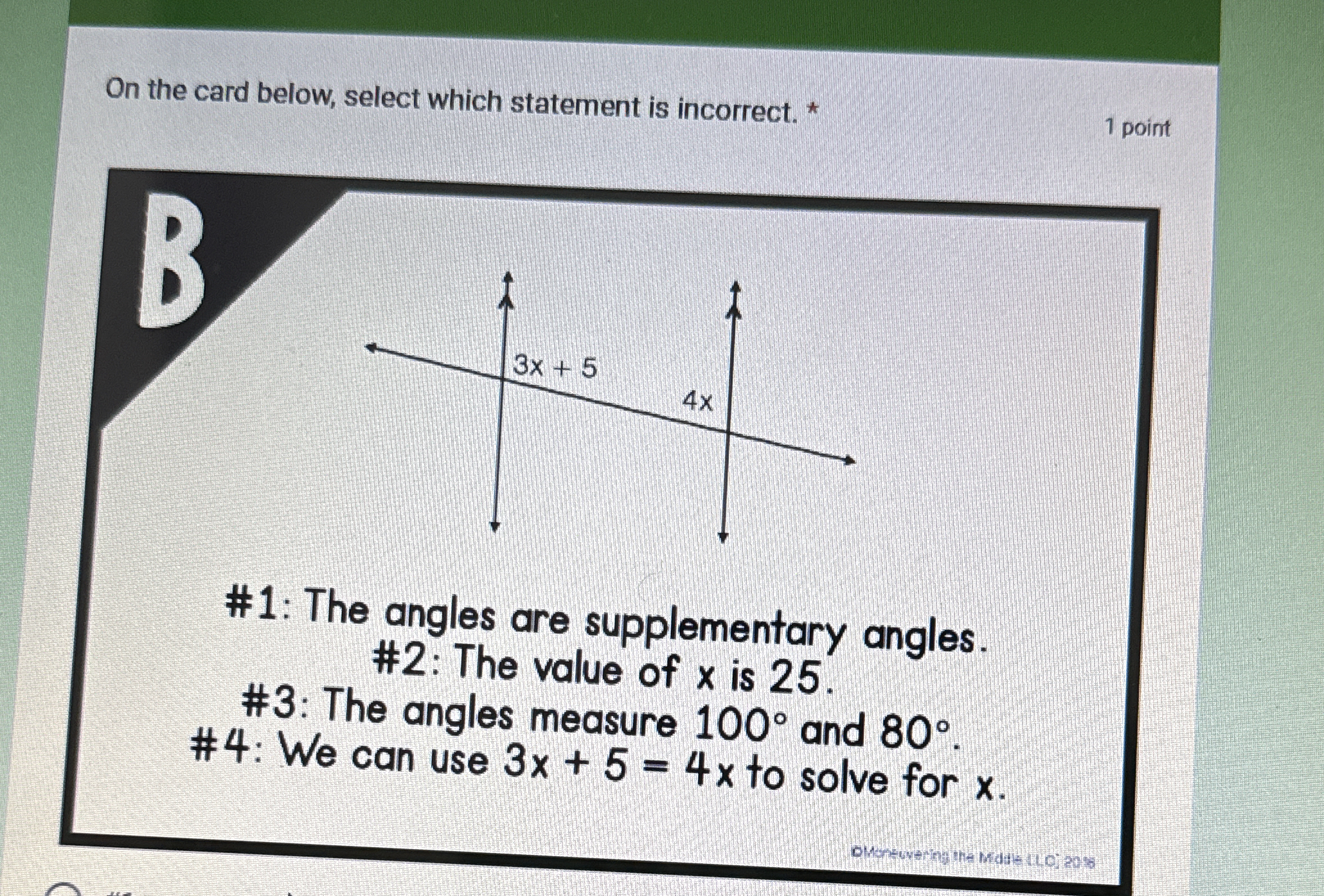
On the card below, select which statement is incorrect: #1: The angles are supplementary angles. #2: The value of x is 25. #3: The angles measure 100° and 80°. #4: We can use 3x +... On the card below, select which statement is incorrect: #1: The angles are supplementary angles. #2: The value of x is 25. #3: The angles measure 100° and 80°. #4: We can use 3x + 5 = 4x to solve for x.
Understand the Problem
The question is asking to identify which of the given statements regarding the angles formed by the intersecting lines is incorrect.
Answer
#2: The value of $x$ is 25.
Answer for screen readers
The incorrect statement is #2: The value of $x$ is 25.
Steps to Solve
-
Identify the relationship between angles The angles formed by intersecting lines can be either supplementary or complementary. Supplementary angles add up to $180^\circ$.
-
Check the given angles against their measures Given that the angles measure $100^\circ$ and $80^\circ$, we can check if they are supplementary: $$ 100^\circ + 80^\circ = 180^\circ $$
-
Evaluate each statement
- Statement #1: The angles are supplementary angles. (True)
- Statement #2: The value of $x$ is $25$. We will verify this next.
- Statement #3: The angles measure $100^\circ$ and $80^\circ$. (True)
- Statement #4: We can use $3x + 5 = 4x$ to solve for $x$.
-
Solve for x using the equation To solve $3x + 5 = 4x$, we rearrange the equation: Subtract $3x$ from both sides: $$ 5 = 4x - 3x $$ Then: $$ 5 = x $$ Thus, $x = 5$.
-
Evaluate Statement #2 Since we calculated $x$ to be $5$ and not $25$, Statement #2 is incorrect.
The incorrect statement is #2: The value of $x$ is 25.
More Information
The angles formed by intersecting lines are either supplementary, where their measures equal $180^\circ$, or vertical angles that are equal in measure. In this case, knowing one angle allows us to easily find information about the other.
Tips
- Confusing supplementary and complementary angles. Remember, supplementary angles add up to $180^\circ$ while complementary angles add up to $90^\circ$.
- Miscalculating the equation when solving for $x$. Always ensure each step follows logically and check your work.
AI-generated content may contain errors. Please verify critical information