Solve the equation x² + 4x = 5.
Understand the Problem
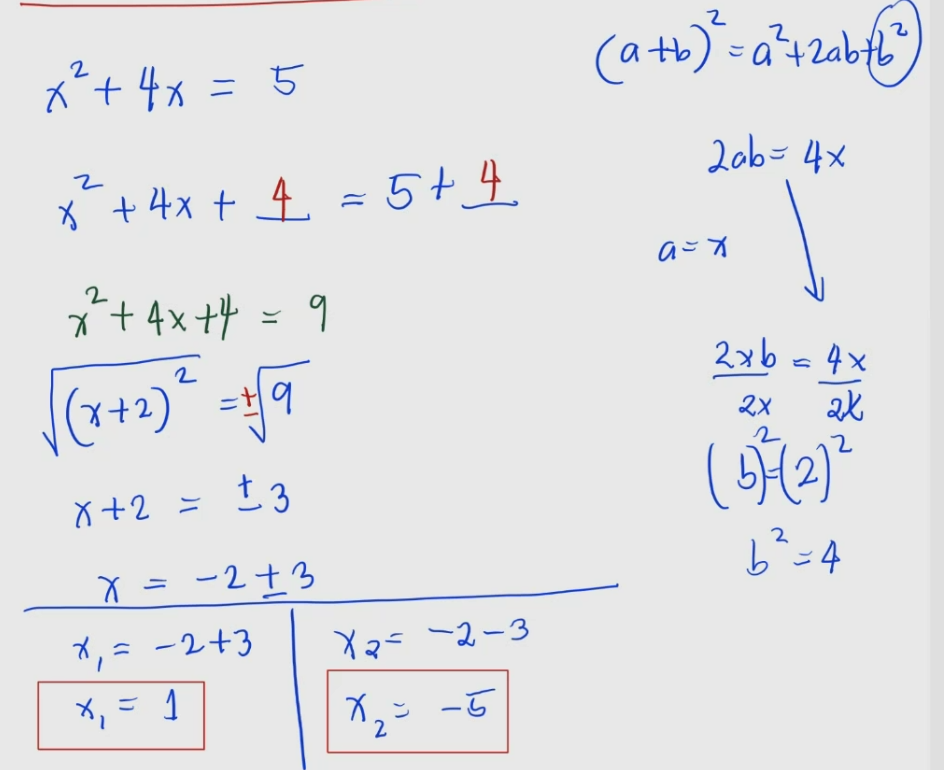
The question involves solving a quadratic equation of the form x² + 4x = 5, which is rewritten, manipulated, and solved step by step. The solution process includes completing the square and finding the roots of the quadratic equation.
Answer
The solutions are $x_1 = 1$ and $x_2 = -5$.
Answer for screen readers
The solutions to the equation $x^2 + 4x = 5$ are:
$$ x_1 = 1 \quad \text{and} \quad x_2 = -5 $$
Steps to Solve
- Rearranging the equation
Start by moving all terms to one side:
$$ x^2 + 4x - 5 = 0 $$
- Completing the square
To complete the square, add and subtract the square of half the coefficient of $x$. Here, take half of $4$ (which is $2$) and square it ($2^2 = 4$):
$$ x^2 + 4x + 4 - 4 - 5 = 0 $$
This simplifies to:
$$ (x + 2)^2 - 9 = 0 $$
- Isolating the square
Move $-9$ to the other side:
$$ (x + 2)^2 = 9 $$
- Taking the square root
Take the square root of both sides:
$$ x + 2 = \pm 3 $$
- Solving for $x$
Now solve for $x$ by isolating it:
- For the positive case:
$$ x + 2 = 3 \implies x = 3 - 2 = 1 $$
- For the negative case:
$$ x + 2 = -3 \implies x = -3 - 2 = -5 $$
Thus, the solutions are:
$$ x_1 = 1 \quad \text{and} \quad x_2 = -5 $$
The solutions to the equation $x^2 + 4x = 5$ are:
$$ x_1 = 1 \quad \text{and} \quad x_2 = -5 $$
More Information
This equation is a typical example of a quadratic equation where completing the square is used to find its roots. Quadratic equations often have two distinct solutions, and this method allows us to determine them with clarity.
Tips
- Failing to correctly complete the square by miscalculating the squared term.
- Forgetting to switch the equation to zero by moving all terms to one side first.
- Not considering both the positive and negative roots when taking the square root.
AI-generated content may contain errors. Please verify critical information