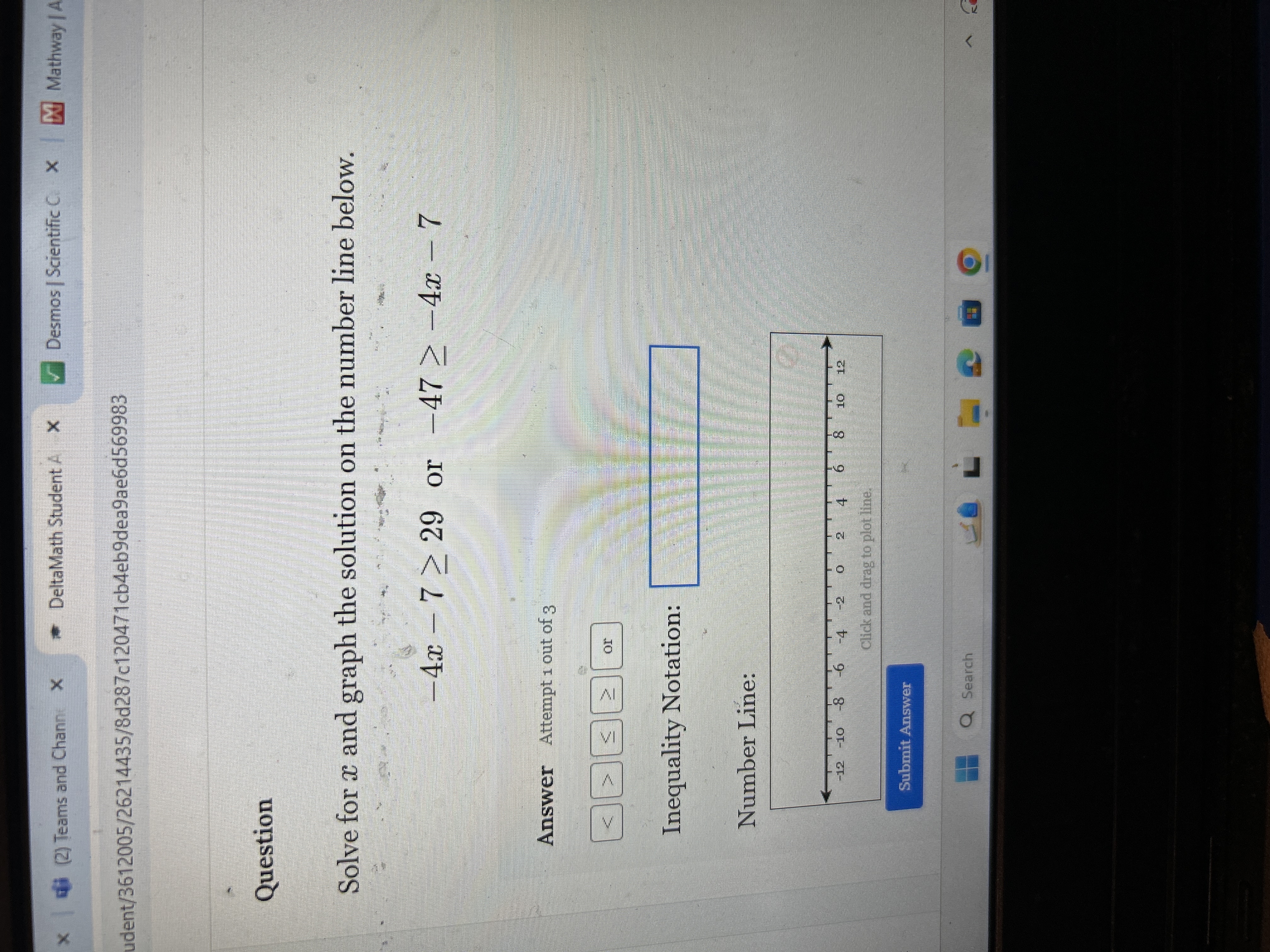
Solve for x and graph the solution on the number line below. -4x - 7 ≥ 29 or -47 ≥ -4x - 7
Understand the Problem
The question is asking to solve two inequalities for x and then graph the solution on a number line. This requires finding the values of x that satisfy at least one of the inequalities provided.
Answer
The solution is \( x \leq -9 \ \text{or} \ x \geq 10 \).
Answer for screen readers
The solution in inequality notation is: $$ x \leq -9 \ \text{or} \ x \geq 10 $$
Steps to Solve
- Solve the first inequality
Start with the first inequality: $$ -4x - 7 \geq 29 $$
Add 7 to both sides: $$ -4x \geq 29 + 7 $$ $$ -4x \geq 36 $$
Now, divide by -4 (remember to flip the inequality sign when dividing by a negative number): $$ x \leq -9 $$
- Solve the second inequality
Now, let's solve the second inequality: $$ -47 \geq -4x - 7 $$
Add 7 to both sides: $$ -47 + 7 \geq -4x $$ $$ -40 \geq -4x $$
Again, divide by -4 (and flip the inequality sign): $$ 10 \leq x $$ or equivalently, $$ x \geq 10 $$
- Combine the solutions
Now we have the solutions from both inequalities:
- From the first: ( x \leq -9 )
- From the second: ( x \geq 10 )
Because it’s an "or" statement, the solution includes all values less than or equal to -9, and all values greater than or equal to 10.
The solution in inequality notation is: $$ x \leq -9 \ \text{or} \ x \geq 10 $$
More Information
These inequalities represent two separate ranges of values for ( x ): one is all numbers less than or equal to -9, and the other is all numbers greater than or equal to 10. This kind of solution can be common in problems involving "or" inequalities.
Tips
- Forgetting to flip the inequality sign: When dividing or multiplying by a negative number, remember to flip the inequality sign.
- Misinterpreting "or": Ensure that both solutions are represented correctly on the number line, as they are separate ranges.
AI-generated content may contain errors. Please verify critical information