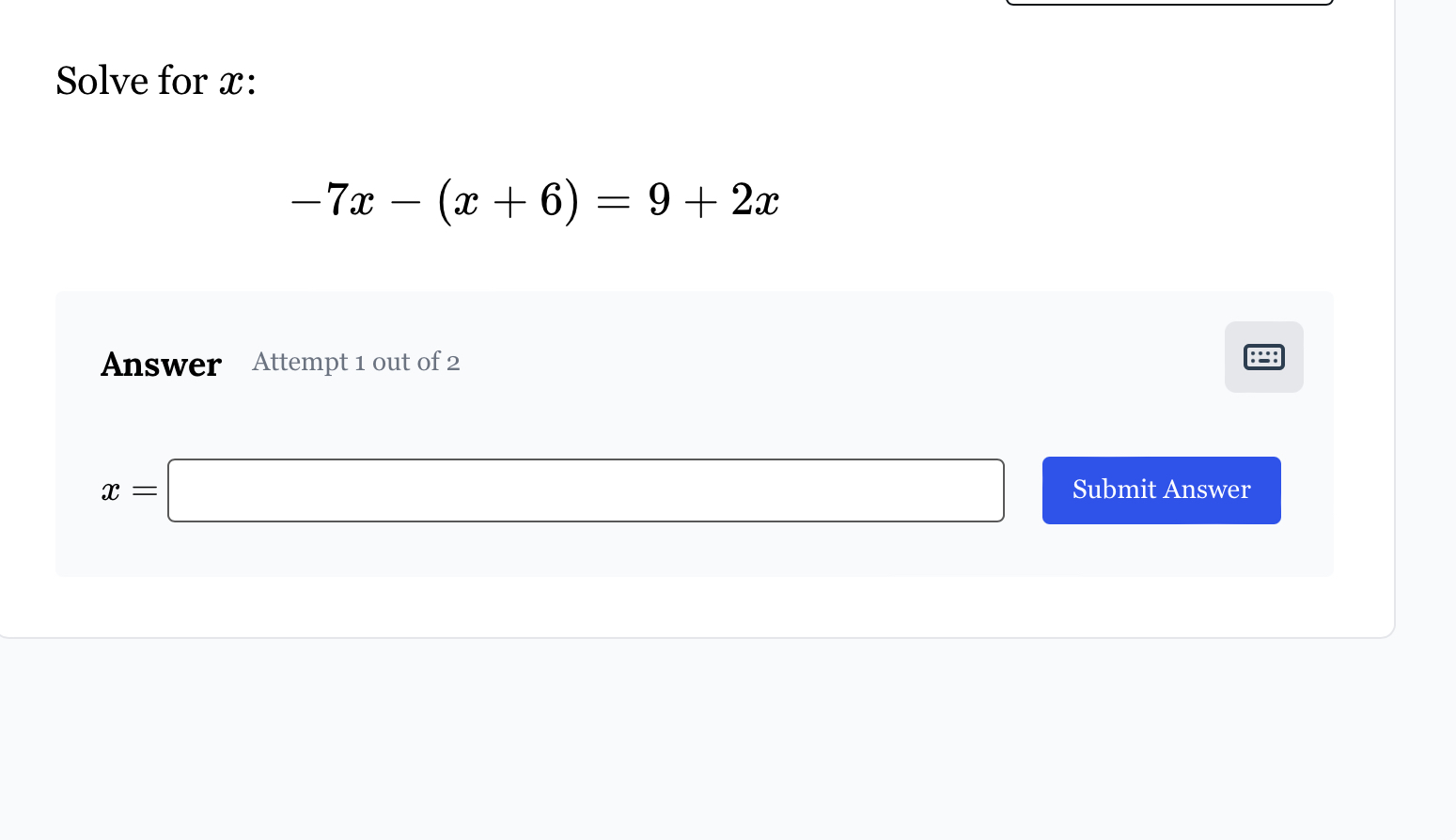
Solve for x: -7x - (x + 6) = 9 + 2x
Understand the Problem
The question is asking to solve the equation for the variable x. The equation provided involves simplifying and rearranging the terms to isolate x on one side.
Answer
$$ x = -\frac{3}{2} $$
Answer for screen readers
$$ x = -\frac{3}{2} $$
Steps to Solve
- Distribute the Negative Sign
Distribute the negative sign across the parentheses on the left-hand side:
$$ -7x - (x + 6) = -7x - x - 6 $$
This simplifies the equation to: $$ -7x - x - 6 = 9 + 2x $$
- Combine Like Terms on the Left Side
Combine the terms involving $x$ on the left-hand side:
$$ -8x - 6 = 9 + 2x $$
- Add 6 to Both Sides
Add 6 to both sides to isolate the $x$ terms:
$$ -8x - 6 + 6 = 9 + 6 + 2x $$
Which simplifies to:
$$ -8x = 15 + 2x $$
- Move $2x$ to the Left Side
Subtract $2x$ from both sides:
$$ -8x - 2x = 15 $$
This gives:
$$ -10x = 15 $$
- Isolate $x$
Divide both sides by $-10$ to solve for $x$:
$$ x = \frac{15}{-10} $$
Which simplifies to:
$$ x = -\frac{3}{2} $$
$$ x = -\frac{3}{2} $$
More Information
The solution $x = -\frac{3}{2}$ indicates that if we substitute $x$ back into the original equation, both sides will be equal. This solution involves rearranging and simplifying terms using basic algebraic principles.
Tips
- Forgetting to Distribute: A common mistake is neglecting to distribute the negative sign across the parentheses properly. Always ensure to apply this step correctly.
- Combining Incorrect Terms: Be cautious while combining like terms; mixing constants with variable terms can lead to incorrect results.
- Sign Errors: Watch for sign changes especially when moving terms from one side of the equation to the other.
AI-generated content may contain errors. Please verify critical information