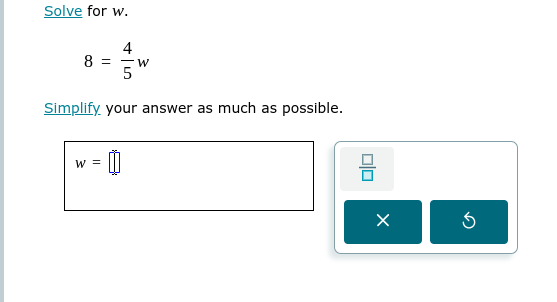
Solve for w in the equation 8 = (4/5)w. Simplify your answer as much as possible.
Understand the Problem
The question is asking to solve the equation 8 = (4/5)w for the variable w and to simplify the answer as much as possible.
Answer
$w = 10$
Answer for screen readers
The solution for $w$ is $10$.
Steps to Solve
-
Isolate the variable w To solve for $w$, we start from the original equation: $$ 8 = \frac{4}{5}w $$ We need to isolate $w$. To do this, we can multiply both sides of the equation by the reciprocal of $\frac{4}{5}$, which is $\frac{5}{4}$.
-
Multiply both sides by the reciprocal Multiply both sides by $\frac{5}{4}$: $$ 8 \cdot \frac{5}{4} = w $$ This simplifies to: $$ w = \frac{8 \cdot 5}{4} $$
-
Simplify the right side Now, compute the expression on the right: $$ w = \frac{40}{4} = 10 $$
The solution for $w$ is $10$.
More Information
To solve for $w$, we used the concept of isolating the variable by utilizing the reciprocal of the coefficient in front of $w$. This method is widely applicable in algebra for solving linear equations.
Tips
- Forgetting to multiply both sides by the reciprocal of the coefficient.
- Not simplifying the fraction properly after multiplication.
AI-generated content may contain errors. Please verify critical information