Simplify. Express your answer using the given expression: 4/h^0 divided by 4/h^-1.
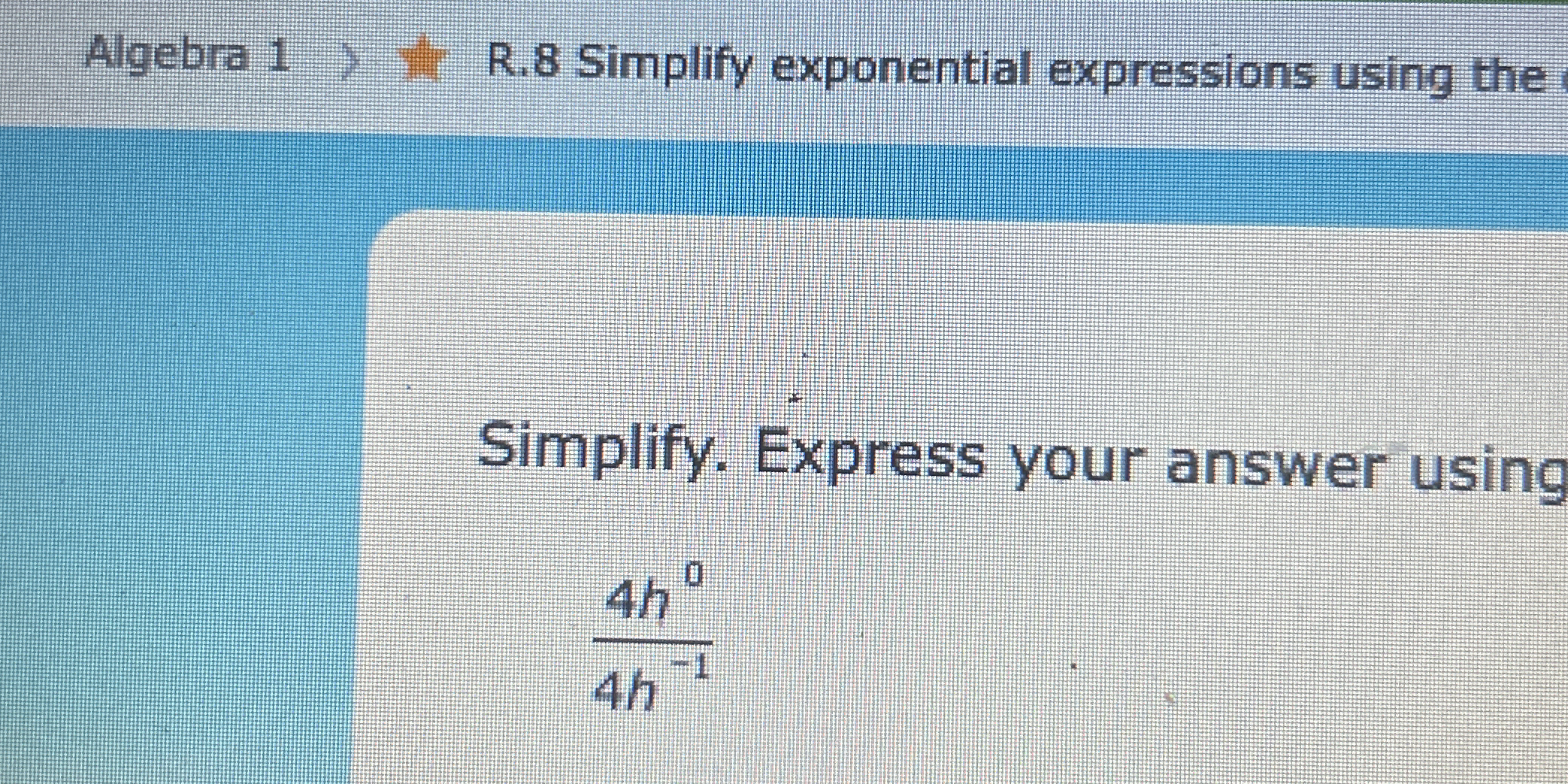
Understand the Problem
The question is asking to simplify the given expression involving exponential terms and to express the answer in a proper format. This requires applying exponent rules and simplification techniques.
Answer
The simplified expression is \( h \).
Answer for screen readers
The simplified expression is ( h ).
Steps to Solve
- Identify the expression to simplify
The expression we need to simplify is:
$$ \frac{4h^0}{4h^{-1}} $$
- Simplify the exponential terms
Recall that any number raised to the power of 0 equals 1. Therefore:
$$ h^0 = 1 $$
This means we can rewrite the expression as:
$$ \frac{4 \cdot 1}{4h^{-1}} = \frac{4}{4h^{-1}} $$
- Simplify the fraction
Next, the 4s in the numerator and denominator can be canceled:
$$ \frac{4}{4h^{-1}} = \frac{1}{h^{-1}} $$
- Apply the negative exponent rule
The negative exponent rule states that $h^{-n} = \frac{1}{h^n}$. Thus we have:
$$ \frac{1}{h^{-1}} = h^1 = h $$
The simplified expression is ( h ).
More Information
In this process, we applied the properties of exponents, specifically that anything raised to the power of zero is one, and that a negative exponent indicates the reciprocal.
Tips
- Forgetting that ( h^0 = 1 ) and continuing to treat ( h^0 ) as an unknown variable.
- Not canceling the common bases and coefficients properly in the fraction.
AI-generated content may contain errors. Please verify critical information