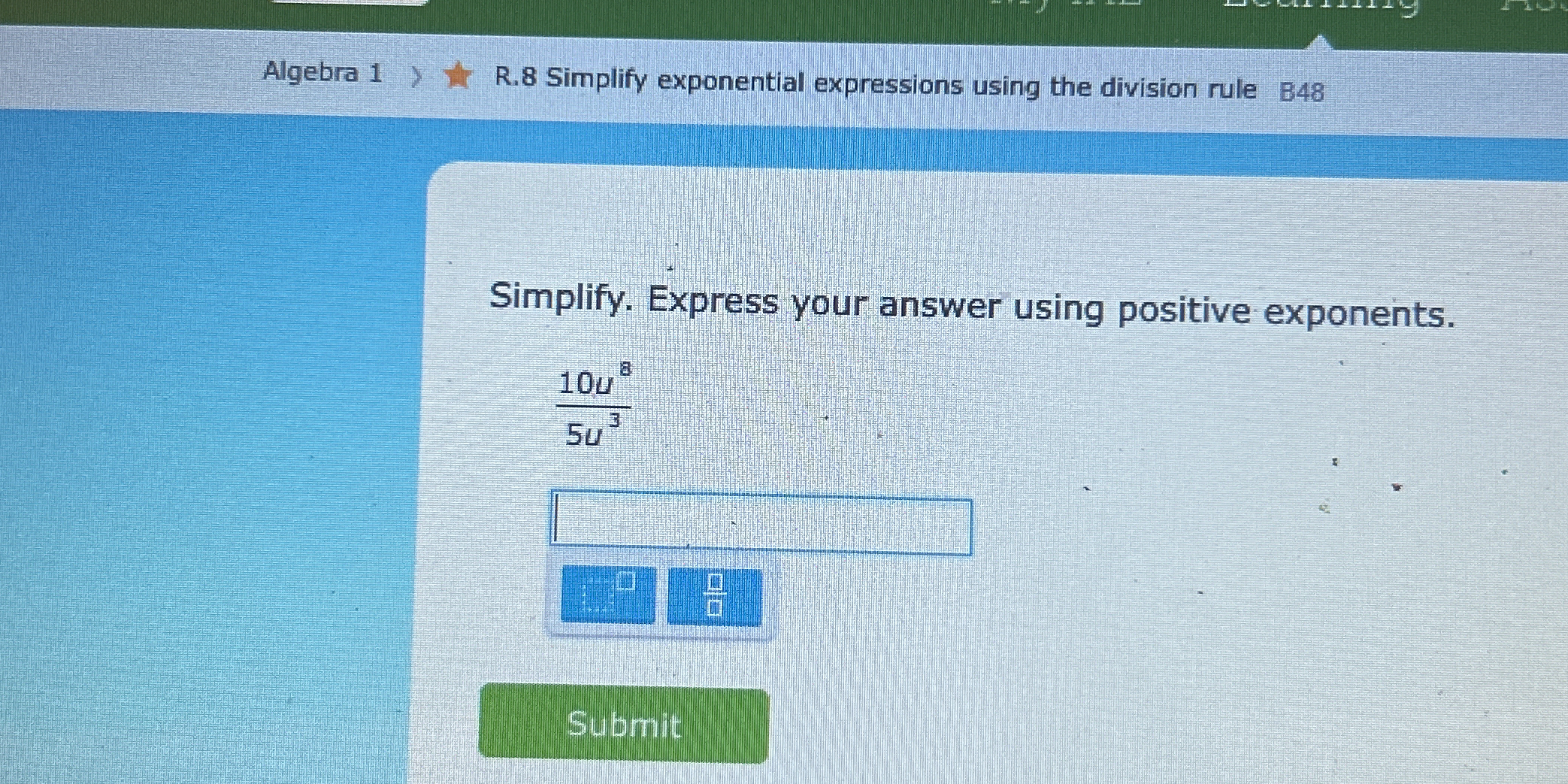
Simplify. Express your answer using positive exponents. 10u^8 / 5u^3
Understand the Problem
The question is asking to simplify the expression \frac{10u^{8}}{5u^{3}} using the division rule of exponents. This involves simplifying both the numerical coefficients and the variable parts of the expression.
Answer
The answer is \( 2u^5 \).
Answer for screen readers
The simplified expression is ( 2u^5 ).
Steps to Solve
- Simplify the Numerical Coefficients
First, divide the numerical coefficients: $$ \frac{10}{5} = 2 $$
- Apply the Division Rule of Exponents
For the variable part, use the rule ( \frac{a^m}{a^n} = a^{m-n} ): $$ \frac{u^8}{u^3} = u^{8-3} = u^5 $$
- Combine the Results
Now, combine the results from the previous steps: $$ \frac{10u^8}{5u^3} = 2u^5 $$
The simplified expression is ( 2u^5 ).
More Information
When simplifying expressions using the division rule of exponents, the key is to handle the numerical coefficients and the variables separately. This method can be applied consistently to many similar problems.
Tips
- Forgetting to simplify the coefficients: Always remember to reduce the numerical part first.
- Incorrectly applying the exponent rule: Ensure to subtract the exponent in the right order.
AI-generated content may contain errors. Please verify critical information