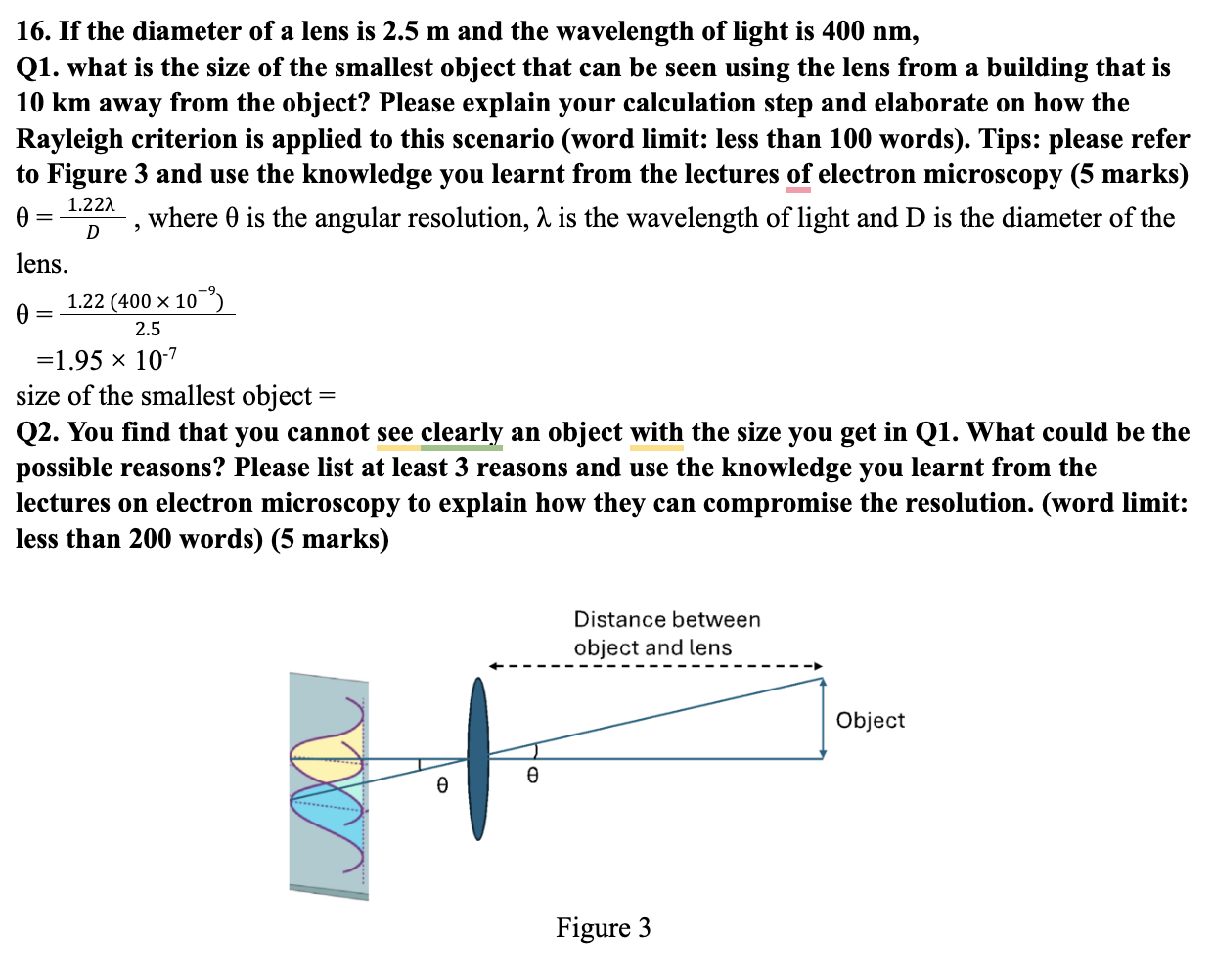
Q1. What is the size of the smallest object that can be seen using the lens from a building that is 10 km away from the object? Please explain your calculation step and elaborate o... Q1. What is the size of the smallest object that can be seen using the lens from a building that is 10 km away from the object? Please explain your calculation step and elaborate on how the Rayleigh criterion is applied to this scenario. Q2. You find that you cannot see clearly an object with the size you get in Q1. What could be the possible reasons? Please list at least 3 reasons and explain how they can compromise the resolution.
Understand the Problem
The question involves calculating the minimum size of an object that can be resolved using a lens, applying the Rayleigh criterion, and discussing potential reasons for not being able to see it clearly despite that size. The user is expected to show calculation steps and provide insights from electron microscopy lectures.
Answer
The size of the smallest object is approximately $1.95 \, \text{mm}$.
Answer for screen readers
The size of the smallest object that can be resolved is approximately $1.95 , \text{mm}$.
Steps to Solve
- Calculate the angular resolution (\theta)
Using the Rayleigh criterion formula:
[ \theta = \frac{1.22 \lambda}{D} ]
where
- (\lambda = 400 \times 10^{-9} , \text{m}) (the wavelength)
- (D = 2.5 , \text{m}) (the diameter of the lens)
Substituting the values, we get:
[ \theta = \frac{1.22 \times (400 \times 10^{-9})}{2.5} = 1.95 \times 10^{-7} , \text{radians} ]
- Calculate the size of the smallest object
Using the formula for size ((s)) being the distance ((L)) to the object times the angular resolution ((\theta)):
[ s = L \cdot \theta ]
Given that the distance (L = 10 , \text{km} = 10,000 , \text{m}),
Substituting the values, we have:
[ s = 10,000 \cdot (1.95 \times 10^{-7}) = 1.95 \times 10^{-3} , \text{m} = 1.95 , \text{mm} ]
- Summary of findings
The size of the smallest object that can be resolved is approximately:
$$ s \approx 1.95 , \text{mm} $$
The size of the smallest object that can be resolved is approximately $1.95 , \text{mm}$.
More Information
The calculation shows that with a lens of 2.5 m diameter and light wavelength of 400 nm, the Rayleigh criterion gives an angular resolution indicating the smallest size that can be distinctly resolved from a distance of 10 km.
Tips
- Failing to convert distance correctly (e.g., mixing kilometers and meters).
- Omitting units when calculating and reporting the final result.
- Not using the correct formula for resolution.
AI-generated content may contain errors. Please verify critical information