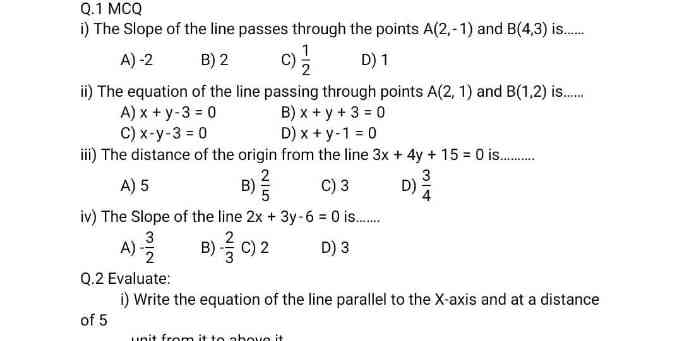
Q.1 MCQ i) The slope of the line passing through points A(2,-1) and B(4,3) is... ii) The equation of the line passing through points A(2,1) and B(1,2) is... iii) The distance from... Q.1 MCQ i) The slope of the line passing through points A(2,-1) and B(4,3) is... ii) The equation of the line passing through points A(2,1) and B(1,2) is... iii) The distance from the origin from the line 3x + 4y + 15 = 0 is... iv) The slope of the line 2x + 3y - 6 = 0 is... Q.2 Evaluate: i) Write the equation of the line parallel to the X-axis and at a distance of 5 units from it above.
Understand the Problem
The question is asking a series of mathematical problems related to lines, slopes, and equations. It includes multiple-choice questions about the slope of a line between two points, the equation of a line given two points, the distance from the origin to a line, and the slope of another linear equation. Additionally, it asks for the equation of a line parallel to the X-axis at a specified distance.
Answer
i) 2, ii) $x + y - 3 = 0$, iii) 3, iv) $-\frac{2}{3}$, Q.2 i) $y = 5$
Answer for screen readers
i) 2
ii) $x + y - 3 = 0$
iii) 3
iv) $-\frac{2}{3}$
Q.2 i) $y = 5$
Steps to Solve
- Calculate the slope between two points
To find the slope $m$ between points A(2, -1) and B(4, 3), use the formula:
$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$
Here, $(x_1, y_1) = (2, -1)$ and $(x_2, y_2) = (4, 3)$, so:
$$ m = \frac{3 - (-1)}{4 - 2} = \frac{3 + 1}{2} = \frac{4}{2} = 2 $$
- Find the equation of the line through two points
For points A(2, 1) and B(1, 2), first calculate the slope $m$:
$$ m = \frac{2 - 1}{1 - 2} = \frac{1}{-1} = -1 $$
Using the point-slope form of a line $y - y_1 = m(x - x_1)$, choose point A(2, 1):
$$ y - 1 = -1(x - 2) $$
This simplifies to:
$$ y - 1 = -x + 2 $$
$$ x + y - 3 = 0 $$ (Option B)
- Calculate the distance from the origin to a line
To find the distance from the origin to the line $3x + 4y + 15 = 0$, use the distance formula:
$$ D = \frac{|Ax_0 + By_0 + C|}{\sqrt{A^2 + B^2}} $$
Here, $A = 3$, $B = 4$, $C = 15$, and $(x_0, y_0) = (0, 0)$:
$$ D = \frac{|3(0) + 4(0) + 15|}{\sqrt{3^2 + 4^2}} = \frac{15}{\sqrt{9 + 16}} = \frac{15}{\sqrt{25}} = \frac{15}{5} = 3 $$
- Determine the slope of a given line
For the line $2x + 3y - 6 = 0$, rearrange into slope-intercept form $y = mx + b$:
$$ 3y = -2x + 6 $$
$$ y = -\frac{2}{3}x + 2 $$
So, the slope is $-\frac{2}{3}$ (Option B).
- Equation of the line parallel to the X-axis
A line parallel to the X-axis has a constant $y$ value. Therefore, for a distance of 5 units above the X-axis, the equation is:
$$ y = 5 $$
i) 2
ii) $x + y - 3 = 0$
iii) 3
iv) $-\frac{2}{3}$
Q.2 i) $y = 5$
More Information
- The slope is defined as the change in $y$ over the change in $x$.
- The equation of a line can be determined using two points and knowing the slope.
- The distance from a point to a line can be calculated using a specific formula involving the line's coefficients.
Tips
- Confusing the coordinates when calculating the slope.
- Not rearranging the line equation into the correct form before identifying the slope.
- Misapplying the distance formula, especially with negative signs.
AI-generated content may contain errors. Please verify critical information