Put the quadratic into vertex form and state the coordinates of the vertex: y = x^2 + 8x - 11.

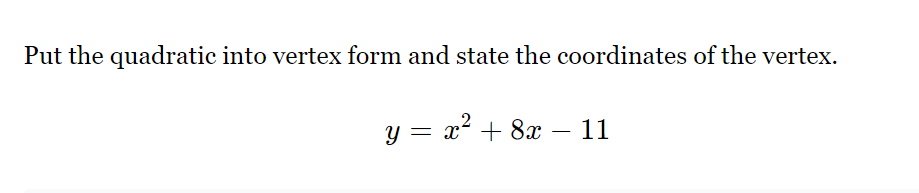
Understand the Problem

The question is asking to convert a given quadratic equation into vertex form and to find the coordinates of the vertex. This involves completing the square and identifying the vertex from the resulting equation.

Answer

The vertex form is $ y = (x + 4)^2 - 27 $ and the vertex coordinates are $ (-4, -27) $.

Steps to Solve

Identify the quadratic expression We start with the quadratic equation ( y = x^2 + 8x - 11 ).
Complete the square To convert to vertex form, we need to complete the square.

Take the coefficient of ( x ), which is ( 8 ), divide it by ( 2 ) and square it: $$ \left(\frac{8}{2}\right)^2 = 16 $$
Rewrite the equation, adding and subtracting ( 16 ): $$ y = (x^2 + 8x + 16 - 16) - 11 $$

Rewrite in vertex form Now combine terms: $$ y = (x + 4)^2 - 27 $$

This is now in vertex form ( y = a(x-h)^2 + k ), where ( (h, k) ) is the vertex.

Identify the vertex From the vertex form ( y = (x + 4)^2 - 27 ), we see that:

( h = -4 ) and ( k = -27 ).

Thus, the coordinates of the vertex are ( (-4, -27) ).

The vertex form of the quadratic is ( y = (x + 4)^2 - 27 ) and the coordinates of the vertex are ( (-4, -27) ).

More Information

The vertex form of a quadratic equation helps easily identify the vertex of the parabola. The vertex can indicate the maximum or minimum point of the quadratic function, depending on the direction the parabola opens.

Tips

Forgetting to change the sign when completing the square can lead to incorrect terms.
Miscalculating the vertex coordinates from the vertex form.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!