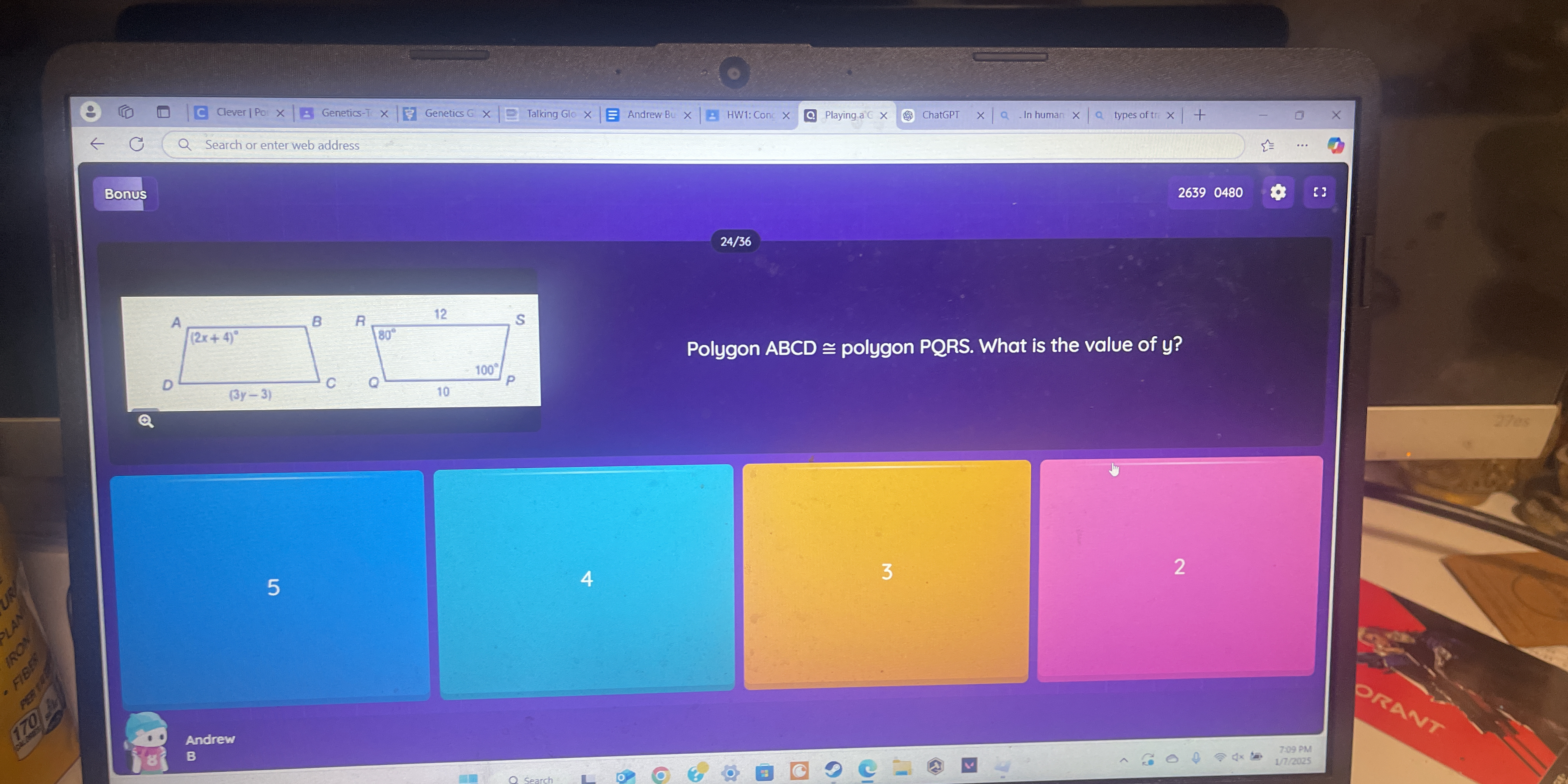
Polygon ABCD ≅ polygon PQRS. What is the value of y?
Understand the Problem
The question is asking for the value of a variable 'y' in the context of two polygons, ABCD and PQRS, that are congruent. It implies using the properties of congruence and the information given about the sides and angles of both polygons to solve for 'y'.
Answer
The value of \( y \) is \( 100 \).
Answer for screen readers
The value of ( y ) is ( 100 ).
Steps to Solve
- Identify congruent sides and angles
Given that polygon ABCD is congruent to polygon PQRS, we can equate corresponding angles and side lengths between the two polygons. For example, angle D (given as $y$) corresponds to angle S (which is $100^\circ$).
- Set up the equation for angle D
From congruence, we know:
[ y = 100 ]
Thus, we can keep $y = 100$ for the first equation.
- Set up the equation for angle A
Next, we can find the value of angle A, which is represented by the expression $(2x + 4)$. We also know that angle S is $80^\circ$:
[ (2x + 4) = 80 ]
- Solve for x
Rearranging the equation gives:
[ 2x + 4 = 80 \implies 2x = 76 \implies x = 38 ]
- Substitute x to find y
Now that we have $x = 38$, substitute it back to find any other necessary values if needed, but in this case, we already have $y = 100$.
The value of ( y ) is ( 100 ).
More Information
In congruent polygons, corresponding angles and sides are equal. This principle allows us to equate angles from one polygon to another and solve for the variables effectively.
Tips
- Confusing the positions of the angles or sides when using congruence. Always ensure you match corresponding angles and sides correctly.
- Forgetting to rearrange equations properly can lead to incorrect solutions. Double-check your algebraic manipulations.
AI-generated content may contain errors. Please verify critical information