lim x → π/2 (1 - sin x) / (cos x)
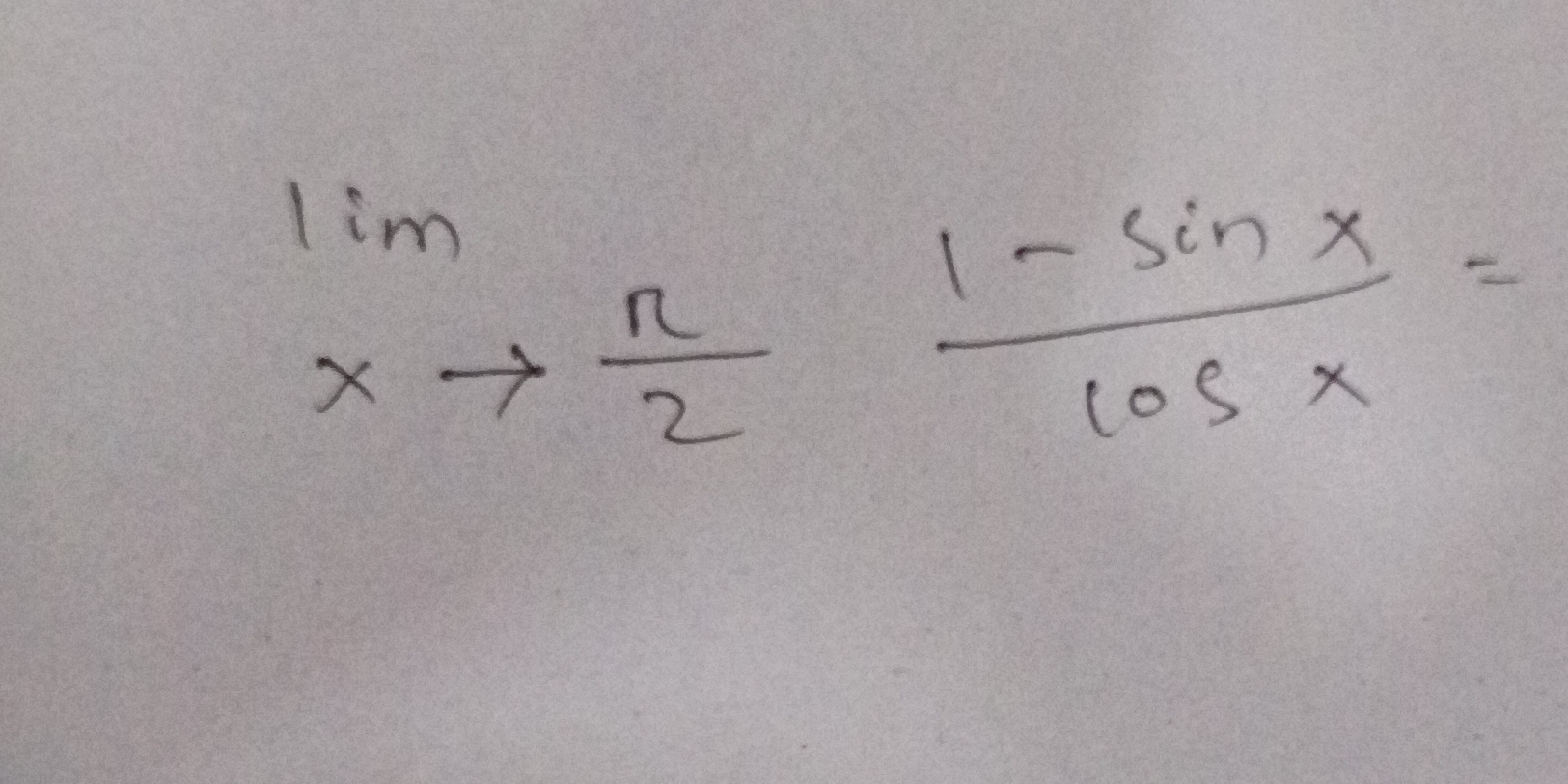
Understand the Problem
The question is asking to evaluate the limit of the expression (1 - sin(x)) / (cos(x)) as x approaches π/2.
Answer
The limit is $0$.
Answer for screen readers
The limit is $0$.
Steps to Solve
- Substituting the limit directly
We begin by substituting $x = \frac{\pi}{2}$ directly into the expression:
$$ \frac{1 - \sin\left(\frac{\pi}{2}\right)}{\cos\left(\frac{\pi}{2}\right)} = \frac{1 - 1}{0} = \frac{0}{0} $$
This results in an indeterminate form ( \frac{0}{0} ).
- Applying L'Hôpital's Rule
Since we have an indeterminate form, we can use L'Hôpital's Rule, which states that if the limit evaluates to ( \frac{0}{0} ) or ( \frac{\infty}{\infty} ), we can take the derivative of the numerator and the derivative of the denominator:
The derivative of the numerator $(1 - \sin(x))$ is:
$$ \frac{d}{dx}(1 - \sin(x)) = -\cos(x) $$
The derivative of the denominator $(\cos(x))$ is:
$$ \frac{d}{dx}(\cos(x)) = -\sin(x) $$
- Re-evaluating the limit
Now we can evaluate the limit again using these derivatives:
$$ \lim_{x \to \frac{\pi}{2}} \frac{-\cos(x)}{-\sin(x)} = \lim_{x \to \frac{\pi}{2}} \frac{\cos(x)}{\sin(x)} = \frac{\cos\left(\frac{\pi}{2}\right)}{\sin\left(\frac{\pi}{2}\right)} = \frac{0}{1} = 0 $$
The limit is $0$.
More Information
This limit demonstrates a fundamental concept in calculus: the evaluation of limits resulting in indeterminate forms and the application of L'Hôpital's Rule to resolve them.
Tips
- Failing to identify the indeterminate form ( \frac{0}{0} ) and then incorrectly substituting the limit.
- Not applying L'Hôpital's Rule correctly or forgetting to differentiate both the numerator and denominator.
AI-generated content may contain errors. Please verify critical information