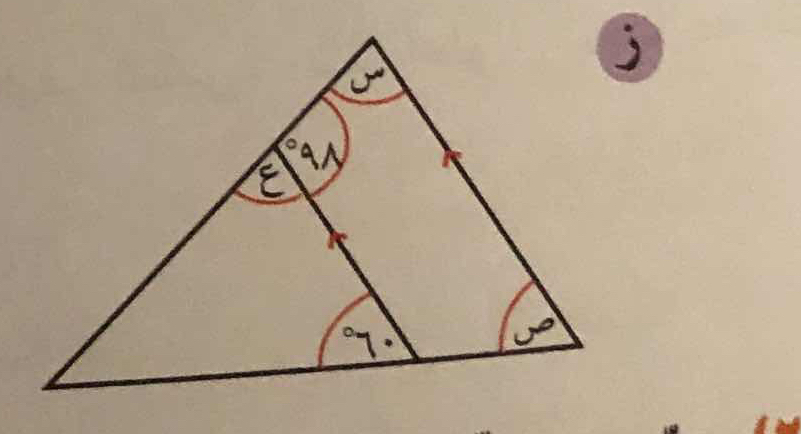
In the triangle, what are the angles represented by E, W, and S if the measures of certain angles are given?
Understand the Problem
The question involves a geometric problem related to angles in a triangle, likely asking for the calculation of one or more angles based on the given information.
Answer
$x + y = 89^\circ$
Answer for screen readers
The sum of angles ( x ) and ( y ) equals ( 89^\circ ), expressed as $x + y = 89^\circ$.
Steps to Solve
- Identify Given Angles
From the triangle diagram, we have the following angles:
-
Angle ( E = 91^\circ )
-
Angle ( F ) (labeled as ( x ))
-
Angle ( G ) (labeled as ( y ))
- Apply Angle Sum Property of a Triangle
The sum of all angles in a triangle is ( 180^\circ ). Therefore, we can write the equation as:
$$ E + F + G = 180^\circ $$
Substituting the known value for ( E ):
$$ 91^\circ + x + y = 180^\circ $$
- Calculate the Sum of Angles
Isolate ( x + y ):
$$ x + y = 180^\circ - 91^\circ $$
This simplifies to:
$$ x + y = 89^\circ $$
- Identify Other Relationships in the Triangle
There are additional angles marked in the diagram that may need to be considered. If any angles ( F ) and ( G ) are connected to other angles outside the triangle, identify those relationships.
- Final Calculations or Derivations
If the problem requires determining specific values for ( x ) or ( y ), any additional equations or relationships can help to derive their values based on the earlier equations.
The sum of angles ( x ) and ( y ) equals ( 89^\circ ), expressed as $x + y = 89^\circ$.
More Information
In a triangle, the interior angles add up to ( 180^\circ ). This property is essential in solving for unknown angles based on known values.
Tips
- Miscalculating the total angle sum by forgetting the fixed ( 180^\circ ) rule.
- Neglecting to analyze additional angle relationships within the triangle.
- Assuming angles outside the triangle do not affect internal calculations.
AI-generated content may contain errors. Please verify critical information