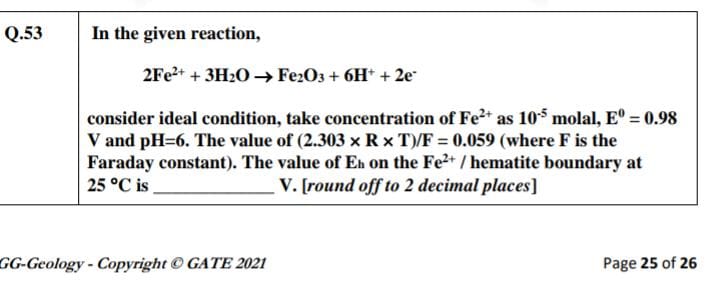
In the given reaction, 2Fe²⁺ + 3H₂O → Fe₂O₃ + 6H⁺ + 2e⁻, consider ideal condition, take concentration of Fe²⁺ as 10⁵ molal, E⁰ = 0.98 V and pH = 6. The value of (2.303 × R × T)/F =... In the given reaction, 2Fe²⁺ + 3H₂O → Fe₂O₃ + 6H⁺ + 2e⁻, consider ideal condition, take concentration of Fe²⁺ as 10⁵ molal, E⁰ = 0.98 V and pH = 6. The value of (2.303 × R × T)/F = 0.059. The value of Eh on the Fe²⁺/hematite boundary at 25 °C is __________ V. [round off to 2 decimal places] Read more

Steps to Solve

1. Identify the Nernst Equation

The Nernst equation relates the electrode potential (Eh) to the standard potential (E°) and the concentrations of the reactants and products: $$ E_h = E° - \frac{(RT)}{nF} \ln Q $$ where:

• ( n ) = number of electrons transferred (in this case, 2),
• ( Q ) = reaction quotient.

2. Determine the Reaction Quotient (Q)

For the reaction, the reaction quotient ( Q ) is given by the formula: $$ Q = \frac{[\text{H}^+]^6}{[\text{Fe}^{2+}]^2} $$ Given ( \text{pH} = 6 ), we can calculate ( [\text{H}^+] ): $$ [\text{H}^+] = 10^{-6} , \text{molal} $$ Now substitute into the ( Q ) equation: $$ Q = \frac{(10^{-6})^6}{(10^{-5})^2} = \frac{10^{-36}}{10^{-10}} = 10^{-26} $$

3. Substitute Values into the Nernst Equation

Now, substitute ( E° = 0.98 , V ), ( R = 8.314 , \text{J/(mol K)} ), ( T = 298 , \text{K} ), ( n = 2 ), ( F = 96485 , \text{C/mol} ), and ( Q = 10^{-26} ): $$ E_h = 0.98 - 0.059 \cdot \frac{1}{2} \ln(10^{-26}) $$

4. Calculate the Natural Logarithm

Calculate ( \ln(10^{-26}) ): $$ \ln(10^{-26}) = -26 \cdot \ln(10) \approx -26 \cdot 2.303 = -59.878 $$

5. Complete the Calculation

Insert this value back into the Nernst equation: $$ E_h = 0.98 - 0.059 \cdot (-29.939) $$ Calculating this gives: $$ E_h = 0.98 + 1.763 = 2.743 , V $$ Rounding off to two decimal places, $$ E_h \approx 2.74 , V $$