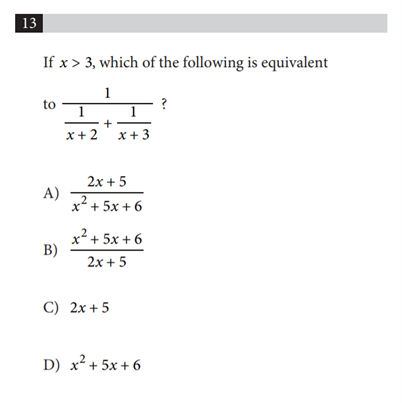
If x > 3, which of the following is equivalent to 1/(x + 2) + 1/(x + 3)?
Understand the Problem
The question is asking to simplify the expression given, which involves adding fractions. We need to find a common denominator and combine the two fractions to determine which of the provided options represents the equivalent expression.
Answer
The answer is $\frac{2x + 5}{x^2 + 5x + 6}$.
Answer for screen readers
The equivalent expression is $\frac{2x + 5}{x^2 + 5x + 6}$.
Steps to Solve
-
Find the common denominator To add the fractions $\frac{1}{x+2}$ and $\frac{1}{x+3}$, we need a common denominator. The common denominator is the product of the two denominators: $$(x + 2)(x + 3)$$
-
Rewrite the fractions Next, rewrite each fraction with the common denominator. [ \frac{1}{x + 2} = \frac{x + 3}{(x + 2)(x + 3)} \quad \text{and} \quad \frac{1}{x + 3} = \frac{x + 2}{(x + 2)(x + 3)} ]
-
Combine the fractions Now, combine the fractions: [ \frac{x + 3}{(x + 2)(x + 3)} + \frac{x + 2}{(x + 2)(x + 3)} = \frac{(x + 3) + (x + 2)}{(x + 2)(x + 3)} ] This simplifies to: [ \frac{2x + 5}{(x + 2)(x + 3)} ]
-
Identify the equivalent expression Now we look for an answer choice that matches: $$ \frac{2x + 5}{(x + 2)(x + 3)} $$
The equivalent expression is $\frac{2x + 5}{x^2 + 5x + 6}$.
More Information
The expression $\frac{1}{x+2} + \frac{1}{x+3}$ combines two fractions with differing denominators. Finding a common denominator is crucial in simplifying fractions. The resultant expression can also be factored into a quadratic form which appears in the answer choices.
Tips
- Forgetting to find the common denominator first can lead to incorrect simplification.
- Failing to properly combine the numerators may result in an incorrect final fraction.
- Not simplifying the final answer, which can lead to confusion with the provided options.
AI-generated content may contain errors. Please verify critical information