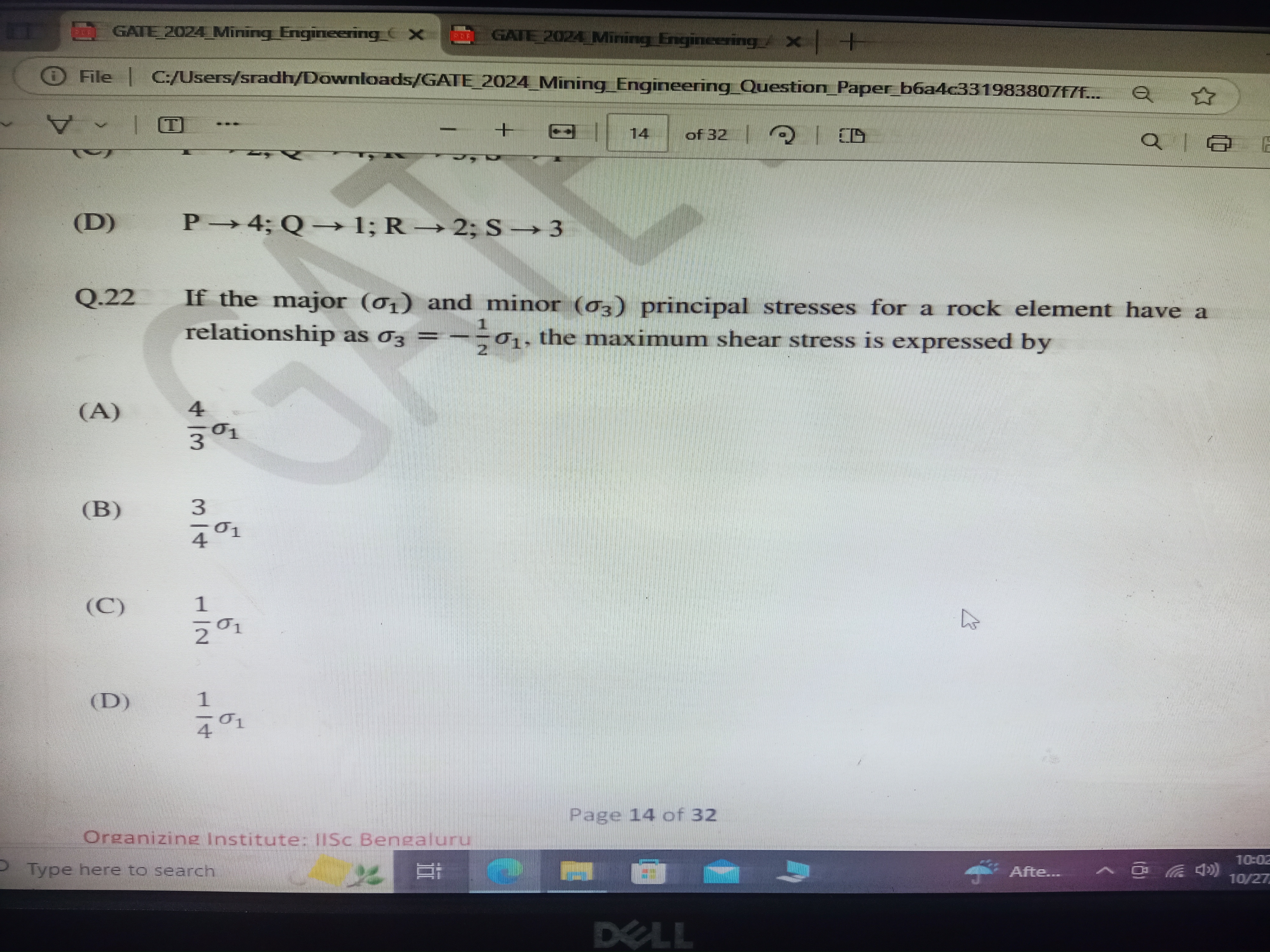
If the major (σ1) and minor (σ3) principal stresses for a rock element have a relationship as σ3 = −1/2 σ1, the maximum shear stress is expressed by
Understand the Problem
The question is asking about the relationship between principal stresses in a rock element and how that relates to the expression for maximum shear stress when given a specific equation for the minor principal stress.
Answer
The maximum shear stress is $\tau_{max} = \frac{3}{4} \sigma_1$.
Answer for screen readers
The maximum shear stress is expressed by: $$ \tau_{max} = \frac{3}{4} \sigma_1 $$
Steps to Solve
- Identify Principal Stresses Identify the notation for principal stresses. In this case, we have:
- Major principal stress: $\sigma_1$
- Minor principal stress: $\sigma_3$
Given the relationship: $$ \sigma_3 = -\frac{1}{2} \sigma_1 $$
-
Calculate Maximum Shear Stress The maximum shear stress, $\tau_{max}$, can be expressed using the principal stresses with the formula: $$ \tau_{max} = \frac{\sigma_1 - \sigma_3}{2} $$
-
Substitute Minor Principal Stress Substitute the expression for $\sigma_3$ into the maximum shear stress formula: $$ \tau_{max} = \frac{\sigma_1 - \left(-\frac{1}{2} \sigma_1\right)}{2} $$
-
Simplify the Expression Simplify the equation: $$ \tau_{max} = \frac{\sigma_1 + \frac{1}{2} \sigma_1}{2} = \frac{\frac{3}{2} \sigma_1}{2} = \frac{3}{4} \sigma_1 $$
The maximum shear stress is expressed by: $$ \tau_{max} = \frac{3}{4} \sigma_1 $$
More Information
In engineering and material science, maximum shear stress is a critical concept for understanding failure under load. The derived relationship helps in assessment and design of structures against yielding.
Tips
- Forgetting to apply the negative sign when using the minor principal stress in calculations.
- Misapplying the formula for maximum shear stress by not correctly identifying the principal stresses involved.
AI-generated content may contain errors. Please verify critical information