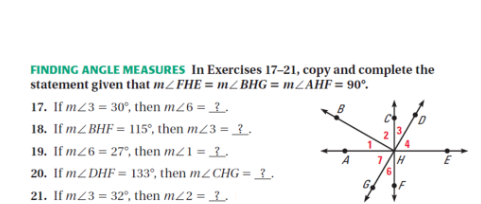
If m∠3 = 30°, then m∠6 = _. If m∠BHF = 115°, then m∠3 = _. If m∠6 = 27°, then m∠1 = _. If m∠DHF = 133°, then m∠CHG = _. If m∠3 = 32°, then m∠2 = _.
Understand the Problem
The question involves finding and completing statements related to angles, based on their measurements and relationships described in the image. Specifically, it requires determining the values of certain angles when given others, applying knowledge of angle relationships such as supplementary and complementary angles.
Answer
17. $m∠6 = 60°$ 18. $m∠3 = 65°$ 19. $m∠1 = 63°$ 20. $m∠CHG = 47°$ 21. $m∠2 = 58°$
Answer for screen readers
-
If $m∠3 = 30°$, then $m∠6 = 60°$.
-
If $m∠BHF = 115°$, then $m∠3 = 65°$.
-
If $m∠6 = 27°$, then $m∠1 = 63°$.
-
If $m∠DHF = 133°$, then $m∠CHG = 47°$.
-
If $m∠3 = 32°$, then $m∠2 = 58°$.
Steps to Solve
- Identify Given Angles and Relationships
Given that $m∠FHE = m∠BHG = m∠AHF = 90°$, we know that these angles are right angles. This means they measure 90 degrees each.
- Determine Relationships
For each exercise, we will apply the fact that angles on a straight line sum up to $180°$ and that angles that form a right angle together add up to $90°$.
- Solve Each Statement
For each exercise, we will find the missing angle using the relationships:
- Exercise 17: If $m∠3 = 30°$, then $m∠6 = 90° - 30° = 60°$.
- Exercise 18: If $m∠BHF = 115°$, then $m∠3 = 180° - 115° = 65°$.
- Exercise 19: If $m∠6 = 27°$, then $m∠1 = 90° - 27° = 63°$.
- Exercise 20: If $m∠DHF = 133°$, then $m∠CHG = 180° - 133° = 47°$.
- Exercise 21: If $m∠3 = 32°$, then $m∠2 = 90° - 32° = 58°$.
-
If $m∠3 = 30°$, then $m∠6 = 60°$.
-
If $m∠BHF = 115°$, then $m∠3 = 65°$.
-
If $m∠6 = 27°$, then $m∠1 = 63°$.
-
If $m∠DHF = 133°$, then $m∠CHG = 47°$.
-
If $m∠3 = 32°$, then $m∠2 = 58°$.
More Information
Each angle relationship is based on the fundamental properties of angles, where the sum of angles on a straight line equals $180°$ and the complementary angles add up to $90°$. This reinforces the concepts of supplementary and complementary angles in geometry.
Tips
- Confusing Complementary and Supplementary Angles: It's important to remember that complementary angles sum up to $90°$, while supplementary angles sum to $180°$.
- Incorrect Calculations: Double-check arithmetic calculations when subtracting angle measures to avoid mistakes.
- Misidentifying Angles: Ensure that the correct angles are being referenced in the relationships given.
AI-generated content may contain errors. Please verify critical information