If m∠1 = (18x - 1)° and m∠2 = (23x + 17)°, find m∠2.
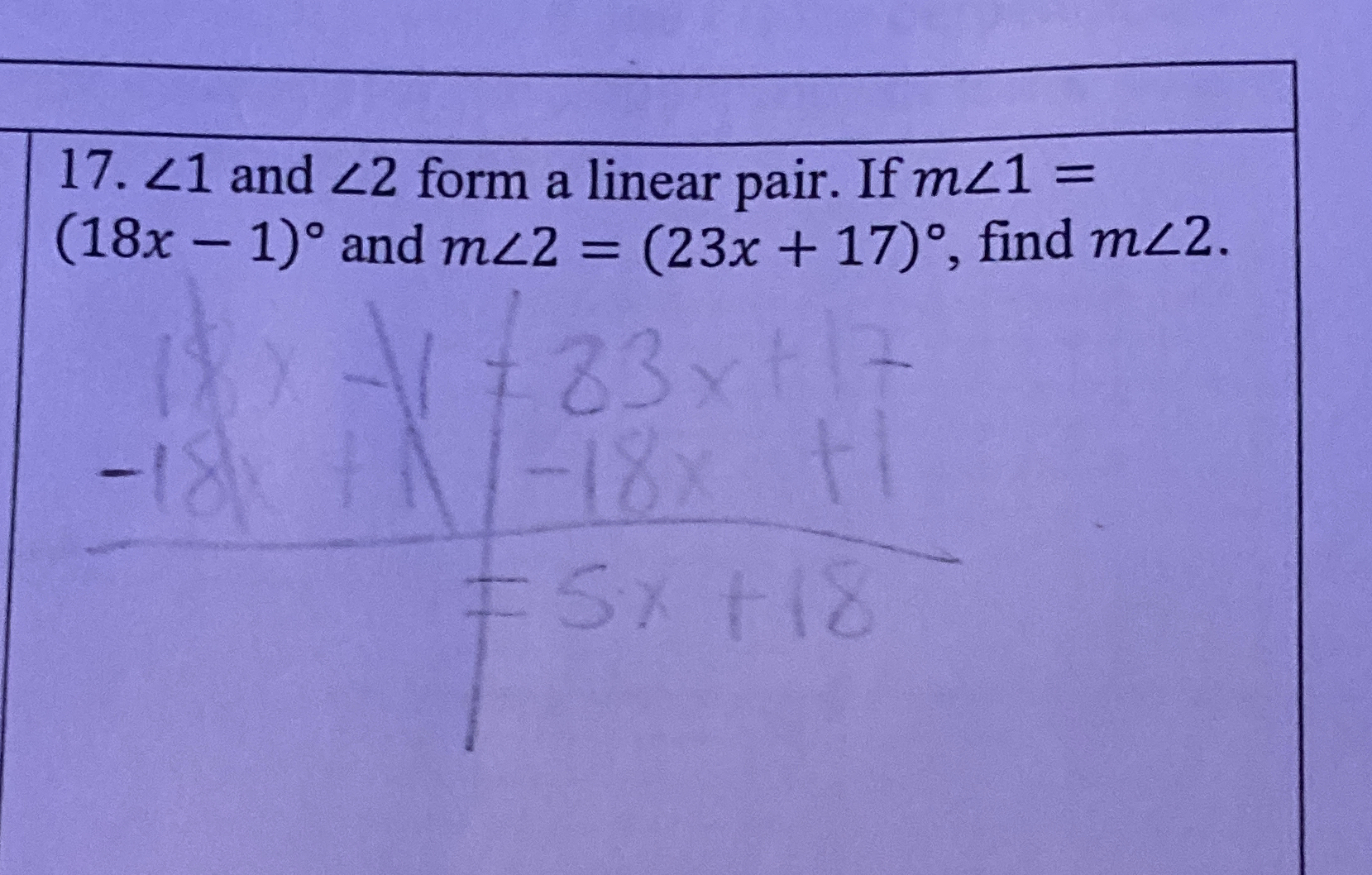
Understand the Problem
The question asks to find the measure of angle 2 given the expressions for the measures of two angles that form a linear pair. We will set up an equation based on the fact that the sum of the angles in a linear pair is 180 degrees.
Answer
The measure of angle 2 is $109^\circ$.
Answer for screen readers
The measure of angle 2 is $109^\circ$.
Steps to Solve
-
Set Up the Equation Since angles 1 and 2 form a linear pair, their measures add up to 180 degrees. Therefore, we can set up the equation: $$ (18x - 1) + (23x + 17) = 180 $$
-
Combine Like Terms Next, combine the terms on the left side of the equation: $$ 18x + 23x - 1 + 17 = 180 $$
This simplifies to: $$ 41x + 16 = 180 $$
- Isolate the Variable Subtract 16 from both sides of the equation to isolate the variable term: $$ 41x = 180 - 16 $$
This simplifies to: $$ 41x = 164 $$
- Solve for x Now, divide both sides by 41 to solve for x: $$ x = \frac{164}{41} $$
Calculating this gives: $$ x = 4 $$
- Substitute Back to Find m∠2 Now substitute ( x = 4 ) back into the expression for ( m∠2 ): $$ m∠2 = 23(4) + 17 $$
This simplifies to: $$ m∠2 = 92 + 17 = 109 $$
The measure of angle 2 is $109^\circ$.
More Information
The calculation shows how the angles relate since they form a linear pair, which is critical in understanding angle relationships in geometry.
Tips
- Neglecting to account for both angles when setting up the equation.
- Mistakes in combining like terms can lead to incorrect values for ( x ).
- Forgetting to substitute back correctly into the angle expression.
AI-generated content may contain errors. Please verify critical information