Understand the Problem
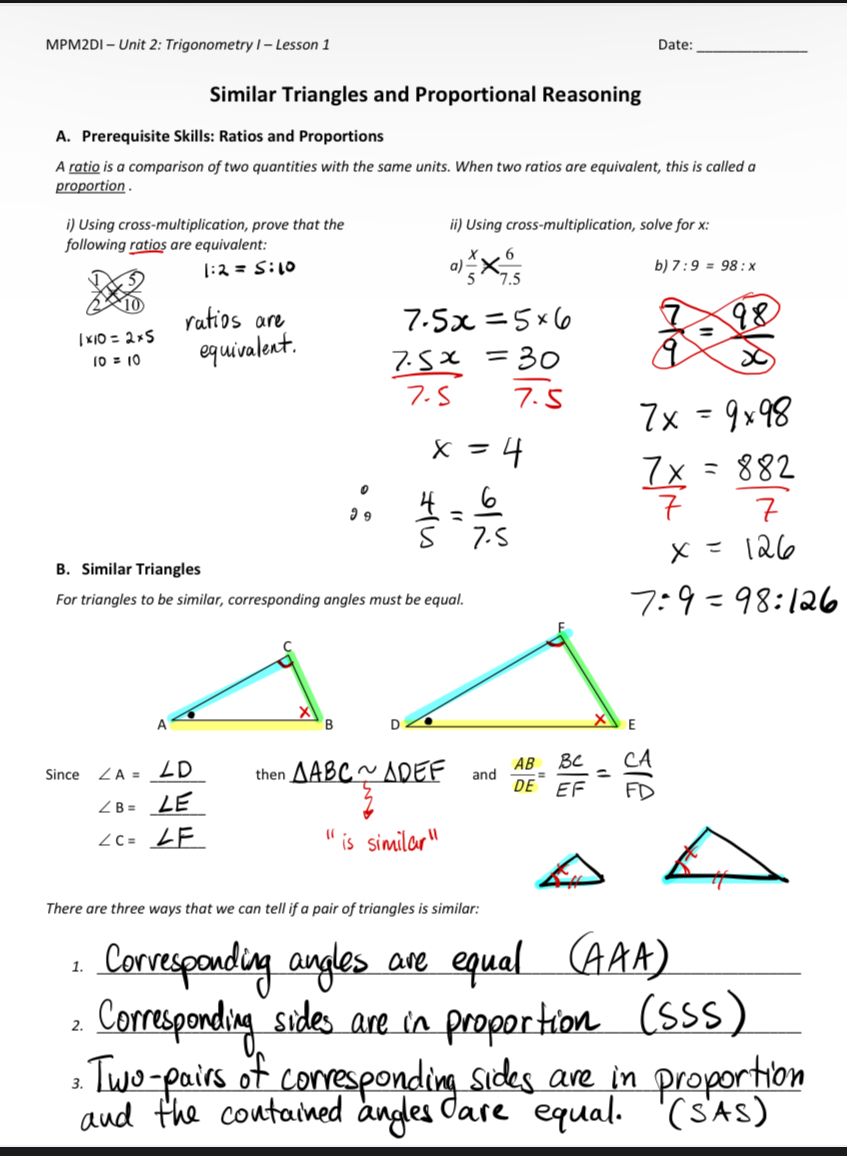
The image provides information on ratios, proportions, and the conditions for triangles to be similar. It includes examples of using cross multiplication and the definitions of corresponding angles and sides in similar triangles.
Answer
The value of \(x\) is \(126\).
Answer for screen readers
The value of (x) is (126).
Steps to Solve
-
Set Up the Proportion Identify the two ratios given in the problem. For example, from the question, the ratio is given as (7:9) and we need to find the value of (x) in the proportion (7:9 = 98:x).
-
Cross-Multiply Using cross multiplication, multiply the means and the extremes. This means: $$ 7 \cdot x = 9 \cdot 98 $$
-
Calculate the Right Side Now, calculate (9 \cdot 98): $$ 9 \cdot 98 = 882 $$
-
Rewrite the Equation Substituting back into the equation gives: $$ 7x = 882 $$
-
Solve for (x) To get (x) by itself, divide both sides by 7: $$ x = \frac{882}{7} $$
-
Perform the Division Calculate the division: $$ x = 126 $$
The value of (x) is (126).
More Information
This value is derived from using the properties of proportions and applying the cross-multiplication method. Proportions are commonly used in solving problems related to similar triangles.
Tips
- Forgetting to cross-multiply correctly, leading to incorrect equations.
- Not simplifying fractions or calculations properly, which can lead to an incorrect value for (x).
AI-generated content may contain errors. Please verify critical information