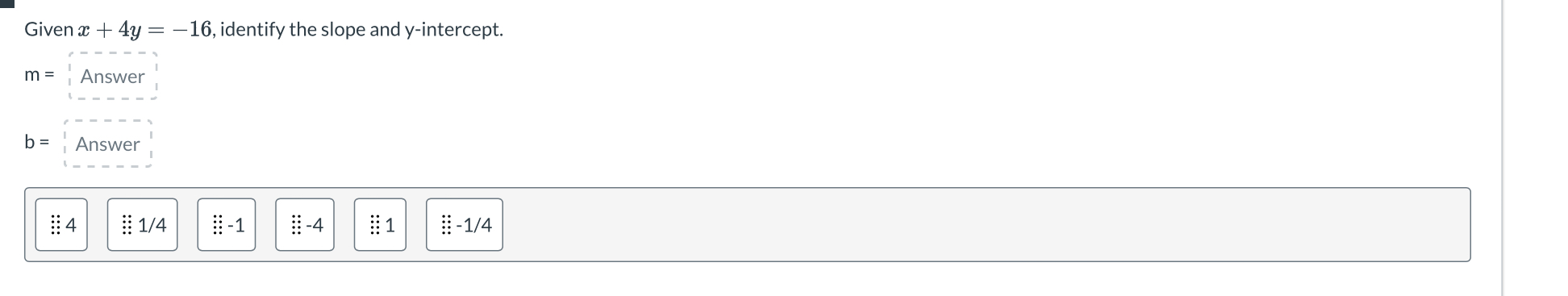
Given x + 4y = -16, identify the slope and y-intercept.
Understand the Problem
The question is asking to identify the slope (m) and y-intercept (b) of the given equation. To solve this, we need to rearrange the equation into slope-intercept form (y = mx + b) and then extract the values of m and b.
Answer
m = $-\frac{1}{4}$, b = $-4$
Answer for screen readers
m = $-\frac{1}{4}$
b = $-4$
Steps to Solve
-
Rearrange the equation To find the slope and y-intercept, we first need to rearrange the given equation $x + 4y = -16$ into the slope-intercept form, which is $y = mx + b$.
-
Isolate y Subtract $x$ from both sides of the equation: $$ 4y = -x - 16 $$
-
Divide by the coefficient of y Next, divide every term by 4 to get $y$ by itself: $$ y = -\frac{1}{4}x - 4 $$
-
Identify m and b Now the equation is in the form $y = mx + b$. Here, $m = -\frac{1}{4}$ and $b = -4$.
m = $-\frac{1}{4}$
b = $-4$
More Information
The slope $m$ indicates that for every 1 unit increase in $x$, $y$ decreases by $\frac{1}{4}$ units. The y-intercept $b$ tells us that the line crosses the y-axis at $-4$.
Tips
- Not isolating y correctly: Some might forget to perform the same operations on both sides of the equation, leading to incorrect expressions for y.
- Misidentifying m and b: Ensure you clearly identify the slope and intercept after getting the equation into the correct form.
AI-generated content may contain errors. Please verify critical information